|
|
A Source Book in Mathematics, 1200-1800
Contents:
Show Summary
Hide Summary
Historical SummaryIsaac Newton started to work on what is now called the calculus in 1664 under Barrow at Cambridge (Selection IV.14). One of his early sources was the Latin edition by F. van Schooten of the Géométrie of Descartes, which also had contributions to the infinitesimal calculus. Newton’s first manuscript notes date from 1665. Here we see emerge his "pricked" letters, such as x for our dx/dt. Studying Wallis’s Arithmetica infinitorum he also discovered the binomial series. Then, in 1669, having studied Nicolas Mercator’s Logarithmotechnia (London, 1668) and James Gregory’s De vera circuli et hyperbolae quadrotura (Padua, 1667), he composed the manuscript later published as De analysi per aequationes numero terminorum infinitas (ed. W. Jones, London, 1711). Expanding on his fluxional methods, he wrote another text in 1671, entitled Methodus fluxionum et serierum infinitorum, first published, in English translation, as The method of fluxions and infinite series, ed. John Colson (London, 1736); the original was first published by Samuel Horsley in the Opera omnia (London, 1779–1785), under the title Geometria analytica.Then, in 1676, in two letters to Henry Oldenburg, the secretary of the Royal Society and, like Mersenne at an earlier date, a man whose scientific contacts connected him with practicallyall who worked in the exact sciences, Newton presented some of his results, especially on the binomial series and on fluxions. The letters were destined for Leibniz, then in his early struggles for the discovery of his own calculus (see Selection V.1). After some time, Newton’s attention was directed toward mechanics and astronomy; the result was the immortal Philosophiae naturalis principia mathematica (London, 1687; Principia for short), with its exposition of the planetary theory on the basis of the law of universal gravity. Newton did not explain his theory of fluxions in this book, preferring to give his proofs in classical geometric form as Huygens had done. However, some of his lemmas and propositions present, in carefully chosen language, a few products of his meditations on the calculus, and we reprint them here as Selections V.5, 6. Then, finally, in an attempt to collect his thoughts on fluxions, Newton produced in 1693 a manuscript that was eventually published as Tractatus de quadratura curvarum (London, 1704), which we have chosen for Selection V.7, being, as it seems, part of the last formulation that Newton gave to his theory of fluxions.The Analysis per aequationes, the Quadratura curvarum, and the Methodus fluxionum have been republished, in their eighteenth-century English translations, by D. T. Whiteside, The mathematical works of Isaac Newton (Johnson Reprint Co., New York, London, 1964).Our first selection of Newton’s work gives essential parts of his two letters of 1676 to Oldenburg, dealing in the main with the binomial series. By applying Wallis’s methods of interpolation and extrapolation to new problems, Newton had taken the concept of negative and fractional exponents from Wallis, and so had been able to generalize the binomial theorem, already known for a long time for positive integral exponents (see Selection I.5 on the Pascal triangle), to these more generalized exponents, by which a polynomial expression was changed into an infinite series. He then was able to show how a great many series that already existed in the literature could be regarded as special cases, either directly or by differentiation or integration.Here follow the two letters from Newton to Oldenburg; they are taken from The correspondence of Isaac Newton, ed. H. W. Turnbull (Cambridge University Press, New York, 1959), vol. 1.Other examples give the solution of the equations and series for sin x and for sin2 x, the solution of Kepler’s problem (to divide a semicircle by a line through a given point on the diameter into two sections of which the areas are in given proportion) for an ellipse, the rectification of the are of an ellipse and a hyperbola, the area of a hyperbola with the aid of the series for the logarithm, the quadrature of the quadratrix tan x/a, and the volume of a segment of an ellipsoid of rotation. Newton, careful not to give too much away, selected these examples from results that were already known.Leibniz answered in his letter of August 17 with an account of several of his own results in finding quadratures, hinting at his possession of a general method. He also offered several series, among them as the ratio of the area of a circle to the circumscribed square—a series which Leibniz had already mentioned to friends in 1673, but which James Gregory had found before.Newton was interested, and answered as follows:Newton continues by mentioning in a guarded way, by means of an anagram, that he has a general method for finding tangents and quadratures, which is not limited by irrationalities He gives a series representation of the binomial integralbut without explanation (which Leibniz had no trouble in finding, as a marginal note to the letter shows). Among examples Newton gives the rectification of the cissoid a formula in series which we can writeand the solution of x fromin the formA few years after Newton’s discovery of the binomial series James Gregory, the Scottish mathematician, rediscovered it independently. We know it from letters of Gregory to the London mathematician John Collins (1625–1683), a correspondent of Newton’s and of many other mathematicians. We give here a translation from Gregory’s letter of November 20, 1670, taken from James Gregory tercentenary memorial volume, ed. H. W. Turnbull (Bell, London, 1939), 131–133.This statement gives for which we recognize as the binomial expansion of whose logarithm isBy pure equation Gregory means an equation of the form rational. He adds an example which gives the daily rate percent at Compound interest equivalent to 6 percent per annum; he takesThenthe correct value is 100.0159919.On the atmosphere of intellectual tension typical of the period, see for example the accounts in J. E. Hofmann, Die Entwicklungsgeschichte der Leibnizschen Mathematik (Leibnizens Verlag, Munich, 1949), 60–87, 194–205, or J. F. Scott, The mathematical work of John Wallis (Taylor and Francis, London, 1938), chaps. 9, 10. See further H. W. Turnbull, The mathematical discoveries of Newton (Blackie and Son, Glasgow, 1945).
Mathematics
4 NEWTON AND GREGORY.
Binomial Series
LETTER OF JUNE 13, 1676
Most worthy Sir,
Though the modesty of Mr. Leibniz, in the extracts from his letter which you have lately sent me, pays great tribute to our countrymen for a certain theory of infinite series, about which there now begins to be some talk, yet I have no doubt that he has discovered not only a method for reducing any quantities whatever to such series, as he asserts, but also various shortened forms, perhaps like our own, if not even better. Since, however, he very much wants to know what has been discovered in this subject by the English, and since I myself fell upon this theory some years ago, I have sent you some of those things which occurred to me in order to satisfy his wishes, at any rate in part.
Fractions are reduced to infinite series by division; and radical quantities by extraction of the roots, by carrying out those operations in the symbols just as they are commonly carried out in decinaal numbers. These are the foundations of these reductions: but extractions of roots are much shortened by this theorem,
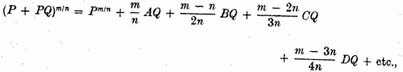
where
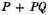
signifies the quantity whose root or even any power, or the root of a power, is to be found; P signifies the first term of that quantity, Q the remaining terms divided by the first, and m/n the numerical index of the power of
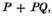
whether that power is integral or (so to speak) fractional, whether positive or negative. For as analysts, instead of aa, aaa, etc., are accustomed to write a2, a3, etc., so instead of

etc. I write
and instead of
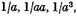
I write
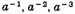
. 1 And so for
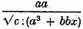
I write
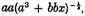
and for
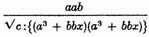
I write
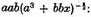
in which last case, if
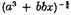
is supposed to be
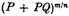
in the Rule, then P will be equal to a3, Q to bbx/a3, m to –2, and n to 3. Finally, for the terms found in the quotient in the course of the working I employ A, B, C, D, etc., namely, A for the first term, Pm/n; B for the second term, ( m/n) AQ; and so on. For the rest, the use of the rule will appear from the examples.
Example 1.
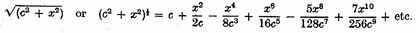
For in this ease,
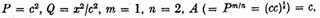
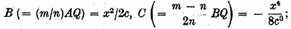
and so on.
LETTER OF OCTOBER 24, 1676
Cambridge October 24 1676
Most worthy Sir,
I can hardly ten with what pleasure I have read the letters of those very distinguished men Leibniz and Tschirnhaus.4 Leibniz’s method for obtaining convergent series is certainly very elegant, and it would have sufficiently revealed the genius of its author, even if he had written nothing else. But what he has scattered elsewhere throughout his letter is most worthy of his reputation—it leads us also to hope for very great things from him. The variety of ways by which the same goal is approached has given me the greater pleasure, because three methods of arriving at series of that kind had already become known to me, so that I could scarcely expect a new one to be communicated to us. One of mine I have described before; I now add another, namely, that by which I first chanced on these series—for I chanced on them before I knew the divisions and extractions of roots which I now use. And an explanation of this will serve to lay bare, what Leibniz desires from me, the basis of the theorem set forth near the beginning of the former letter.
At the beginning of my mathematical studies, when I had met with the works of our celebrated Wallis, on considering the series by the intercalation of which he himself exhibits the area of the circle and the hyperbola, the fact that, in the series of curves whose common base or axis is x and the ordinates
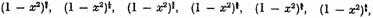
etc.,
if the areas of every other of them, namely
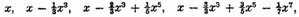
etc.
could be interpolated, we should have the areas of the intermediate ones, of which the first
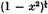
is the circle: in order to interpolate these series I noted that in all of them the first term was x and that the second terms
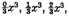
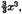
etc., were in arithmetical progression, and hence that the first two terms of the series to be intercalated ought to be
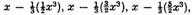
etc. To intercalate the rest I began to reflect that the denominators 1, 3, 5, 7, etc. were in arithmetical progression, so that the numerical coefficients of the numerators only were still in need of investigation. But in the alternately given areas these were the figures of powers of the number 11, namely of these, 11 0, 11 1, 11 2, 11 3, 11 4, that is, first 1; then 1, 1; thirdly, 1, 2, 1; fourthly 1, 3, 3, 1; fifthly 1, 4, 6, 4, 1, etc. And so I began to inquire how the remaining figures in these series could be derived from the first two given figures, and I found that on putting m for the second figure, the rest would be produced by continual multiplication of the terms of this series,
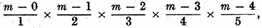
etc.
For example, let

and
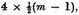
that is 6 will be the third term, and
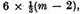
that is 4 the fourth, and

that is 1 the fifth, and
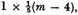
that is 0 the sixth, at which term in this case the series stops. Accordingly, I applied this rule for interposing series among series, and since, for the circle, the second term was
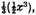
I put
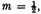
and the terms arising were
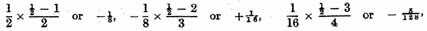
and so to infinity. Whence I came to understand that the area of the circular segment which I wanted was
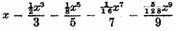
etc.
And by the same reasoning the areas of the remaining curves, which were to be inserted, were likewise obtained: as also the area of the hyperbola and of the other alternate curves in this series

etc. And the same theory serves to intercalate other series, and that through intervals of two or more terms when they are absent at the same time. This was my first entry upon these studies, and it had certainly escaped my memory, had I not a few weeks ago east my eye back on some notes.
But when I had learnt this, I immediately began to consider that the terms
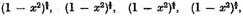
etc.,
that is to say,
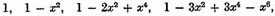
etc.
could be interpolated in the same way as the areas generated by them: and that nothing else was required for this purpose hut to omit the denominators 1, 3, 5, 7, etc., which are in the terms expressing the areas; this means that the coefficients of the terms of the quantity to be intercalated
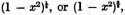
or in general
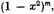
arise by the continued multiplication of the terms of this series
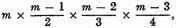
etc.,
so that (for example)
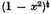
was the value of
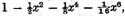
etc.,

etc.,
and
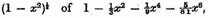
etc.
So then the general reduction of radicals into infinite series by that rule, which I laid down at the beginning of my earlier letter, became known to me, and that before I was acquainted with the extraction of roots. But once this was known, that other could not long remain hidden from me. For in order to test these processes, I multiplied
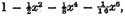
etc.
into itself; and it became
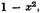
the remaining terms vanishing by the continuation of the series to infinity. And even so
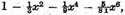
etc. multiplied twice into itself also produced
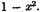
And as this was not only sure proof of these conclusions so too it guided me to try whether, conversely, these series, which it thus affirmed to be roots of the quantity
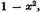
might not be extracted out of it in an arithmetical manner. And the matter turned out well. This was the form of the working in square roots.
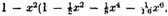
etc.
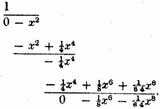
TO FIND THE NUMBER OF A LOGARITHM
Given b, log
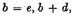
log
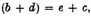
it is required to find the number whose logarithm is
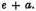
Take a series of continual proportions b, d, d2/b, d3/b2, etc., and another series
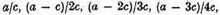
etc., and let f/c be the product of the first two terms of the second series, g/c that of the first three, h/c that of the first four, i/c that of the first five, etc. The number whose logarithm is
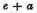
will be
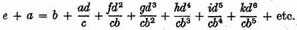
Hence with a little work but without difficulty any pure equation whatever may be solved.
1 Newton had learned this method of broken and negative exponents from Wallis, but the idea goes back as far as Oresme and Chuquet; see Selection II.2. Through the influence of Wallis and Newton the method was gradually adopted by other mathematicians. The otation
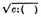
indicates the cube root.
2 Kepler, in his Astronomia nova stellae Martis (Heidelberg, 1609), showed that the problem of finding the position of a planet in its elliptical orbit leads to this other problem.
3 Newton’s letters were first published in J. Wallis, Opera mathematica, III (Oxford, 1699), 622–629; the letter of Gregory was first published in Correspondence of scientific men of the X V IIthcentury, ed. S. J. Rigaud (2 vols.; Oxford University Press, Oxford, 1841), II, 209. Gregory, like Newton, used his result to expand many functions into infinite series. He also discovered Taylor’s theorem; see Selection V.11. In a letter to Collins of February 15, 1671, we find as one of his results the series for tan-1x, or, more precisely (since the tangent for Gregory is a line), the expansion
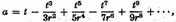
if r tan

( Gregory Memorial Volume, p. 170), first published in Commercium epistoIicum J. Collins et aliorum de analysi promota (London, 1712), 25–26. For

we obtain the series of Leibniz. On Collins see p. 290.
4 Tschirnhaus (see Selection II.11) wrote a letter to Oldenburg, dated September 1, 1676, of which Oldenburg informed Newton.
Contents:
Chicago:
Isaac Newton, "Binomial Series," A Source Book in Mathematics, 1200-1800, ed. H. W. Turnbull in A Source Book in Mathematics, 1200-1800, ed. D. J. Struik (Princeton: Princeton University Press, 1969, 1986), 284–290. Original Sources, accessed October 21, 2025, http://www.originalsources.com/Document.aspx?DocID=25RHR8TE8RT146W.
MLA:
Newton, Isaac. "Binomial Series." A Source Book in Mathematics, 1200-1800, edited by H. W. Turnbull, Vol. 1, in A Source Book in Mathematics, 1200-1800, edited by D. J. Struik, Princeton, Princeton University Press, 1969, 1986, pp. 284–290. Original Sources. 21 Oct. 2025. http://www.originalsources.com/Document.aspx?DocID=25RHR8TE8RT146W.
Harvard:
Newton, I, 'Binomial Series' in A Source Book in Mathematics, 1200-1800, ed. . cited in 1969, 1986, A Source Book in Mathematics, 1200-1800, ed. , Princeton University Press, Princeton, pp.284–290. Original Sources, retrieved 21 October 2025, from http://www.originalsources.com/Document.aspx?DocID=25RHR8TE8RT146W.
|