Mathematics

Weierstrass’s Al Functions

¹

To the system of equations relating integrals ["Integralgleichungen"] from which, according to Jacobi, one must begin in the theory of Abelian integrals ["Abelsche funktionen"], I shall give the following form, which I have recognized to be the simplest and most appropriate one. Let

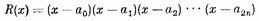
be a polynomial function of degree

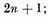
here I assume to begin with that the quantities are all real and ordered in such a way that

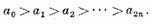
Now let R(x) be decomposed into two factors,

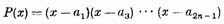
and

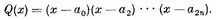
Considering

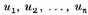
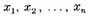
as independent variables and as functions of them, let the relations between these 2n variables be expressed by the following n equations:

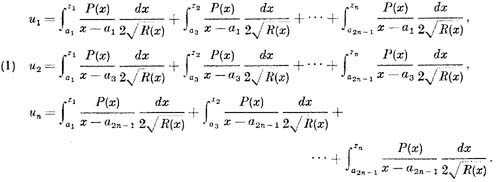
With the help of Abel’s Theorem, I now establish in detail the proposition already stated by Jacobi, which I consider the foundation of the whole theory, namely, that whereas for given values of

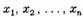
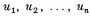
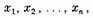
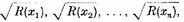
the quantities have infinitely many different values, if, conversely, are given, then the values of as well as the corresponding values of are uniquely determined. Moreover . . . 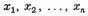are the roots of a [polynomial] equation of degree n whose coefficients are . . . single-valued functions of the independent variables 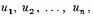while a second polynomial function of x, whose coefficients are the same kind of functions of 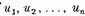gives for 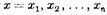the corresponding values of

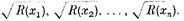
Therefore, every symmetric rational function of

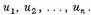
can be regarded as a single-valued function of In particular, it becomes evident that

where a signifies one of the integers

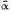
is the square of such a function. Accordingly, I denote by the largest integer less than or equal to a/2 and set

I also denote

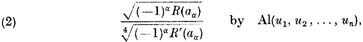
and call the

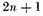
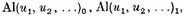
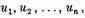
quantities etc., Abelian functions, since they are the ones which completely correspond to the elliptic functions² sn u, cn u, dn u. The series, expanded in powers of have the following form:

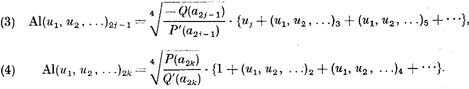
In these formulas j stands for any one of the integers

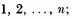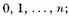
k for any of the integers and designates a homogeneous polynomial of degree α. I note generally that j and l as well as j′ and l′ will stand below for one of the integers k, on the other hand, will stand for one of the integers It is to be noted further that wherever there appears the root (of 2nd or 4th degree) of a positive expression, formed by multiplication and division of the differences etc., its positive value shall always be taken.

. . . . .

2.

Now I shall introduce those quantities which are analogous to the so-called complete elliptic integrals of the first kind. If x is real and lies between the limits

and a_α, then

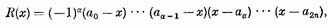
and hence one can set

where all roots are to be taken as positive.

Furthermore, if x lies between + ∞ and a₀,

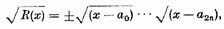
and if x lies between a_2n and – ∞:

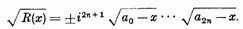
Now if a and b are two real quantities, and F(x) denotes a rational function of x, then I designate by

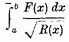
that particular value of the integral

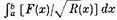
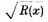
which is obtained if in the integration is always determined by the above formulas, using the upper [positive] sign. Assuming this, let

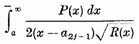
be designated by

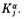
Then the relation

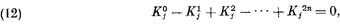
for

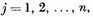
holds.

Further, let

so that

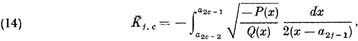
where

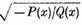
is to be taken as positive in the first integration, in the other one. Then it turns out that

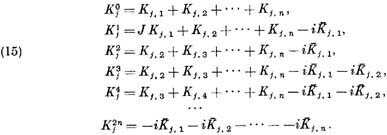
A special case of Abel’s Theorem then leads to the following formulas, in which α, β, γ denote numbers from the set

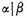
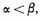
and the symbol denotes 0 when and 1 when

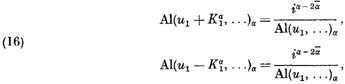
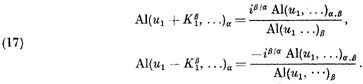
From this, one may deduce

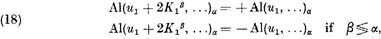
and if one sets

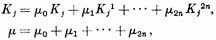
where

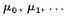
represent arbitrary integers, then

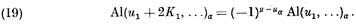
Now set

and

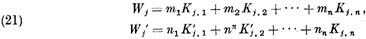
where

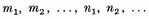
also designate arbitrary integers; one obtains the formulas

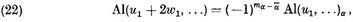
where, if

, m₀ must be taken equal to zero; and

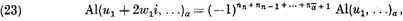
in which formula the factor of

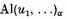
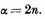
is to be set equal to 1 for With the help of formula (17), one obtains similar relations for the functions

The functions

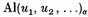
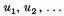
have the peculiar property ["Eigenthümlichkeit"] that they all have the same singularities ["für dieselben Betrâgen der unendlich werden"]. 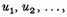Furthermore, as will be shown in the proof of the main theorem given in §1 (if one specifies fixed limits, arbitrarily large, for the absolute values of which they are not to exceed), each of the mcan be represented as the quotient of two series, expanded in positive integral powers of which converge for all values of these variables within the assigned limits. 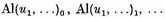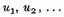This leads to the conjecture that might be represented as fractions with a common denominator, in which this denominator as well as the numerators would be functions of which would never become infinite, and could be expanded in uniformly convergent series of integral positive powers of their arguments. If (where p and p_α are functions of the type just described), then d log Al(u₁ , . . 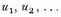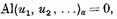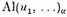.) mustconsist of two parts, one of which becomes infinite for all values of for which the other, for all those values which cause to become infinite. 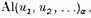The same is the case for the higher logarithmic differentials of Let us assume now that

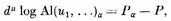
where

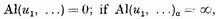
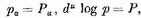
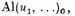
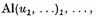
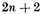
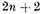
when then one can set d^u log and, since P_α, P can be expressed in terms of hence also in terms of one must arrive in this way at differential equations for the quantities indicated.

For elliptic functions, the calculation is very easy. For, if

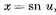
one has:

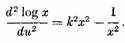
Now if one sets

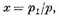
there results

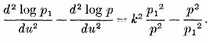
This equation can be factored, giving the two equivalent equations

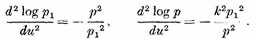
It has been shown previously that, if the absolute value of u has an upper bound M, sn u can be represented as the quotient of two series of integral powers of u, converging for all values of the variable less than M; hence it can be proved rigorously that the two functions p and p₁ defined by the two preceding differential equations can be expanded into uniformly convergent (for all real and imaginary values of u) series of positive integral powers of u. And if the four arbitrary constants which they contain are determined so that, for

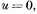
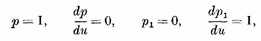
then, indeed,

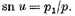
There are similar expansions for cn u, dn u, and in this way one arrives at those representations of elliptic functions which Abel had mentioned in a letter to Legendre³ without giving the details, or any indication of the procedure. From the functions p and p₁ one also obtains the Jacobian functions

Θ(u) and H(u) easily, and thus can carry out the development of elliptic functions in all forms without basing them on transformation or multiplication formulas. The above differential equations for p and p₁ can be made a basis for the complete theory of elliptic transcendental functions.

¹ K. Weierstrass "Zur Theorie der Abelsche Funktionen," J. reine ang. Math. 52 (1856), 285–339; Werke, I, 297–355.

² Like Jacobi, Weierstrass wrote sin am u, cos am u, Δ am u where we have written sn u, cn u, and dn u.

³ See J. reine ang. Math., vol. 4, p. 244; also vol. 6, p. 76 (K. W.)