Science
XXXIII James Clerk Maxwell 1831–1879
THE MAXWELL AND HERTZ THEORY OF ELECTRICITY AND LIGHT*
It was at the moment when the experiments of Fresnel were forcing the scientific world to admit that light consists of the vibrations of a highly attentuated fluid filling interplanetary spaces that the researches of Ampère were making known the laws of the mutual action of currents and were so enunciating the fundamental principles of electro-dynamics.
It needed but one step to the supposition that that same fluid, the ether, which is the medium of luminous phenomena, is at the same time the vehicle of electrical action. In imagination Amère made this stride; but the illustrious physicist could not foresee that the seducing hypothesis with which he was toying, a mere dream for him, was ere long to take a precise form and become one of the vital concerns of exact science.
A dream it remained for many years, till one day, after electrical measurements had become extremely exact, some physicist, turning over the numerical data, much as a resting pedestrian might idly turn over a stone, brought to light an odd coincidence. It was that the factor of transformation between the system of electro-statical units and the system of electro-dynamical units was equal to the velocity of light. Soon the observations directed to this strange coincidence became so exact that no sane head could longer hold it a mere coincidence. No longer could it be doubted that some occult affinity existed between optical and electrical phenomena. Perhaps, however, we might be wondering to this day what this affinity could be were it not for the genius of Clerk Maxwell.
DISPLACEMENT CURRENTS
The reader is aware that solid bodies are divided into two classes, conductors through which electricity can move in the form of a galvanic current, and nonconductors, or dielectrics. The electricians of former days regarded dielectrics as quite inert, having no part to play but that of obstinately refusing passage to electricity. Had that been so, any one nonconductor might be replaced by any other without making any difference in the phenomena; but Faraday found that that was not the case. Two condensers of the same form and dimensions put into connection with the same source of electricity do not take the same charge, though the thickness of the isolating plate be the same, unless the matter of that plate be chemically the same. Now Clerk Maxwell had too deeply studied the researches of Faraday not to comprehend the importance of dielectrics and the imperative obligation to recognize their active part.
Besides, if light is but an electric phenomenon, when it traverses a thickness of glass electrical events must take place in that glass. And what can be the nature of those events? Maxwell boldly answers, they are, and must be, currents.
All the experience of his day seemed to contradict this. Never had currents been observed except in conductors. How was Maxwell to reconcile his audacious hypothesis with a fact so well established as that? Why is it that under certain circumstances those supposed currents produce manifest effects, while under ordinary conditions they can not be observed at all?
The answer was that dielectrics resist the passage of electricity not so much more than conductors do, but in a different manner. Maxwell’s idea will best be understood by a comparison.
If we bend a spring, we meet a resistance which increases the more the spring is bended. So, if we can only dispose of a finite force, a moment will come when the motion will cease, equilibrium being reached. Finally, when the force ceases the spring will in flying back restore the whole of the energy which has been expended in bending it.
Suppose, on the other hand, that we wish to displace a body plunged into water. Here again a resistance will be experienced, but it will not go on increasing in proportion as the body advances, supposing it to be maintained at a constant velocity. So long as the motive force acts, equilibrium will never, then, be attained; nor when the force is removed will the body in the least tend to return, nor can any portion of the energy expended be restored. It will, in fact, have been converted into heat by the viscosity of the water.
The contrast is plain; and we ought to distinguish elastic resistance from viscous resistance. Using these terms, we may express Maxwell’s idea by saying that dielectrics offer an elastic resistance, conductors a viscous resistance, to the movements of electricity. Hence, there are two kinds of currents; currents of displacement which traverse dielectrics and ordinary currents of conduction which circulate in conductors.
Currents of the first kind, having to overcome an elastic resistance which continually increases, naturally can last but a very short time, since a state of equilibrium will quickly be reached.
Currents of conduction, on the other hand, having only a viscous resistance to overcome, must continue so long as there is any electromotive force.
Let us return to the simile used by M. Cornu in his notice in the Annuaire du Bureau des Longitudes for 1893. Suppose we have in a reservoir water under pressure. Lead a tube plumb downward into the reservoir. The water will rise in the tube, but the rise will stop when hydrostatic equilibrium is attained—that is, when the downward pressure of the water in the tube above the point of application of the first pressure on the reservoir, and due to the weight of the water, balances that first pressure. If the pipe is large, there will be no friction or loss of head, and the water so raised can be used to do work. That represents a current of displacement.
If, on the other hand, the water flows out of the reservoir by a horizontal pipe, the motion will go on till the reservoir is emptied; but if the tube is small and long there will be a great loss of energy and considerable production of heat by friction. That represents a current of conduction.
Though it would be vain, not to say idle, to attempt to represent all details, it may be said that everything happens just as if the currents of displacement were acting to bend a multitude of little springs. When the currents cease, electrostatic equilibrium is established, and the springs are bent the more, the more intense is the electric field. The accumulated work of the springs—that is, the electrostatic energy—can be entirely restored as soon as they can unbend, and so it is that we obtain mechanical work when we leave the conductors to obey the electrostatic attractions. Those attractions must be due to the pressure exercised on the conductors by the bent springs. Finally, to pursue the image to the death, the disruptive discharge may be compared to the breaking of the springs when they are bent too much.
On the other hand, the energy employed to produce conduction currents is lost, being wholly converted into heat, like that spent in overcoming the viscosity of fluids. Hence it is that the conducting wires become heated.
From Maxwell’s point of view it seems that all currents are in closed circuits. The older electricians did not so opine. They regarded the current circulating in a wire joining the two poles of a pile as closed; but if in place of directly uniting the two poles we place them in communication with the two armatures of a condenser, the momentary current which lasts while the condenser is getting charged was not considered as a current round a closed circuit. It went, they thought, from one armature through the wire, the battery, the other wire, to the other armature, and there it stopped. Maxwell, on the contrary, supposed that in the form of a current of displacement it passes through the nonconducting plate of the condenser, and that precisely what brings it to cessation is the opposite electromotive force set up by the displacement of electricity in this dielectric.
Currents become sensible in three ways—by their heating effects, by their actions on other currents and on magnets, and by the induced currents to which they give rise. We have seen why currents of conduction develop heat and why currents of displacement do not. But Maxwell’s hypothetical currents ought at any rate to produce electromagnetic and inductive effects. Why do these effects not appear? The answer is, that it is because a current of displacement can not last long enough. That is to say, they can not last long in one direction. Consequently in a dielectric no current can long exist without alteration. But the effects ought to and will become observable if the current is continually reversed at sufficiently short intervals.
THE NATURE OF LIGHT
Such, according to Maxwell, is the origin of light. A luminiferous wave is a series of alternating currents produced in dielectrics, in air, or even in the interplanetary void, and reversed in direction a million of million of times per second. The enormous induction due to these frequent alternations sets up other currents in the neighboring parts of the dielectric, and so the waves are propagated.
Calculation shows that the velocity of propagation would be equal to the ratio of the units, which we know is the velocity of light.
Those alternative currents are a sort of electrical oscillation. Are they longitudinal, like those of sound, or are they transversal, like those of Fresnal’s ether? In the case of sound the air undergoes alternative condensations and rarefactions. The ether of Fresnal, on the other hand, behaves as if it were composed of incompressible layers capable only of slipping over one another. Were these currents in open paths, the electricity carried from one end to the other would become accumulated at one extremity. It would thus be condensed and rarefied like air, and its vibrations would be longitudinal. But Maxwell only admits currents in closed circuits; accumulation is impossible, and electricity behaves like the incomprehensible ether of Fresnel, with its transversal vibrations.
EXPERIMENTAL VERIFICATION
We thus obtain all the results of the theory of waves. Yet this was not enough to decide the physicists to adopt the ideas of Maxwell. It was a seductive hypothesis; but physicists consider hypotheses which lead to no distinct observational consequences as beyond the borders of their province. That province, so defined, no experimental confirmation of Maxwell’s theory invaded for twenty-five years.
What was wanted was some issue between the two theories not too delicate for our coarse methods of observation to decide. There was but one line of research along which any experimentum crucis was to be met with.
The old electro-dynamics makes electro-magnetic induction take place instantaneously; but according to Maxwell’s doctrine it propagates itself with the velocity of light.
The point was then to measure, or at least to make certain, a velocity of propagation of inductive effects. This is what the illustrious German physicist Hertz has done by the method of interferences.
The method is well known in its application to optical phenomena. Two luminous rays from one identical center interfere when they reach the same point after pursuing paths of different lengths. If the difference is one, two, or any whole number of wave lengths, the two lights re-enforce one another so that if their intensities are equal, that of their combination is four times as great. But if the difference is an odd number of half wave lengths, the two lights extinguish one another.
Luminiferous waves are not peculiar in showing this phenomenon; it belongs to every periodic change which is propagated with definite velocity. Sound interferes just as light does, and so must electro-dynamic induction if it is strictly periodic and has a definite velocity of propagation. But if the propagation is instantaneous there can be no interference, since in that case there is no finite wave length.
The phenomenon, however, could not be observed were the wave length greater than the distance within which induction is sensible. It is therefore requisite to make the period of alternation as short as possible.
ELECTRICAL EXCITERS
We can obtain such currents by means of an apparatus which constitutes a veritable electrical pendulum. Let two conductors be united by a wire. If they have not the same electric potential the electrical equilibrium is disturbed and tends to restore itself, just as the molar equilibrium is disturbed when a pendulum is carried away from the position of repose.
A current is set up in the wire, tending to equalize the potential, just as the pendulum begins to move so as to be carried back to the position of repose. But the pendulum does not stop when it reaches that position. Its inertia carries it farther. Nor, when the two electrical conductors reach the same potential, does the current in the wire cease. The equilibrium instantaneously existing is at once destroyed by a cause analogous to inertia, namely self-induction. We know that when a current is interrupted it gives rise in parallel wires to an induced current in the same direction. The same effect is produced in the circuit itself, if that is not broken. In other words, a current will persist after the cessation of its causes, just as a moving body does not stop the instant it is no longer driven forward.
When, then, the two potentials become equal, the current will go on and give the two conductors relative charges opposite to those they had at first. In this case, as in that of the pendulum, the position of equilibrium is passed, and a return motion is inevitable. Equilibrium, again instantaneously attained, is at once again broken for the same reason; and so the oscillations pursue one another unceasingly.
Calculation shows that the period depends on the capacity of the conductors in such a way that it is only necessary to diminish that capacity sufficiently (which is easily done) to have an electric pendulum capable of producing an alternating current of extremely short period.
All that was well enough known by the theoretical researches of Lord Kelvin and by the experimentation of Federson on the oscillatory discharge of the Leyden jar. It was not that which constituted the originality of Hertz.
But it is not enough to construct a pendulum; it is further requisite to set it into oscillation. For that, it is necessary to carry it off from equilibrium and to let it go suddenly, that is to say, to release it in a time short as compared to the period of its oscillation.
For if, having pulled a pendulum to one side by a string, we were to let go of the string more slowly than the pendulum would have descended of itself, it would reach the vertical without momentum, and no oscillation would be set up.
In like manner, with an electric pendulum whose natural period is, say, a hundred-millionth of a second, no mechanical mode of release would answer the purpose at all, sudden as it might seem to us with our more than sluggish conceptions of promptitude. How, then, did Hertz solve the problem?
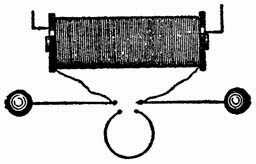
To return to our electric pendulum, a gap of a few millimeters is made in the wire which joins the two conductors. This gap divides our apparatus into two symmetrical parts, which are connected to the two poles of a Ruhmkorff coil. The induced current begins to charge the two conductors, and the difference of their potential increases with relative slowness.
At first the gap prevents a discharge from the conductors; the air in it plays the rôle of insulator and maintains our pendulum in a position diverted from that of equilibrium.
But when the difference of potential becomes great enough, a spark will jump across. If the self-induction is great enough and the capac-city and resistance small enough, there will be an oscillatory discharge whose period can be brought down to a hundred-millionth of a second. The oscillatory discharge would not, it is true, last long by itself; but it is kept up by the Ruhmkorff coil, whose current is itself oscillatory with a period of about a hundred-thousandth of a second, and thus the pendulum gets a new impulse as often as that.
The instrument just described is called a resonance exciter. It produces oscillations which are reversed from a hundred million to a thousand million times per second. Thanks to this extreme frequency, they can produce inductive effects at great distances. To make these effects sensible another electric pendulum is used, called a resonator. In this the coil is suppressed. It consists simply of two little metallic spheres very near to one another, with a long wire connecting them in a roundabout way.
The induction due to the exciter will set the resonator in vibration the more intensely the more nearly the natural periods of vibration are the same. At certain phases of the vibration the difference of potential of the two spheres will be just great enough to cause the sparks to leap across.
PRODUCTION OF THE INTERFERENCES
Thus we have an instrument which reveals the inductive waves which radiate from the exciter. We can study them in two ways. We may either expose the resonator to the direct induction of the exciter at a great distance, or else make this induction act at a small distance on a long conducting wire which the electric wave will follow and which in its turn will act at a small distance on the resonator.
Whether the wave is propagated along a wire or across the air, interferences can be produced by reflection. In the first case it will be reflected at the extremity of the wire, which it will again pass through in the opposite direction. In the second case it can be reflected on a metallic leaf which will act as a mirror. In either case the reflected ray will interfere with the direct ray, and positions will be found in which the spark of the resonator will be extinguished.
Experiments with a long wire are the easier and furnish much valuable information, but they cannot furnish an experimentum crucis, since in the old theory, as in the new, the velocity of the electric wave in a wire should be equal to that of light. But experiments on direct induction at great distances are decisive. They not only show that the velocity of propagation of induction across air is finite, but also that it is equal to the velocity of the wave propagated along a wire, conformably to the ideas of Maxwell.
SYNTHESIS OF LIGHT
I shall insist less on other experiments of Hertz, more brilliant but less instructive. Concentrating with a parabolic mirror the wave of induction that emanates from the exciter, the German physicist obtained a true pencil of rays of electric force, susceptible of regular reflection and refraction. These rays, were the period but one-millionth of what it is, would not differ from rays of light. We know that the sun sends us several varieties of radiations, some luminiferous, since they act on the retina, others dark, infra-red, or ultra-violet, which reveal themselves in chemical and calorific effects. The first owe the qualities which render them sensible to us to a physiological chance. For the physicist, the infra-red differs from red only as red differs from green; it simply has a greater wave length. That of the Hertzian radiations is far greater still, but they are mere differences of degree, and if the ideas of Clerk Maxwell are true, the illustrious professor of Bonn has effected a genuine synthesis of light.
CONCLUSION
Nevertheless, our admiration for such unhoped-for successes must not let us forget what remains to be accomplished. Let us endeavor to take exact account of the results definitely acquired.
In the first place, the velocity of direct induction through air is finite; for otherwise interferences could not exist. Thus the old electro-dynamics is condemned. But what is to be set up in its place? Is it to be the doctrine of Maxwell, or rather some approximation to that, for it would be too much to suppose that he had foreseen the truth in all its details? Though the probabilities are accumulating, no complete demonstration of that doctrine has ever attained.
We can measure the wave length of the Hertzian oscillations. That length is the product of the period into the velocity of propagation. We should know the velocity if we knew the period; but this last is so minute that we cannot measure it; we can only calculate it by a formula due to Lord Kelvin. That calculation leads to figures agreeable to the theory of Maxwell; but the last doubts will only be dissipated when the velocity of propagation has been directly measured. (See Note I.)
But this is not all. Matters are far from being as simple as this brief account of the matter would lead one to think. There are various complications.
In the first place, there is around the exciter a true radiation of induction. The energy of the apparatus radiates abroad, and if no source feeds it, it quickly dissipates itself and the oscillations are rapidly extinguished. Hence arises the phenomenon of multiple resonance, discovered by Messrs. Sarasin and De la Rive, which at first seemed irreconcilable with the theory.
On the other hand, we know that light does not exactly follow the laws of geometrical optics, and the discrepancy, due to diffraction, increases proportionately to the wave length. With the great waves of the Hertzian undulations these phenomena must assume enormous importance and derange everything. It is doubtless fortunate, for the moment at least, that our means of observation are as coarse as they are, for otherwise the simplicity which struck us would give place to a dedalian complexity in which we should lose our way. No doubt a good many perplexing anomalies have been due to this. For the same reason the experiments to prove a refraction of the electrical waves can hardly be considered as demonstrative.
It remains to speak of a difficulty still more grave, though doubtless not insurmountable. According to Maxwell, the coefficient of electrostatic induction of a transparent body ought to be equal to the square of its index of refraction. Now this is not so. The few bodies which follow Maxwell’s law are exceptions. The phenomena are plainly far more complex than was at first thought. But we have not yet been able to make out how matters stand, and the experiments conflict with one another.
Much, then, remains to be done. The identity of light with a vibratory motion in electricity is henceforth something more than a seductive hypothesis; it is a probable truth. But it is not yet quite proved.
NOTE I.—Since the above was written another great step has been taken. M. Blondlot has virtually succeeded, by ingenious experimental contrivances, in directly measuring the velocity of a disturbance along a wire. The number found differs little from the ratio of the units; that is, from the velocity of light, which is 300,000 kilometers per second. Since the interference experiments made at Geneva by Messrs. Sarasin and De la Rive have shown, as I said above, that induction is propagated in air with the same velocity as an electric disturbance which follows a conducting wire, we must conclude that the velocity of the induction is the same as that of light, which is a confirmation of the ideas of Maxwell.
M. Fizeau had formerly found for the velocity of electricity a number far smaller, about 180,000 kilometers. But there is no contradiction. The currents used by M. Fizeau, though intermittent, were of small frequency and penetrated to the axis of the wire, while the currents of M. Blondlot, oscillatory and of very short period, remained superficial and were confined to a layer of less than a hundredth of a millimeter in thickness. One may readily suppose the laws of propagation are not the same in the two cases.
NOTE II.—I have endeavored above to render the explanation of the electrostatic attractions and of the phenomena of induction comprehensible by means of a simile. Now let us see what Maxwell’s idea is of the cause which produces the mutual attractions of currents.
While the electrostatic attractions are taken to be due to a multitude of little springs—that is to say, to the elasticity of the ether—it is supposed to be the living force and inertia of the same fluid which produce the phenomena of induction and electrodynamical effects.
The complete calculation is far too extended for these pages, and I shall again content myself with a simile. I shall borrow it from a well known instrument—the centrifugal governor.
The living force of this apparatus is proportional to the square of the angular velocity and to the square of the distance of the balls.
According to the hypothesis of Maxwell, the ether is in motion in galvanic currents, and its living force is proportional to the square of the intensity of the current, which thus correspond, in the parallel I am endeavoring to establish, to the angular velocity of rotation.
If we consider two currents in the same direction, the living force, with equal intensity, will be greater the nearer the currents are to one another. If the currents have opposite directions, the living force will be greater the farther they are apart.
In order to increase the angular velocity of the regulator and consequently its living force, it is necessary to supply it with energy and consequently to overcome a resistance which we call its inertia.
In the same way, in order to increase the intensity of a current, we must augment the living force of the ether, and it will be necessary to supply it with energy and to overcome a resistance which is nothing but the inertia of the ether and which we call the induction.
The living force will be greater if the currents are in the same direction and near together. The energy to be furnished the counter electomotive force of induction will be greater. This is what we express when we say that the mutual action of two currents is to be added to their self-induction. The contrary is the case when their directions are opposite.
If we separate the balls of the regulator, it will be necessary, in order to maintain the angular velocity, to furnish energy, because with equal angular velocity the living force is greater the more the balls are separated.
In the same way, if two currents have the same direction and are brought toward one another, it will be necessary, in order to maintain the intensity to supply energy, because the living force will be augmented. We shall, therefore, have to overcome an electromotive force of induction which will tend to diminish the intensity of the currents. It would tend on the contrary to augment it, if the currents had the same direction and were carried apart, or if they had opposite directions and were brought together.
Finally, the centrifugal force tends to increase the distance between the balls, which would augment the living force were the angular velocity to be maintained.
In like manner, when the currents have the same direction, they attract each other—that is to say, they tend to approach each other, which would increase the living force if the intensity were maintained. If their directions are opposed they repel one another and tend to separate, which would again tend to increase the living force were the intensity kept constant.
Thus the electrostatic effects would be due to the elasticity of the ether and the electrodynamical phenomena to the living force. Now, ought this elasticity itself to be explained, as Lord Kelvin thinks, by rotations of small parts of the fluid? Different reasons may render this hypothesis attractive; but it plays no essential part in the theory of Maxwell, which is quite independent of it.
In the same way, I have made comparisons with divers mechanisms. But they are only similes, and pretty rough ones. A complete mechanical explanation of electrical phenomena is not to be sought in the volumes of Maxwell, but only a statement of the conditions which any such explanation has to satisfy. Precisely what will confer long life on the work of Maxwell is its being unentangled with any special mechanical hypothesis.
* Translated from a paper by M. Henri Poincaré.