Synthesis of the Elements in Stars

E. Margaret Burbidge, Geoffrey R. Burbidge, William A. Fowler, and Fred Hoyle

(Reviews of Modern Physics 29, 547–650 [1957])

I. INTRODUCTION

ELEMENT ABUNDANCES AND NUCLEAR STRUCTURE

MAN INHABITS A UNIVERSE composed of a great variety of elements and their isotopes. Ninety elements are found terrestrially and one more, technetium, is found in stars; only promethium has not been found in nature. Some 272 stable and 55 naturally radioactive isotopes occur on the earth. In addition, man has been able to produce artificially the neutron, technetium, promethium, and ten transuranic elements. The number of radioactive isotopes he has produced now numbers 871 and this number is gradually increasing.

Each isotopic form of an element contains a nucleus with its own characteristic nuclear properties which are different from those of all other nuclei. Thus the total of known nuclear species is almost 1,200, with some 327 of this number known to occur in nature. In spite of this, the situation is not as complex as it might seem. Research in "classical" nuclear physics since 1932 has shown that all nuclei consist of two fundamental building blocks. These are the proton and the neutron which are called nucleons in this context. As long as energies below the meson production threshold are not exceeded, all "prompt" nuclear processes can be described as the shuffling and reshuffling of protons and neutrons into the variety of nucleonic packs called nuclei. Only in the slow beta-decay processes is there any interchange between protons and neutrons at low energies, and even there, as in the prompt reactions, the number of nucleons remains constant. Only at very high energies can nucleons be produced or annihilated. Prompt nuclear processes plus the slow beta reactions make it possible in principle to transmute any one type of nuclear material into any other even at low energies of interaction.

With this relatively simple picture of the structure and interactions of the nuclei of the elements in mind, it is natural to attempt to explain their origin by a synthesis or buildup starting with one or the other or both of the fundamental building blocks. The following question can be asked: What has been the history of the matter, on which we can make observations, which produced the elements and isotopes of that matter in the abundance distribution which observation yields? This history is hidden in the abundance distribution of the elements. To attempt to understand the sequence of events leading to the formation of the elements it is necessary to study the so-called universal or cosmic abundance curve.

Whether or not this abundance curve is universal is not the point here under discussion. It is the distribution for the matter on which we have been able to make observations. We can ask for the history of that particular matter. We can also seek the history of the peculiar and abnormal abundances, observed in some stars. We can finally approach the problem of the universal or cosmic abundances. To avoid any implication that the abundance curve is universal, when such an implication is irrelevant, we commonly refer to the number distribution of the atomic species as a function of atomic weight simply as the atomic abundance distribution. In graphical form, we call it the atomic abundance curve.

Here Burbidge et al. present a table of the atomic abundances of the elements produced by the different processes. We do not reproduce this table here but illustrate the general behavior with figure 55.1. The processes accounting for the different stable isotopes are given in an appendix that we do not reproduce.

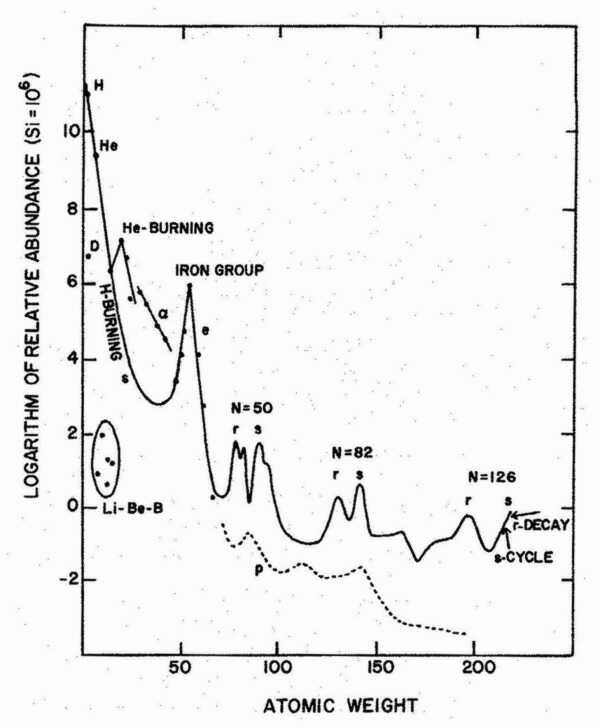Fig. 55.1 Schematic curve of atomic abundances as a function of atomic weight based on the data of Suess and Urey (Su56). Suess and Urey have employed relative isotopic abundances to determine the slope and general trend of the curve. There is still considerable spread of the individual abundances about the curve illustrated, but the general features shown are now fairly well established. These features are outlined in Table 55.1. Note the overabundances relative to their neighbors of the alpha-particle nuclei A = 16, 20, ... 40, the peak at the iron group nuclei, and the twin peaks at A = 80 and 90, at 130 and 138, and at 194 and 208.

The first attempt to construct such an abundance curve was made by Goldschmidt (Go37). An improved curve was given by Brown (Br49) and more recently Suess and Urey (Su56) have used the latest available data to give the most comprehensive curve so far available. These curves are derived mainly from terrestrial, meteoritic, and solar data, and in some cases from other astronomical sources. Abundance determinations for the sun were first derived by Russell (Ru29) and the most recent work is due to Goldberg, Aller, and Müller (Go57). Accurate relative isotopic abundances are available from mass spectroscopic data, and powerful use was made of these by Suess and Urey in compiling their abundance table. This table, together with some solar values given by Goldberg et al., forms the basic data for this paper.

It seems probable that the elements all evolved from hydrogen, since the proton is stable while the neutron is not. Moreover, hydrogen is the most abundant element, and helium, which is the immediate product of hydrogen burning by the pp chain and the CN cycle, is the next most abundant element. The packing-fraction curve shows that the greatest stability is reached at iron and nickel. However, it seems probable that iron and nickel comprise less than 1% of the total mass of the galaxy. It is clear that although nuclei are tending to evolve to the configurations of greatest stability, they are still a long way from reaching this situation.

It has been generally stated that the atomic abundance curve has an exponential decline to

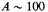
and is approximately constant thereafter. Although this is very roughly true it ignores many details which are important clues to our understanding of element synthesis. These details are shown schematically in figure 55.1 and are outlined in the left-hand column of table 55.1.

It is also necessary to provide an explanation of the origin of the naturally radioactive elements. Further, the existence of the shielded isobars presents a special problem.

FOUR THEORIES OF THE ORIGIN OF THE ELEMENTS Any completely satisfactory theory of element formation must explain in quantitative detail all of the features of the atomic abundance curve. Of the theories so far developed, three assume that the elements were built in a primordial state of the universe. These are the nonequilibrium theory of Gamow, Alpher, and Herman [see (A150)], together with the recent modifications by Hayashi and Nishida (Ha56), the poly-neutron theory of Mayer and Teller (Ma49), and the equilibrium theory developed by Klein, Beskow, and Treffenberg (K147). A detailed review of the history and development of these theories was given by Alpher and Herman (A153).

Each of these theories possesses some attractive features, but none succeeds in meeting all of the requirements. It is our view that these are mainly satisfied by the fourth theory in which it is proposed that the stars are the seat of origin of the elements. In contrast with the other theories which demand

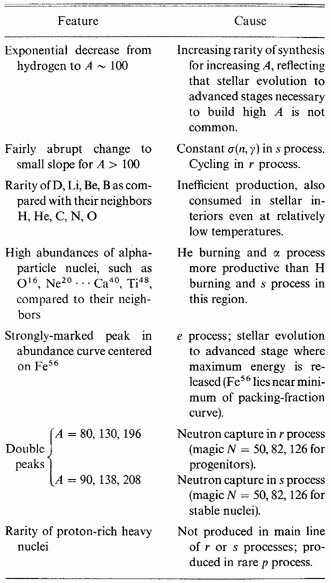Table 55.1 Features of the abundance curve

matter in a particular primordial state for which we have no evidence, this latter theory is intimately related to the known fact that nuclear transformations are currently taking place inside stars. This is a strong argument, since the primordial theories depend on very special initial conditions for the universe. Another general argument in favor of the stellar theory is as follows.

It is required that the elements, however they were formed, are distributed on a cosmic scale. Stars do this by ejecting material, the most efficient mechanisms being probably the explosive ejection of material in supernovae, the less energetic but more frequent novae, and the less rapid and less violent ejection from stars in the giant stages of evolution and from planetary nebulae. Primordial theories certainly distribute material on a cosmic scale but a difficulty is that the distribution ought to have been spatially uniform and independent of time once the initial phases of the universe were past. This disagrees with observation. There are certainly differences in composition between stars of different ages, and also stars at particular evolutionary stages have abnormalities such as the presence of technetium in the S-type stars and Cf²⁵⁴ in supernovae.

It is not known for certain at the present time whether all of the atomic species heavier than hydrogen have been produced in stars without the necessity of element synthesis in a primordial explosive stage of the universe. Without attempting to give a definite answer to this problem we intend in this paper to restrict ourselves to element synthesis in stars and to lay the groundwork for future experimental, observational, and theoretical work which may ultimately provide conclusive evidence for the origin of the elements in stars. However, from the standpoint of the nuclear physics alone it is clear that our conclusions will be equally valid for a primordial synthesis in which the initial and later evolving conditions of temperature and density are similar to those found in the interiors of stars.

GENERAL FEATURES OF STELLAR SYNTHESIS Except at catastrophic phases a star possesses a self-governing mechanism in which the temperature is adjusted so that the outflow of energy through the star is balanced by nuclear energy generation. The temperature required to give this adjustment depends on the particular nuclear fuel available. Hydrogen requires a lower temperature than helium; helium requires a lower temperature than carbon, and so on, the increasing temperature sequence ending at iron since energy generation by fusion processes ends here. If hydrogen is present the temperature is adjusted to hydrogen as a fuel, and is comparatively low. But if hydrogen becomes exhausted as stellar evolution proceeds, the temperature rises until helium becomes effective as a fuel. When helium becomes exhausted the temperature rises still further until the next nuclear fuel comes into operation, and so on. The automatic temperature rise is brought about in each case by the conversion of gravitational energy into thermal energy.

In this way, one set of reactions after another is brought into operation, the sequence always being accompanied by rising temperature. Since penetrations of Coulomb barriers occur more readily as the temperature rises it can be anticipated that the sequence will be one in which reactions take place between nuclei with greater and greater nuclear charges. As it becomes possible to penetrate larger and larger barriers the nuclei will evolve towards configurations of greater and greater stability, so that heavier and heavier nuclei will be synthesized until iron is reached. Thus there must be a progressive conversion of light nuclei into heavier ones as the temperature rises.

There are a number of complicating factors which are superposed on these general trends. These include the following.

The details of the rising temperature and the barrier effects of nuclear reactions at low temperatures must be considered.

The temperature is not everywhere the same inside a star, so that the nuclear evolution is most advanced in the central regions and least or not at all advanced near the surface. Thus the composition of the star cannot be expected to be uniform throughout. A stellar explosion does not accordingly lead to the ejection of material of one definite composition, but instead a whole range of compositions may be expected.

Mixing within a star, whereby the central material is mixed outward, or the outer material inward, produces special effects.

Material ejected from one star may subsequently become condensed in another star. This again produces special nuclear effects.

All of these complications show that the stellar theory cannot be simple, and this may be a point in favor of the theory, since the abundance curve which we are trying to explain is also not simple. Our view is that the elements have evolved, and are evolving, by a whole series of processes. These are explained in the following sections, and illustrated in figure 55.1.

II. PHYSICAL PROCESSES INVOLVED IN STELLAR SYNTHESIS, THEIR PLACE OF OCCURRENCE, AND THE TIME-SCALES ASSOCIATED WITH THEM

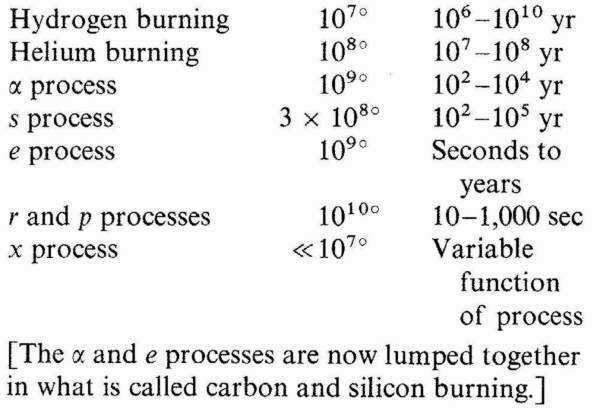
MODES OF ELEMENT SYNTHESIS As was previously described in an introductory paper on this subject by Hoyle, Fowler, Burbidge, and Burbidge (Ho56), it appears that in order to explain all of the features of the abundance curve, at least eight different types of synthesizing processes are demanded, if we believe that only hydrogen is primeval. In order to clarify the later discussion we give an outline of these processes here (see also Ho54, Fo56).

Hydrogen Burning Hydrogen burning is responsible for the majority of the energy production in the stars. By hydrogen burning in element synthesis we shall mean the cycles which synthesize helium from hydrogen and which synthesize the isotopes of carbon, nitrogen, oxygen, fluorine, neon, and sodium which are not produced by helium burning and the α process.

Helium Burning These processes are responsible for the synthesis of carbon from helium, and by further α-particle addition for the production of

and perhaps

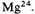
α Process These processes include the reactions in which α particles are successively added to

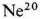
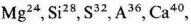
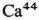
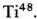
to synthesize the four-structure nuclei and probably and The source of the α particles is different in the α process than in helium burning.

e Process This is the so-called equilibrium process previously discussed by Hoyle (Ho46, Ho54) in which under conditions of very high temperature and density the elements comprising the iron peak in the abundance curve (vanadium, chromium, manganese, iron, cobalt, and nickel) are synthesized.

s Process This is the process of neutron capture with the emission of gamma radiation (n, γ) which takes place on a long time-scale, ranging from ~100 years to ~10⁵ years for each neutron capture. The neutron captures occur at a slow (s) rate compared to the intervening beta decays. This mode of synthesis is responsible for the production of the majority of the isotopes in the range

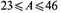
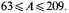
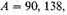
(excluding those synthesized predominantly by the α process), and for a considerable proportion of the isotopes in the range Estimates of the time-scales in different regions of the neutron-capture chain in the s process will be considered later in this section. The s process produces the abundance peaks at and 208.

r Process This is the process of neutron capture on a very short time-scale, ~0.01–10 sec for the beta-decay processes interspersed between the neutron captures. The neutron captures occur at a rapid (r) rate compared to the beta decays. This mode of synthesis is responsible for production of a large number of isotopes in the range

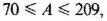
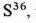
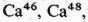
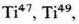
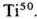
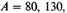
and also for synthesis of uranium and thorium. This process may also be responsible for some light element synthesis, e.g., and perhaps and The r process produces the abundance peaks at and 194.

p Process This is the process of proton capture with the emission of gamma radiation (p, γ), or the emission of a neutron following gamma-ray absorption (γ, n), which is responsible for the synthesis of a number of proton-rich isotopes having low abundances as compared with the nearby normal and neutron-rich isotopes.

x Process This process is responsible for the synthesis of deuterium, lithium, beryllium, and boron. More than one type of process may be demanded here (described collectively as the x process), but the characteristic of all of these elements is that they are very unstable at the temperatures of stellar interiors, so that it appears probable that they have been produced in regions of low density and temperature.

Here we omit the detailed specification of which of these eight processes are responsible for the synthesis of the different isotopes of the elements.

TIME-SCALES FOR DIFFERENT MODES OF SYNTHESIS Here Burbidge et al. discuss the internal stellar temperatures required for different modes of nucleosynthesis, together with the duration of each process. These very approximate considerations may be summarized as follows:

III. HYDROGEN BURNING, HELIUM BURNING, THE α PROCESS, AND NEUTRON PRODUCTION

This section and the sections to follow are devoted to detailed elaboration and discussion of the different physical processes introduced in Sec. II. These sections treat quantitatively experimental and theoretical evaluations of the cross sections and reaction rates of the nuclear processes involved in energy generation and element synthesis in stars. The material supplements and extends that published in a series of articles in 1954 (Fo54, Bo54, Ho54), in 1955 (Fo55, Fo55a), and in 1956 (Bu56). In the first part of this section we give a discussion of the relations between nuclear cross sections and nuclear reaction rates in stellar interiors and of the notation used in this and the following sections.

CROSS-SECTION FACTOR AND REACTION RATES The experimental results to be discussed will be used to derive the numerical value of the nuclear cross-section factor for a charged particle reaction defined by

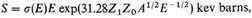
where σ(E) is the cross section in barns (10^-24 cm²) measured at the center-of-mass energy E in kev. The charges of the interacting particles are Z₁ and Z₀ in units of the proton charge and

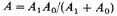
is their reduced mass in atomic mass units. S is measured in the center-of-mass system. From measurements made in the laboratory system with incident particle energy E₁ and with target nuclei at rest, the quantity S is given by

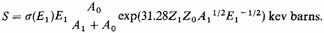
For a nonresonant or off-resonant reaction S is a slowly varying function of the energy E. Methods for extrapolating to the effective thermal energy E₀ in stellar interiors have been given by numerous authors (Sa52a, Fo54, Sa55, Ma57). The effective thermal energy at temperature T is

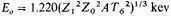
where T₆ is the temperature measured in units of 10⁶° K. The width of the effective range of thermal energy is

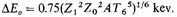
The mean reaction rate of a thermonuclear process may be expressed as

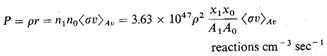
where n₁ and n₀ are the number densities of the interacting particles per cm³ and

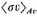
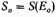
is the average of the cross section multiplied by the velocity in cm³ sec^-1. The quantity r is the reaction rate per gram per second. The quantities x₁ and x₀ are the amounts of the interacting nuclei expressed as fractions by weight. In terms of kev barns, it is found for a nonresonant process that

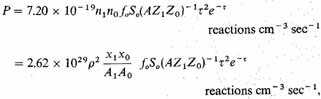
where S₀ is in kev barns and

(this τ is not to be confused with the mean lifetime of the interacting particles which will always be accompanied by appropriate subscripts, etc.). The term f₀ is the electron screening or shielding factor discussed by Salpeter (Sa54), evaluated at E₀. The cross-section factor S₀ as customarily calculated does not include allowance for electron screening.

The mean lifetime of the nuclei of type 0 for the interaction with nuclei of type 1 is given by

where v₀ is the number of nuclei of type 0 consumed in each reaction. The quantity p₁(0) is the mean reaction rate per nucleus of type 0. If nuclei of type 0 are regenerated in a cycle of reactions then

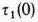
becomes the mean cycle time for nuclei of type 0.

The most satisfactory procedure for determining S₀ is to make experimental observations on cross sections over a range of energies not too large compared to E₀. The cross-section factor, S, can then be plotted as a function of E and an appropriate extrapolation to find S₀ can be made. This is not always possible and computational procedures for several frequently occurring cases will now be given.

Here we omit detailed formulae for the calculation of cross sections from experimentally determined parameters of a resonance that falls outside and within the range of

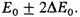
We also omit formulae for cross sections that are averages over several resonances and for the case of light nuclei, where the interaction energy may fall in the flat minimum between resonances. [Consult W. A. Fowler, C. R. Caughlan, and B. A. Zimmerman, Annual Reviews of Astronomy and Astrophysics 5, 525 (1967), 13, 69 (1975)].

PURE HYDROGEN BURNING The point of view that element synthesis begins with pure hydrogen (primordial or continuously produced) condensed in stars is based on the existence of the so-called direct pp chain of reactions by which hydrogen is converted into helium. This chain is initiated by the direct pp reaction

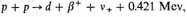
which has good theoretical foundations but which has not yet been observed experimentally in the laboratory because of its extremely low cross section even at relatively high interaction energies. In the above equation and in what follows we use v₊ for neutrinos emitted with positrons,

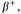
and v_- for antineutrinos emitted with electrons, 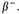We use nuclear rather than atomic mass differences in expressing the Q values of all reactions. There is of course practically no difference in atomic and nuclear Q values when positrons or electrons are not involved.

The calculated cross section for the pp reaction is

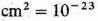
barn at 1-Mev laboratory energy. This is much too small for detection with currently available techniques.

Details of the reaction rate for the pp reaction are given for a new value of the beta-decay constant, and the results indicate that the energy generation of the proton-proton reaction is larger than that of the carbon-nitrogen cycle for central stellar temperatures less than

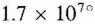
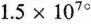
(with the sun at ); the opposite is true at higher temperatures.

PURE HELIUM BURNING When hydrogen burning in a star’s main-sequence stage leads eventually to hydrogen exhaustion, a helium core remains at the star’s center. It has been suggested (Sa52, Sa53, Op51, Op54) that the fusion of helium plays an important role in energy generation and element synthesis in the red-giant stage of the star’s evolution. The fusion occurs through the processes

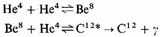
or, in a more condensed notation, through

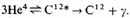
We refer to this as the 3α reaction. These processes are believed to occur at a late stage of the red giant evolution in which the hydrogen in the central core has been largely converted into helium, and in which gravitational contraction (Ho55) has raised the central temperature to ~10⁸ degrees, and the density to ~10⁵ g/cc. Under these conditions, as shown by Salpeter, an equilibrium ratio of

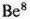
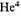
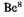
to nuclei equal to ~10^-9 is established. This conclusion followed from experimental measurements (He48, He49, To49, Wh41) which established the fact that was unstable to disintegration into alpha particles but only by 95 kev with an uncertainty of about 5 kev.

Rates for the triple alpha reaction and the alpha gamma reactions of

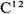
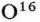
and are given.

α PROCESS As has just been discussed, helium-burning synthesizes

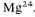
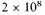
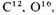
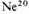
and perhaps a little This occurs at temperatures between 10⁸ and degrees and results in the exhaustion of the helium produced in hydrogen-burning. An inner core of and develops in the star and eventually undergoes gravitational contraction and heating just as occurred previously in the case of the helium core. Calculations of stellar evolutionary tracks have not yet been carried to this stage, but it is a reasonable extrapolation of current ideas concerning the cause of evolution into the giant stage. Gravitation is a "built-in" mechanism in stars which leads to the development of high temperature in the ashes of exhausted nuclear fuel. Gravitation takes over whenever nuclear generation stops; it raises the temperature to the point where the ashes of the previous processes begin to burn. Implicit in this argument is the assumption that mixing of core and surrounding zones does not occur.

No important reactions occur among

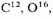
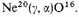
and until significantly higher temperatures, of the order of 10⁹ degrees, are attained. Two effects then arise. The γ rays present in the thermal assembly become energetic enough to promote The resulting transformation is strongly exothermic, viz.:

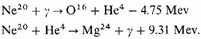
Combining these two equations yields:

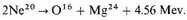
Additional alpha gamma reactions that build up heavier elements are discussed; these elements stand out in abundance above other neighboring nuclei.

SUCCESSION OF NUCLEAR FUELS IN AN EVOLVING STAR Starting with primeval hydrogen condensed into stars, pure hydrogen burning, pure helium burning, and finally the α process successively take place at the stellar center and then move outward in reaction zones or shells. When the star first contracts the generation of energy by hydrogen burning develops internal pressures which oppose gravitational contraction, and the star is stabilized on the main sequence at the point appropriate to its mass. Similarly, the generation of energy in helium burning should lead to a period of relative stability during the red-giant stage of evolution. It is assumed that mixing does not occur. This is substantiated by the fact that, as hydrogen becomes exhausted in the interior, the star evolves off the main sequence which is the location of stars with homogenous interiors.

At the end of helium burning most of the nuclear binding energy has been abstracted, and indeed the cycle of contraction, burning, contraction ... must eventually end when the available energy is exhausted, that is, when the most stable nuclei at the minimum in the packing fraction curve are reached, near

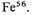
If a star which condensed originally out of pure hydrogen remains stable, it eventually forms the iron-group elements at its center, and this "iron core" continues to grow with time until gravitation, unopposed by further energy generation, leads eventually to a violent instability. At this point it suffices only to emphasize that the instability may result in the ejection of at least part of the "iron core" and its thin surrounding shells of lighter elements into the interstellar medium and that in this way a reasonable picture of the production of the abundance peak at the iron-group elements can be formulated. 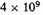Production of the iron group of elements requires temperatures near degrees, at which statistical equilibrium is reached (the e process).

BURNING OF HYDROGEN AND HELIUM WITH MIXTURES OF OTHER ELEMENTS; STELLAR NEUTRON SOURCES In the previous discussion we considered the effect of heating hydrogen and its reaction products to very high temperatures. First, the hydrogen is converted to helium, and the resulting helium is converted to

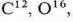
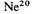
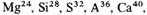
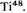
and Then α particles released by (γ, α) reactions on the build the α-particle nuclei and also and Finally, at very high temperatures, the latter nuclei are converted into the iron group. Further heating of the iron group, although of astrophysical importance, does not lead directly to any further synthesis. Thus all the remaining elements and isotopes must be provided for otherwise than by a cooking of pure hydrogen.

Very much more complicated reactions arise when we consider the cooking of hydrogen and helium mixed with small concentrations of the elements already provided for, e.g.,

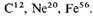
It is easy to see how such admixtures can arise. Since stars eject the products of nuclear synthesis into the interstellar gas it seems highly probable that only the "first" stars can have consisted of pure hydrogen. The results of hydrogen cooking in such stars would follow the lines described above. But once the interstellar gas was contaminated by this first cooking, nuclear processes would operate on hydrogen which contains impurities. The eventual hydrogen exhaustion will lead to helium burning with impurities. As we shall see later, the presence of other nuclei can lead to highly important effects.

In addition, hydrogen and helium may in some cases become adulterated with impurities even in the "first" stars. For example

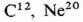
built in the inner central regions of such stars may be circulated into the outer hydrogen envelopes.

CN cycle When

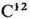
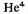
produced in helium burning is mixed with hydrogen at high enough temperatures, hydrogen is converted to by the CN cycle in addition to the pp chain previously considered. The implications for energy generation in hot main-sequence stars have been considered by numerous authors since Bethe (Be39) and von Weizsäcker (We38). The reactions of the CN cycle are

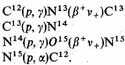
These four reactions produce

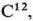
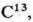
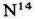
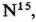
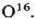
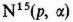
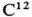
the heavier stable isotope of carbon, and the two stable forms of nitrogen. For and the (p, α) reaction is not exothermic and only the (p, γ) reaction occurs. At the (p, α) reaction becomes exothermic and much more rapid than the (p, γ) reaction, which serves only as a small leak of material to The reaction reproduces the original and a true cycle of reactions is established. Not only does this give rise to the catalytic conversion of hydrogen into helium until hydrogen is exhausted, but it also results in the carbon and nitrogen isotopes not being consumed in hydrogen burning.

The cross sections of the CN-cycle reactions have been under experimental investigation in the Kellogg Radiation Laboratory for some years and a review of the reaction rates in stars as known up to 1954 is included in the earlier paper by Fowler (Fo54), with numerical computations by Bosman-Crespin et al. (Bo54). New measurements of the CN-cycle reactions in the 100-kev range of interaction energies are now underway.

A detailed discussion of the CN reaction rates follows. This section is followed by a discussion of the burning of other nuclei in hydrogen and of stellar neutron sources.

IV, V, VI, VII, VIII, IX, X. We omit here a 43-page discussion of the myriad details of the e, s, r, p and x processes.

XI. VARIATIONS IN CHEMICAL COMPOSITION AMONG STARS, AND THEIR BEARING ON THE VARIOUS SYNTHESIZING PROCESSES

Different processes of element synthesis take place at different epochs in the life-history of a star. Thus the problem of element synthesis is closely allied to the problem of stellar evolution. In the last few years work from both the observational and theoretical sides has led to a considerable advance in our knowledge of stellar evolution. Theoretical work by Hoyle and Schwarzschild (Ho55) has been repeatedly mentioned since it affords estimates of temperatures in the helium core and in the hydrogen-burning shell of a star in the red-giant stage. However, the calculated model is for a star of mass 1.2 solar masses and a low metal content (~1/20 of the abundances given by Suess and Urey), and is thus intended to apply to Population II stars.

From the observational side, the attack has been through photometric observations of clusters of stars, from which their luminosities and surface temperatures have been plotted in color-magnitude or Hertzsprung-Russell (HR) diagrams, by many workers. We refer here only to two studies, by Johnson (Jo54) and by Sandage (Sa57a). A cluster may be assumed to consist of stars of approximately the same age and initial composition, and hence its HR diagram represents a "snapshot" of the stage to which evolution has carried its more massive members, once they have started to evolve fairly rapidly off the main sequence after their helium cores have grown to contain 10 to 30% of the stellar mass. Clusters of different ages will have main sequences extending upwards to stars of different luminosity, and the point at which its main sequence ends can be used to date a cluster; the rate of use of nuclear fuel, given by the luminosity, depends on the mass raised to a fairly high power (3 or 4).

Figure 55.2 is due to Sandage (Sa57a), and is a composite HR diagram of a number of galactic (Population I) clusters together with one globular cluster, M3 (Population II). The right-hand ordinate gives the ages corresponding to main sequences extending upwards to given luminosities. This diagram gives an idea of the way in which stars of different masses (which determine their position on the main sequence) evolve into the red-giant region. The most massive stars evolve into red supergiants (e.g., the cluster h and χ Persei); the difference between the red giants belonging to the Population I cluster M67 and the Population II cluster M3 should also be noted. Since stars evolve quite rapidly compared with the time they spend on the main sequence, the observed HR diagram may be taken as nearly representing the actual evolutionary tracks in the luminosity-surface-temperature plane.

A feature of Population II stellar systems is that their HR diagrams contain a "horizontal branch" [see, for example, the work by Arp, Baum, and Sandage (Ar53)] which probably represents their evolutionary path subsequent to the red-giant stage. This feature is not included in the diagram of M3 in figure 55.2, although it is well represented by that cluster, since this diagram is intended to show just the red-giant branches of clusters. The old Population 1 cluster M67 has a sparse distribution of stars which may lie on the Population I analogy of the horizontal branch; the other Population I clusters in figure 55.2 do not show such a feature. Presumably the evolutionary history of a more massive Population I star subsequent to its existence as a red giant is more rapid.

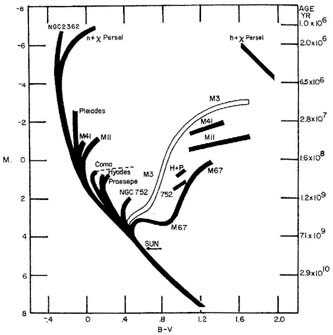Fig. 55.2 Composite Hertzsprung-Russell diagram of a number of galactic (Population I) star clusters, together with one globular cluster, M 3 (Population II), by Sandage (Sa57a). The abscissa measures the color on the B - V system and defines surface temperature (increasing from right to left). The left-hand ordinate gives the absolute visual magnitude, Mv, of the stars (showing that luminosity increases upward). The heavy black bands (Population I) and unfilled band (Population II) represent the regions in the temperature-luminosity plane that are occupied by stars. The name of each cluster is shown alongside the appropriate band. The right-hand ordinate gives the ages of the clusters, corresponding to main sequences extending upward to given luminosities. Note that the clusters all have a common main sequence below about Mv = +3.5 and also that the red giants have luminosities (defined by their masses) different from those they had while on the main sequence.

Whenever reference is made in different parts of this paper to particular epochs in a star’s evolutionary life, we are referring to a schematic evolutionary diagram for the star which has the same general characteristics as the HR diagrams in figure 55.2. With this background in mind, we turn now to astrophysical observations which provide many indications of element synthesis in stars. This is either taking place at the present time or else it has occurred over a time-scale spanned by the ages of nearby Population II stars.

A discussion of the N:C ratio during different stages of evolution follows. Certain stars exhibit

Table 55.2 A comparison between the chemical compositions of evolved stars and young stars

abundances different from the "normal" abundances given by Suess and Urey (Su56). These stars include the O or B star HD 160641 (A154), the hot subdwarf HZ 44 (Mu58), and the young stars τ Scorpii and 10 Lacertae (Tr55, Tr57). These abnormal abundances are given in table 55.2.

XII. GENERAL ASTROPHYSICS

EJECTION OF MATERIAL FROM STARS AND THE ENRICHMENT OF THE GALAXY IN HEAVY ELEMENTS Here Burbidge et al. argue that the helium and heavier elements present at the time of formation of Population I stars must have been ejected into interstellar space by red giants, supergiants, or supernovae. Elements synthesized in processes requiring temperatures of the order of 10⁹ or 10⁸° are ejected, respectively, from supernovae or the red giants and supergiants. The estimates of the total mass of material ejected from supernovae were made by assuming that an average supernova ejects 1.4 solar masses, that supernovae occur once every 300 years (Type I) or every 50 years (Type II), and that the age of the Galaxy is

years. The exponential-type light curve of Type I supernovae is taken to indicate the rapid production and capture of neutrons (r process). The estimate of the ejection of mass by red giants and supergiants was made by assuming that these stars eject most of their material and that stars that have gone through their whole evolution make up 45% of the total mass of stars (Sa55a). Assuming that 16% and 29% lie, respectively, in the form of white dwarfs and ejected gas, Burbidge et al. 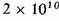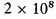conclude that some solar masses have been ejected into space by red giants and supergiants and about solar masses have been ejected into space by supernovae.

The estimates are not accurate enough to establish whether or not some helium was present in the original matter of the Galaxy. Deuterium could be produced through the capture of neutrons by hydrogen in the expanding envelopes of Type II supernovae or, perhaps, in the primeval big-bang explosion of the expanding universe. Because the Galaxy was originally all gas, and because it now contains about 10% by mass of neutral hydrogen (Hu56), star formation and element synthesis must have occurred at a greater rate early in the life of the Galaxy. The present value for the expansion rate of our galaxy (Hu56a) leads to an expansion age of

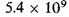
years, which according to Burbidge et al. is in conflict with the greater ages of some star clusters in the Galaxy.

SUPERNOVA OUTBURSTS In the following discussion we consider the course of evolution that in our view leads to the outbursts of supernovae. According to a well-known calculation by Chandrasekhar the pressure balance in a star cannot be wholly maintained by degeneracy for masses greater than a certain critical mass. For pure

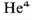
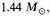
this critical mass turns out to be while for pure iron the value is

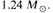
It follows that stars with masses greater than the critical value cannot be in mechanical support unless there is an appreciable temperature contribution to their internal pressures. Mechanical support therefore demands high internal temperatures in such stars.

Our arguments depend on these considerations. We are concerned with stars above the Chandrasekhar limit, and assume that mechanical support is initially operative to a high degree of approximation. This is not a restriction on the discussion, since our eventual aim will be to show that mechanical support ceases to be operative. A discussion of catastrophic stars would indeed be trivial if a lack of mechanical support were assumed from the outset.

The next step is to realize that a star (with mass greater than the critical value) must go on shrinking indefinitely unless there is some process by which it can eject material into space. The argument for this startling conclusion is very simple. Because of the high internal temperature, energy leaks outwards from the interior to the surface of the star, whence it is radiated into space. This loss of energy can be made good either by a slow shrinkage of the whole mass of the star (the shrinkage being "slow" means that mechanical support is still operative to a high degree of approximation), or by a corresponding gain of energy from nuclear processes. But no nuclear fuel can last indefinitely, so that a balance between nuclear energy generation and the loss from the surface of the star can only be temporary. For stars of small mass the permissible period of balance exceeds the present age of the galaxy, but this is not so for the stars of larger mass now under consideration. Hence for these stars shrinkage must occur, a shrinkage that is interrupted, but only temporarily, whenever some nuclear fuel happens for a time to make good the steady outflow of energy into space.

Shrinkage implies a rising internal temperature, since mechanical support demands an increasing thermal pressure as shrinkage proceeds. It follows that the internal temperature must continue to rise so long as the critical mass is exceeded, and so long as mechanical support is maintained. The nuclear processes consequent on the rise of temperature are, first, production of the α-particle nuclei at temperatures from

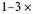
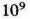
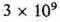
degrees and, second, production of the nuclei of the iron peak at temperatures in excess of degrees. It is important in this connection that the temperature is not uniform inside a star. Thus near the center, where the temperature is highest, nuclei of the iron peak may be formed, while outside the immediate central regions would be the α-particle nuclei, formed at a somewhat lower temperature, and still further outwards would be the light nuclei together with the products of the s process, formed at a much lower temperature. Indeed some hydrogen may still be present in the outermost regions of the star near the surface. The operation of the r process turns out to depend on such hydrogen being present in low concentration. The p process depends, on the other hand, on the outer hydrogen being present in high concentration.

Turning now to the nuclei of the iron peak, the statistical equations show that the peak is very narrow at

degrees, the calculated abundances falling away sharply as the atomic weight either decreases or increases from 56 by a few units. At higher temperatures the peak becomes somewhat wider, ranging to copper on the upper side and down to vanadium on the lower side. Beyond these limits the abundances still fall rapidly away to negligible values with one exception, the case of

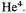
The statistical equation relating the abundances of

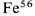
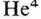
and is given here.

To convert 1 g of iron into helium demands an energy supply of

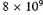
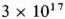
ergs. This may be compared with the total thermal energy of 1 gram of material at degrees which amounts to only ergs. Evidently the conversion of iron into helium demands a supply of energy much greater than the thermal content of the material. The supply must come from gravitation, from a shrinkage of the star, and clearly the shrinkage must be very considerable in order that sufficient energy becomes available. This whole energy supply must go into the conversion and hence into nuclear energy, so that very little energy is available to increase the thermal content of the material. However, instantaneous mechanical stability in such a contraction would demand a very large increase in the internal temperature. Thus we conclude that in this contraction, in which the thermal energy is, by hypothesis, only increased by a few percent, there is no mechanical stability, so that the contraction takes place by free fall inward of the central parts of the star. 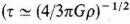At a density of 10⁸ g/cm³, this implies an implosion of the central regions in a time of the order of 1/5 of a second and in our view it is just this catastrophic implosion that triggers the outburst of a supernova.

Here Burbidge et al. conclude that the loss of energy by the neutrino emission of the Urca process is ineffective, compared with the refrigerating action of the conversion of

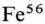
to

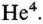
The last question to be discussed is the relationship between the implosion of the central regions of a star and the explosion of the outer regions. Two factors contribute to produce explosion in the outer regions. The temperature in the outer regions is very much lower than the central temperature. Because of this the outer material does not experience the same extensive nuclear evolution that the central material does. Particularly, the outer material retains elements that are capable of giving a large energy yield if they become subject to sudden heating, e.g.,

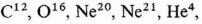
and perhaps even hydrogen. The second point concerns the possibility of the outer material experiencing a sudden heating. Because under normal conditions the surface temperature of a star is much smaller than the central temperature, material in the outer regions normally possesses a thermal energy per unit mass that is small compared with the gravitational potential energy per unit mass. Hence any abnormal process that causes the thermal energy suddenly to become comparable with the gravitational energy must lead to a sudden heating of the outer material. This is precisely the effect of an implosion of the central regions of a star. Consequent on implosion there is a large-scale conversion of gravitational energy into dynamical and thermal energy in the outer zones of the star.

One last point remains. Will the gravitational energy thus released be sufficient to trigger a thermonuclear explosion in the outer parts of the star? The answer plainly depends on the value of the gravitational potential. Explosion must occur if the gravitational potential is large enough. In a former paper (Bu56) it was estimated that a sudden heating to 10⁸ degrees would be sufficient to trigger an explosion. This corresponds to a thermal energy ~10¹⁶ ergs per gram. If the gravitational potential per gram at the surface of an imploding star is appreciably greater than this value, explosion is almost certain to take place. For a star of mass

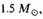
for instance, the gravitational potential energy per unit mass appreciably exceeds 10¹⁶ ergs per gram at the surface if the radius of the star is less than 10¹⁰ cm. At the highly advanced evolutionary state at present under consideration it seems most probable that this condition on the radius of the star is well satisfied. Hence it would appear as if implosion of the central regions of such stars must imply explosion of the outer regions.

Two cases may be distinguished, leading to the occurrence of the r and p processes. A star with mass only slightly greater than Chandrasekhar’s limit can evolve in the manner described above only after almost all nuclear fuels are exhausted. Hence any hydrogen present in the outer material must comprise at most only a small proportion of the total mass. This is the case of hydrogen deficiency that we associate with Type I supernovae, and with the operation of the r process. In much more massive stars, however, the central regions may be expected to exhaust all nuclear fuels and proceed to the point of implosion while much hydrogen still remains in the outer regions. Indeed the "central region" is to be defined in this connection as an innermost region containing a mass that exceeds Chandrasekhar’s limit. For massive stars the central region need be only a moderate fraction of the total mass, so that it is possible for a considerable proportion of the original hydrogen to survive to the stage where central implosion takes place. This is the case of hydrogen excess that in the former paper we associated with the Type II supernovae, where the p process may occur. However, the rarity of the p-process isotopes, and hence the small amount of material which must be processed to synthesize them, suggests that if Type II supernovae are responsible, the p process is a comparatively rare occurrence even among them. On the other hand, in any supernova in which a large flux of neutrons was produced, a small fraction of those having very high energies might escape to the outer parts of the envelope, and after decaying there to protons, might interact with the envelope material and produce the p-process isotopes.

Energy from the explosive thermonuclear reactions, perhaps as much as fifty percent of it, will be carried inwards, causing a heating of the material of the central regions. It is to this heating that we attribute the emission of the elements of the iron peak during the explosion of supernovae.

Supernova light curves are then given (see selection 70).

A150 R. A. Alpher and R. C. Herman, Revs. Modern Phys. 22, 153 (1950).

A153 R. A. Alpher and R. C. Herman, Ann. Rev. Nuclear Sci. 2, 1 (1953).

A154 L. H. Aller, Mem. soc. roy. sci. Liège 14, 337 (1954).

Ar53 Arp, Baum, and Sandage, Astron. J. 58, 4 (1953).

Be39 H. A. Bethe, Phys. Rev. 55, 103, 434 (1939).

Bo54 Bosman-Crespin, Fowler, and Humblet, Bull. soc. sci. Liège 9, 327 (1954).

Br49 H. Brown, Revs. Modern Phys. 21, 625 (1949).

Bu56 Burbidge, Hoyle, Burbidge, Christy, and Fowler, Phys. Rev. 103, 1145 (1956).

Fo54 W. A. Fowler, Mem. soc. roy. sci. Liège 14, 88 (1954).

Fo55 Fowler, Burbidge, and Burbidge, Astrophys. J. 122, 271 (1955).

Fo55a Fowler, Burbidge, and Burbidge, Astrophys. J. Suppl. 2, 167 (1955).

Fo56 W. A. Fowler and J. L. Greenstein, Proc. Natl. Acad. Sci. U.S. 42, 173 (1956).

Go37 V. M. Goldschmidt, Skrifter Norske Videnskaps-Acad. Oslo. I. Mat.-Naturv. Kl. No. 4 (1937).

Go57 L. Goldberg, E. A. Müller and L. H. Aller, Astrophys. J. Suppl. 5, 1 (1960).

Ha56 C. Hayashi and M. Nishida, Progr. Theoret. Phys. 16, 613 (1956).

He48 A. Hemmendinger, Phys. Rev. 73, 806 (1948).

He49 A. Hemmendinger, Phys. Rev. 75, 1267 (1949).

Ho46 F. Hoyle, Monthly Notices Roy. Astron. Soc. 106, 343 (1946).

Ho54 F. Hoyle, Astrophys. J. Suppl. 1, 121 (1954).

Ho55 F. Hoyle and M. Schwarzschild, Astrophys. J. Suppl. 2, 1 (1955).

Ho56 Hoyle, Fowler, Burbidge, and Burbidge, Science 124, 611 (1956).

Hu56 H. C. van de Hulst, Verslag Gewone Vergader. Afdel. Natuvrk. Koninkl. Ned. Akad. Wetenschap. 65, no. 10, 157 (1956).

Hu56a Humason, Mayall, and Sandage, Astron. J. 61, 97 (1956)

Jo54 H. L. Johnson, Astrophys. J. 120, 325 (1954).

K147 O. Klein, Arkiv mat. astron. fysik 34A, no. 19 (1947); F. Beskow and L. Treffenberg, Arkiv mat. astron. fysik 34A, nos. 13, 17 (1947).

Ma49 M. G. Mayer and E. Teller, Phys. Rev. 76, 1226 (1949).

Ma57 J. B. Marion and W. A. Fowler, Astrophys. J. 125, 221 (1957).

Mu58 G. Münch, Astrophys. J. 127, 642 (1958).

Op51 E. J. Öpik, Proc. Roy. Irish Acad. A54, 49 (1951).

Op54 E. J. Öpik, Mem. soc. roy. sci. Liège 14, 131 (1954).

Ru29 H. N. Russell, Astrophys. J. 70, 11 (1929).

Sa52 E. E. Salpeter, Astrophys. J. 115, 326 (1952).

Sa52a E. E. Salpeter, Phys. Rev. 88, 547 (1952).

Sa53 E. E. Salpeter, Ann. Rev. Nuclear Sci. 2, 41 (1953).

Sa54 E. E. Salpeter, Australian J. Phys. 7, 373 (1954).

Sa55 E. E. Salpeter, Phys. Rev. 97, 1237 (1955).

Sa55a E. E. Salpeter, Astrophys. J. 121, 161 (1955).

Sa57a A. R. Sandage, Astrophys. J. 125, 435 (1957).

Su56 H. E. Suess and H. C. Urey, Revs. Modern Phys. 28, 53 (1956).

To49 Tollestrup, Fowler, and Lauritsen, Phys. Rev. 76, 428 (1949).

Tr55 G. Traving, Z. Astrophys. 36, 1 (1955).

Tr57 G. Traving, Z. Astrophys. 41, 215 (1957)

We38 C. F. von Weizsäcker, Physik. Z. 39, 633 (1938).

Wh41 J. A. Wheeler, Phys. Rev. 59, 27 (1941).

1. W. D. Harkins, Journal of the American Chemical Society 39, 856 (1917), and Physical Review 38, 1270 (1931). Also see G. Oddo, Zeitschrift für anorganische Chemie 87, 253 (1914).

2. H. E. Suess, and H. C. Urey, Reviews of Modern Physics 28, 53 (1956). See also selection 39.

3. H. C. Urey and C. A. Bradley, Physical Review 38, 718 (1931); G. I. Pokrowski, Physikalische Zeitschrift 32, 374 (1931).

4. The work of E. Fermi and A. Turkevich was discussed by R. A. Alpher and R. C. Herman in the Reviews of Modern Physics 22, 153 (1950).

5. See R. V. Wagoner, W. A. Fowler, and F. Hoyle, Astrophysical Journal 148, 3 (1967), for details. Also see selections 13 and 131.

6. F. Hoyle, Astrophysical Journal Supplement 1, 121 (1954). See also F. Hoyle, Monthly Notices of the Royal Astronomical Society 106, 333 (1946).

7. F. Hoyle, W. A. Fowler, G. R. Burbidge, and E. M. Burbidge, Science 124, 611 (1956). The 1957 Reviews of Modern Physics article that constitutes this selection is reproduced in its entirety by the American Institute of Physics as Resource Letter OE-1 on the Origin of the Elements. See also A. G. W. Cameron, Publications of the Astronomical Society of the Pacific 69, 201 (1957); Chalk River Report CRL-41 (1957) and Atomic Energy of Canada Limited No. 454, 1 (1957).

8. W. D. Arnett and D. D. Clayton, Nature 227, 780 (1970).