Name: A Source Book in Astronomy and Astrophysics, 1900-1975 - Radio Waves from Space: Origin of Radiowaves
Author: Hendrik C. van de Hulst

Authors

Topics

Events

Contents

A Source Book in Astronomy and Astrophysics, 1900-1975

Contents:

Author: Hendrik C. van de Hulst | Date: 1945

Show Summary

General Summary

Although Karl Jansky discovered extraterrestrial radio radiation in the early 1930s (see selection 6), astronomers paid little attention to it until 1940, when Grote Reber confirmed Jansky’s discovery and two astrophysicists, Louis Henyey and Philip Keenan, developed Reber’s thesis that the radio emission comes from a hot interstellar gas. At that time the Dutch astronomer Jan Oort was actively involved in theoretical work on galactic structure, and when Reber published his radio map of the Milky Way in 1944, Oort asked a graduate student, Hendrik van de Hulst, to study and review at a colloquium the theory behind Reber’s observations. Oort also asked van de Hulst to see if there were any spectral lines in the radio spectrum.

Van de Hulst helped organize the colloquium at which he presented the paper reproduced here. His most startling prediction is the possibility of detecting a radio frequency spectral line from the neutral hydrogen (H I) regions. He realized that these regions would be cold and that most of the atoms would be in their lowest energy ground state. The electron of the hydrogen atom in this state has two possibilities for its spin, and a change from one to the other can give rise to an emission or absorption at a wavelength of 21 cm. As van de Hulst points out, these changes will occur rarely in the tenuous interstellar gas, but an observer might well detect them when looking through the vast extent of interstellar space. As we shall see in selection 92, this remarkable prediction led to the detection of interstellar neutral hydrogen and eventually to a description of the temperature, density, and motions of interstellar matter.

Van de Hulst also considered the lines emitted during the recombination of free electrons with free protons in ionized hydrogen (H II) regions. Through a numerical error in his original calculations, he incorrectly asserted that these lines will not be observable because of broadening by the Stark effect, but he was perhaps the first to call attention to the fact that these lines must occur at radio as well as optical wavelengths. In fact, subsequent theoretical considerations have shown that thermal motions dominate the broadening of these lines, and radio frequency recombination lines are now used to specify the physical conditions within H II regions.

When reviewing theoretical explanations for the continuum radio emission of the Milky Way, van de Hulst concluded that Reber’s observations were consistent with radiation from a hot interstellar gas but that Jansky’s observations at longer radio wavelengths were not. As Iosif Shklovskii subsequently pointed out, the radio emission of the ionized hydrogen (H II) regions predominates

at the shorter radio wavelengths, whereas a different mechanism, synchrotron radiation, predominates at long radio wavelengths.

In the final part of this paper, van de Hulst remarks on the tremendous importance of radio astronomy to cosmology. Here he notices that the Doppler effect, which weakens the light from distant galaxies, is not as important at radio wavelengths; therefore, deeper regions of the universe can be observed at radio wavelengths. Furthermore, by using the observations then available, van de Hulst is able to rule out the static universe in which red shift is not a Doppler effect. In this section, then, he anticipates much of the subsequent work on radio cosmology, which did not begin in earnest until over a decade later (see selection 118).

Radio Waves from Space: Origin of Radiowaves

Hendrik C. van de Hulst

TRANSLATED BY ELSA VAN DIEN AND VINCENT ICKE

(Nederlands tijdschrift voor natuurkunde 11, 210–221 [1945])^*

Summary—Radio waves received from any celestial object—they being the far infrared portion of its spectrum—deserve attention. Although observations of objects with small angular sizes are prevented by diffraction, the sun may be a measurable object for future instruments.

The radiation observed from our galaxy must be caused by the interstellar gas; the stars are ruled out by their small angular dimensions, and the solid smoke particles by their low temperature.

The spectral emission of a homogeneous layer of ionized hydrogen is computed. The continuous spectrum arising from free-free transitions has the intensity of blackbody radiation at wavelengths larger than 6 m and has a nearly constant intensity at wavelengths smaller than 2 m, corresponding to a large and to a small optical thickness, respectively. These intensities, shown in figure 91.1, agree with those computed by Henyey and Keenan and tally fairly well with the observations. No better accordance is to be expected, owing to the unknown electron density and extent of the interstellar gas and to unsatisfactory data about the directional sensitivity of the antenna.

Discrete lines of hydrogen are proved to escape observation. The 21.2-cm line, resulting from transitions between hyperfine structure components of the hydrogen ground level, might be observable if the lifetime of the upper level does not exceed

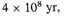
which, however, is improbable.

Reber’s observation of the Andromeda nebula suggests a rather high electron density. A cosmological remark concludes the article. The low background intensity caused by remote nebulae contradicts the Hubble-Tolman static model.

ASTRONOMICAL IMPORTANCE

ALTHOUGH THE EXISTENCE of radio waves of extraterrestrial origin has been known for about ten years, astronomers have not yet paid much attention to them. This neglect is partly due to the incomplete data at hand; not much more than an order of magnitude of the intensity and a rough dependence on direction of the radiation has been established. Hence, little is to be expected from a careful discussion of observational material.

Also, the existence of these radio waves is not very interesting from the purely theoretical point of view. The production of radio waves is by no means an essential feature of the physical condition of the interstellar matter. The amounts of energy transformed into radio radiation are so small that they are negligible in the large energy balance that starts with the ionization of interstellar atoms by the light of the stars. We cannot expect any new insight regarding the physical condition of the interstellar gas from purely theoretical considerations of the origin of these radio waves;¹ the condition of the interstellar gas is mainly characterized by its density, degree of ionization, and the distribution of electron velocities.

However, the possibility of direct observation of these waves has now made the subject attractive. For twenty centuries astronomers have obtained all their knowledge from observations in the rather narrow energy range around the visual frequencies. To this end, they built themselves powerful instruments and did not spare any trouble. Now we know that the earth’s atmosphere leaves open another frequency range, near the radio frequencies. The first observations have been made, but the technique of observing is still in its infancy. Bakker has shown that it can be improved greatly with means at present available.²

The long wavelengths involve one difficulty. Without telescopes of enormous apertures, the radio waves will never yield a detailed picture of the sky. For the time being we shall have to be satisfied to reach a resolving power of about 1°. The sun, the Milky Way, and the brightest extragalactic nebulae would then be measurable objects. In the Milky Way the run of the intensity with latitude and longitude, and especially the distribution of H I and H II regions of the interstellar gas, could be investigated. Moreover, it is possible that the very distant extragalactic nebulae would constitute a diffuse background, which would be of special cosmological interest.

THE SPECTRUM OF THE MILKY WAY

We shall try to derive, purely theoretically, the spectrum of the Milky Way at these frequencies. We extend our considerations over the entire width of the "radio window" in the earth’s atmosphere, that is, wavelengths from 20 m to 1 cm. The observations at wavelengths 14, 16, and 1.86 m yield intensities of equal order of magnitude. Although a continuous spectrum is probable, we do not want to exclude the possibility of discrete spectral lines. (No restriction is intended by speaking about the Milky Way; the Milky Way is what we observe of our stellar system with its threefold population: stars, interstellar smoke, and interstellar gas.) The maximum intensity of the radio waves has been observed in the direction of the constellation of Sagittarius. We know indeed, from many other data, that this is the direction of the galactic center, in which we look through the deepest and densest layers of our stellar system. I shall schematize a working model³ by assuming a homogeneous layer of constitution equal to what we know near the sun and a depth of 16,000

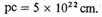
Firstly, we try to establish in which group of the population of our stellar system the observed radio waves chiefly originate. We use the law of radiation of a blackbody, which in this region, since

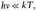
is the Rayleigh-Jeans law:

[Here j is the brightness of the blackbody in units of erg sec^-1 cm^-2 Hz^-1 rad^-2 at frequency v.]

STARS Stars probably radiate approximately like black-bodies, with a mean temperature of

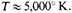
The surfaces of the sun and other stars therefore have an intensity denoted by the line

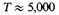
in figure 91.1, which increases in proportion to v². It does not seem impossible that in the future the sun might be measurable at decimeter wavelengths,⁴ although Reber’s method is not yet accurate enough to observe the sun. It is certain, moreover, that the stars together do not give a sky background of sufficient intensity; for the stellar discs certainly cover less than 1 part in 10¹⁰ of the area of the sky.

INTERSTELLAR SMOKE The solid particles of interstellar matter have a temperature of about 3° K. Even if they covered the whole sky, their contribution could be neglected. This conclusion is not changed by the greater details given by Whipple and Greenstein:⁵ namely, (1) the argument that too small a particle cannot radiate at all in this frequency range because it has no "eigen-frequency," and (2) the possibility that the smoke particles near the galactic center may have a temperature as high as 30° K, because of the greater energy density of stellar radiation.

INTERSTELLAR GAS The interstellar gas has a kinetic temperature of around 10,000° K; unlike the stars, it radiates from the whole area of the sky. The question is only if the layers are optically thick enough to yield blackbody radiation, and this question cannot be answered without going in some detail into the mechanism of emission.

Here van de Hulst gives a summary of the equations that describe radiation transfer and the intensity of radiation emitted by a homogeneous layer of gas.^6,7 He reproduces the classical expression for the volume emissivity,

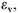
of a region of ionized hydrogen with electron density N_e and temperature T. During the encounters of free electrons with free protons a bremsstrahlung (braking radiation or free-free emission) is emitted with

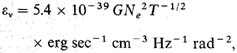
where the Gaunt factor, G, lies between 6 and 9 for the considered case. An optically thin region of total extent l has a brightness j_v, given by

whereas, because of self-absorption, an optically thick region has a brightness given by equation (1). Substituting

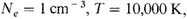
and

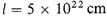
into equations (1) and (2), van de Hulst obtains the curved line in figure 91.1, where

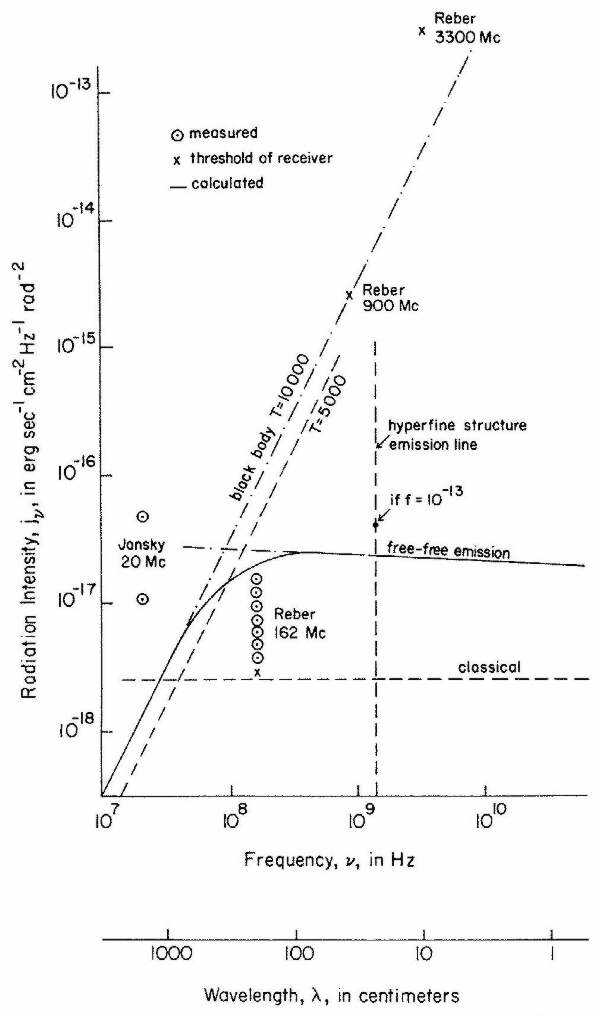Fig. 91.1 The theoretical and observed radiation spectra at radio frequencies. The thermal radiation of stars is denoted by the straight line T = 5,000°; and the free-free emission of a hot gas with temperature T = 10,000° K, electron density Ne = 1 cm-3, and extent l = 5 × 1022 cm is given by the solid curved line. The black dot denotes the expected intensity of the 21-cm transition of interstellar neutral hydrogen, and the dashed line denotes the classical calculations for free-free emission (which do not take into account the Gaunt factor). Reber’s observations are inconsistent with a stellar origin, and Jansky’s results are inconsistent with both the free-free and the stellar radiation mechanism.

the transition between optically thick and thin cases occurs at wavelengths between 1 and 10 m.

The agreement between the observed and the computed intensities is satisfactory. It is obvious why Reber has not found anything at the two short wavelengths. His sensitivity would have been just good enough for blackbody radiation, but the actual radiation is much weaker. Reber’s highest intensity, at 1.8 m, agrees well with the computed intensity in the direction of the center of the Milky Way. We must not let our joy over this discovery, however, cause us to overlook the large uncertainties. The observed intensities are first of all definitely uncertain by a factor of 2 higher, which makes the intensity a factor of 4 higher.

The comparison of Jansky’s observations with the theory comes off somewhat differently. Since the gas is optically thick at these wavelengths, the intensity depends only on the temperature. We must thus conclude that Jansky’s measured points in figure 91.1 lie too high by a factor of 10 or more. It seems quite possible to place most of the blame for this on the insufficiently known directional characteristics [of his antenna]. We cannot reasonably gain more than a factor of 2 by assuming a higher temperature.

ARE THERE ANY SEPARATE SPECTRAL LINES?

We have stated that the bound-bound transitions contribute, on the average, only a negligible amount to the continuum. However, the energy liberated in these transitions is emitted in separate lines, and we might expect that within the rather narrow lines the intensity will still be appreciably higher than in the continuum.

Here van de Hulst notices that the α transitions between levels with principal quantum numbers

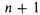
and n might be seen at radio wavelengths with

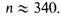
He asserts that Stark broadening of these lines should make them unobservable.⁸

All these lines, like the free-free continuum, will be formed only in the H II regions. But quite a different possibility remains. The ground level of hydrogen is split by hyperfine structure into two states of distance 0.047 cm^-1.⁹ The spins of the electron and the proton are in the one state parallel, in the other antiparallel. At the spontaneous reversal of the spin a quantum of 21.2 cm would be emitted. Of course, such a transition is forbidden. But the infrequent occurrence of this transition is compensated by the fact that in interstellar space the dilution of the radiation makes the ground state a preferred state over all others (including the free levels) by a factor of 10¹⁴. In the H I regions, where the ionized state does not occur, practically all atoms are in the ground state. We suspect that these are distributed about evenly over both sublevels.

It would be of tremendous interest if this line were observable. Without self-absorption, the intensity in the line is

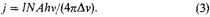
[Here A is the transition probability for spontaneous emission, l is the extent of the neutral hydrogen along the line of sight, N is the number density of the neutral hydrogen, h is Planck’s constant, v is the frequency of the hyperfine transition, and Δv is the width of the line.]

The value for A can be theoretically calculated but it is as yet unknown. I therefore substitute

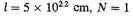
cm^-3, and

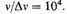
The contrast between line and continuum is sufficient if j is greater than the continuum value of

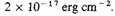
This condition is satisfied if A is greater than

that is, if the lifetime of the hydrogen atom in its highest hyperfine level is less than

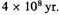
That does not seem to be an impossible requirement. With the usual definition of oscillator strength, f, this requirement amounts to

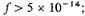
with Shortley’s definition¹⁰ of magnetic dipole strength, S, this becomes

Because S is usually of order unity, the case does not seem hopeless, not even if we consider that the low sensitivity of present receivers makes it necessary to increase the requirement by a factor of 100. However, there are reasons to believe that the true S will be rather smaller. Because the rigorous calculation has not been performed, the existence of this line remains speculative.

Van de Hulst next notices that Reber’s detection of radio radiation from the Andromeda nebula requires a much higher electron density in this object.

A COSMOLOGICAL REMARK

What do we observe of the remaining spiral nebulae? They are individually small, but together they should be able to produce a detectable background brightness. An even stronger statement can be made: if (1) the universe is perfectly homogeneous, (2) the spiral nebulae are all opaque, and (3) there exists no absorption between the nebulae, nor any other causes by which the distant nebulae are attenuated, then the entire sky must be covered with nebulae of a surface brightness equal to that of a nearby nebula. This argument has already been applied by Herschel to the stars surrounding us. The evident fact that the night sky is not everywhere as bright as the solar disk is a proof of the finite size of our galaxy. The "universe of stars" is not homogeneous.

Conclusions are not so easy to draw for the "universe of nebulae." We are now entering the domain of cosmology. Instead of distance we shall use the time T that the light from a nebula has required to reach us. Hubble’s observations with the 100-in telescope on Mount Wilson reach to

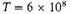
yr. Out to that distance about 1% of the sky area has already been covered, and yet no departures from homogeneity have been discovered. The extrapolation to a completely covered sky thus does not seem senseless.

The other causes by which the light from distant nebulae is weakened are also important. We see the distant nebulae in a very early stage of their development. The intensity is therefore uncertain, but we would sooner expect a higher than a lower brightness. The most important factor is the red shift. All the nebular light is shifted to longer wavelengths by an amount

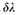
that, out to the deepest observation of

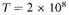
yr, is nearly always proportional to the distance. I thank Professor Oort for this essential point in the argument. The more distant nebulae have greater red shifts and less of the ultraviolet region of their spectra is photographed. The result is a considerable apparent attenuation of the observed light from the distant nebulae. In the radio region, the radiation of (originally) shorter wavelength received from the distant nebulae is almost as intense as that from the nearby nebulae. The radio region should therefore be very suitable for tracing the influence of the remaining effects on the background brightness of the sky.

I have in particular investigated whether a decision is possible between the two models put forward for comparison by Hubble and Tolman¹¹ to explain the numbers of nebulae photographed at Mount Wilson. The first model is an expanding universe where the red shift is due to the Doppler effect. In the second model the red shift is ascribed to an unknown cause, and the universe is static.

Van de Hulst then calculates the ratio of the brightness of the sky background to that of a nearby nebula for objects with spectral indices

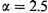
and

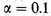
in either an expanding or a static universe.¹²

The rough calculation above indeed confirms the suggestion of Professor Oort. In the photographic region the background for both models is about 1% of the brightness of a nearby nebula. The results in the radio region, however, are entirely different. The expanding model again gives about 1% and the static model a divergent result. Therefore, we must take into account the shielding that causes a uniformly bright sky. We compare these calculations with the observations showing definitely that also in the radio region both the Milky Way and the Andromeda nebula stand out as bright objects against a dark background. Thus we may conclude that the static model is incorrect. We must bear in mind that this argument has only eliminated a certain combination of hypotheses; it is not possible to decide which of the hypotheses is wrong. Nevertheless, it is satisfying that these observations rule out a model that also has many theoretical objections.

1. G. Reber, Astrophysical Journal 91, 621 (1940), and Proceedings of the Institute of Radio Engineers 28, 68 (1940); L. G. Henyey and P. C. Keenan, Astrophysical Journal 91, 625 (1940).

2. I. S. Shklovskii, Astronomicheskii zhurnal 29, 418 (1952).

* A portion of this translation, by Elsa van Dien, originally appeared in H. Shapley (ed). Source Book in Astronomy 1900–1950 [Cambridge: Harvard University Press, 1960].

1. See the review in section 2 of the Astronomical Colloquium of the Nederlandsche Astronomen-Club No. 2, Ned. tijd. natuurkunde 10, 243 (1943), and the literature cited therein.

2. Ned. tijd. natuurkunde 11, 201 (1945).

3. Chosen in agreement with L. G. Henyey and P. C. Keenan, Ap. J. 91, 625 (1940).

4. In the meantime, Southworth (J. Franklin Inst. 239, 285 [1945]) has succeeded in measuring the solar radiation in the wavelength range 1–10 cm.

5. F. L. Whipple and J. L. Greenstein, Proc. Nat. Acad. Sci. 23, 177 (1937).

6. Full derivation by D. H. Menzel, Ap. J. 85, 330 (1937).

7. Taken from D. H. Menzel and C. L. Pekeris, M.N.R.A.S. 96, 77 (1935).

8. Cf. D. R. Inglis and E. Teller, Ap. J. 90, 439 (1939).

9. H. Kopfermann, Kernmomente (1940), p. 15, with substitution of

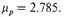
10. G. H. Shortley, Phys. Rev. 57, 225 (1940).

11. E. Hubble and R. C. Tolman, Ap. J. 82, 302 (1935).

12. O. Heckmann, Theorien der Kosmologie (Berlin, 1942), §17d.

Contents:

Related Resources

None available for this document.

Download Options

Title: A Source Book in Astronomy and Astrophysics, 1900-1975

Select an option:

*Note: A download may not start for up to 60 seconds.

Email Options

Title: A Source Book in Astronomy and Astrophysics, 1900-1975

Select an option:

Email addres:

*Note: It may take up to 60 seconds for for the email to be generated.

Chicago: Hendrik C. van de Hulst, "Radio Waves from Space: Origin of Radiowaves," A Source Book in Astronomy and Astrophysics, 1900-1975, trans. Elsa Van Dien and Vincent Icke in A Source Book in Astronomy and Astrophysics, 1900-1975, ed. Kenneth R. Lang and Owen Gingerich (Cambridge: Harvard University Press, 1979), 627–632. Original Sources, accessed December 12, 2024, http://www.originalsources.com/Document.aspx?DocID=8UDG98GEKHG81LI.

MLA: C. van de Hulst, Hendrik. "Radio Waves from Space: Origin of Radiowaves." A Source Book in Astronomy and Astrophysics, 1900-1975, translted by Elsa Van Dien and Vincent Icke, Vol. 11, in A Source Book in Astronomy and Astrophysics, 1900-1975, edited by Kenneth R. Lang and Owen Gingerich, Cambridge, Harvard University Press, 1979, pp. 627–632. Original Sources. 12 Dec. 2024. http://www.originalsources.com/Document.aspx?DocID=8UDG98GEKHG81LI.

Harvard: C. van de Hulst, H, 'Radio Waves from Space: Origin of Radiowaves' in A Source Book in Astronomy and Astrophysics, 1900-1975, trans. . cited in 1979, A Source Book in Astronomy and Astrophysics, 1900-1975, ed. , Harvard University Press, Cambridge, pp.627–632. Original Sources, retrieved 12 December 2024, from http://www.originalsources.com/Document.aspx?DocID=8UDG98GEKHG81LI.