Name: A Source Book in Astronomy and Astrophysics, 1900-1975 - Ionization in the Solar Chromosphere
Author: Meghnad Saha

A Source Book in Astronomy and Astrophysics, 1900-1975

Contents:

Author: Meghnad Saha | Date: 1920

Show Summary

General Summary

Stellar spectroscopy originated in 1817 when Joseph von Fraunhofer discovered that the continuum spectrum of the sun is crossed by an enormous number of dark lines. These observations received a physical explanation in 1859, when Gustav Kirchhoff used laboratory measurements to show that dark line spectra are caused by the absorption of a cool gas overlying a hot gas. By the late 1860s Kirchhoff, his associate Robert Bunsen, and the Swedish spectroscopist Anders Ångström had shown that the solar spectrum contains lines of hydrogen, sodium, calcium, barium, strontium, magnesium, copper, iron, chromium, nickel, cobalt, zinc, and gold. During the subsequent decade Norman Lockyer showed experimentally that elements exhibit different spectra under varying conditions of temperature and pressure and that, in particular, the arc and higher temperature spark spectra of the same element differed.

In order to achieve a quantitative analysis of stellar spectra, it was necessary to calculate the distribution of atoms in their various states, but before this could be done, a better understanding of the nature of the atom itself was required. Perhaps most fundamental were Joseph Thomson’s discovery of the electron in 1897 and Ernest Rutherford’s 1911 discovery that most of the atom’s mass and all its positive charge are concentrated in a nucleus near its center. The first successful attempt at explaining atomic spectra came with Niels Bohr, who pictured the atom in terms of specific electron orbits surrounding the massive atomic nucleus.

Shortly after Bohr had proposed his atomic model, a young physicist in Calcutta, Meghnad Saha, began a systematic study of the previous twenty-five years of the Monthly Notices of the Royal Astronomical Society. Thus, when he read J. Eggert’s 1919 paper on the dissociation of gases in Physikalische Zeitschrift, he was well prepared to begin the work on thermal ionization that can be considered the starting point of modern quantitative astrophysics. In 1919 Saha visited Alfred Fowler’s laboratory at Imperial College, London, and later Hermann Nernst’s laboratory in Berlin, so there is some question where he wrote the fundamental paper presented here. In any event, it was Edward Milne and Ralph Fowler who subsequently developed important extensions to the work and provided a quantitative theory of the spectral sequence.

In his analysis Saha, seeing the analogy between the dissociation of molecules and the ionization of atoms, merely replaced the mass of the atom with the mass of the electron in the well known expression for the degree of dissociation of a molecule to obtain his famous ionization equation.

Saha’s equation relates the degree of ionization of an atom to temperature and pressure, and therefore indicates that the relative intensities of different spectral lines are caused, in part, by differences in the pressure and temperatures of stellar atmospheres. For example, the application of Saha’s equation explained for the first time the apparent absence of rubidium and caesium in the sun: these elements, with low ionization potentials, are almost entirely ionized at the temperature of the solar photosphere.

Within a year after this paper, Saha had used his ionization equation to specify the temperatures of stars of different spectral types. In addition to confirming that the spectral sequence of stars is a temperature sequence, Saha’s result indicated that differences in stellar spectra are caused by differences in excitation rather than differences in chemical composition. It thus paved the way for the work of Cecilia Payne, who showed that stars with different spectra have essentially the same composition.

Ionization in the Solar Chromosphere

Meghnad Saha

(Philosophical Magazine 40, 479–488 [1920])

IT HAS BEEN KNOWN for a long time that the high-level chromosphere is generally distinguished by those lines which are relatively more strengthened in the spark than in the arc, and which Lockyer originally styled as enhanced lines.

Here Saha presents a table showing that the lines of calcium, strontium, barium, scandium, and titanium present in the chromosphere are more intense in the laboratory spark than in the laboratory flame.¹

It appears that no satisfactory explanation of this fact, as well as of the extraordinary height reached by these lines, has yet been offered. It is intimately connected with the physical mechanism of the arc and the spark. In this connexion, it is well to recall Lockyer’s original hypothesis, which, however, does not seem to have been, at any time, much in favour with the physicists. According to Lockyer, the passage from the arc to the spark means a great, though localised, increase of temperature, to which mainly the enhancement of the lines was to be ascribed. But, apart from its physical incompleteness, Lockyer’s theory launches us amidst great difficulties as far as the interpretation of solar phenomena is concerned. It would lead us to the hypothesis that the outer chromosphere is at a substantially higher temperature than the photosphere, and the lower chromosphere; and that the temperature of the sun increases as we pass radially outwards. This hypothesis is, however, quite untenable and is in flagrant contradiction to all accepted theories of physics.

A much more plausible explanation is that the lines in question are not due to radiations from the normal atom of the element, but from "an ionized atom, i.e., one which has lost an electron." The high-level chromosphere is, according to this view, the seat of very intense ionization. Let us see briefly how this hypothesis has grown up.

Modern theories of atomic structure and radiation leave little doubt that the "enhanced lines" are due to the ionized atom of the element. As a concrete example, let us take the case of the calcium H, K, and g lines. The "H, K" lines are of the enhanced type, while "g" is of the normal type. The "H, K" are the leading members of the principal pair-series of the system of double lines of Calcium, while the "g-" line is the first member of the system of single lines of Calcium. Lorenser and Fowler² have shown that the series formula of the double lines is of the type

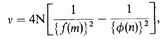
while the series formula of the single lines is of the type

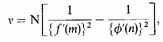
where f(m), φ(n) are functions of the form

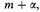
according to Rydberg, and

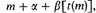
according to Ritz, t(m) being a function of m which vanishes with increasing values of m.

In other words, in the series formula of the enhanced lines, the spectroscopic constant is 4N instead of the usual Rydberg number N. In the light of Bohr’s theory, this is to be understood in the sense that, during the emission of the enhanced lines, the nucleus, and the system of electrons (excluding the vibrating one) taken together behave approximately as a double charge, so that the spectroscopic constant,

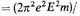
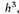
becomes 4N, as

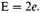
This means that if the nuclear charge is n, the total number of electrons is

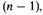
and the system has been produced by the removal of one electron from the normal atom.

What has been said of the Calcium lines H and K is also true of the Strontium pair 4,216 and 4,078, and the Barium

Table 38.1 Levels at which different chromospheric lines originate

pair 4,934 and 4,554, i.e., they are due to the ionized atom of these elements. The principal lines of the system of single lines of these elements also occur in the flash spectrum, but table 38.1 shows that they reach a much lower level.

No satisfactory series formula are known for the other high-level chromospheric elements, viz., Titanium, Scandium, Iron, and other elements. But the recent remarkable work of Kossel and Sommerfeld³ makes it quite clear that the spark-lines of these elements are due to the ionized atom. The spark-lines of alkalies have not been much investigated and lie in the ultraviolet beyond 3,000, so that, even if they are present in the high-level chromosphere, we shall have no means of detecting them.⁴

As regards Hydrogen, ionized Hydrogen would mean simply the hydrogen core, and this probably by itself would be incapable of emitting any radiation. But as

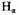
and

lines occur high in the chromosphere, we have to admit that hydrogen probably is not much ionized in the chromosphere.

The case of helium is very interesting. It is well known that the Fraunhofer spectrum does not contain any helium lines, which are obtained only in the flash spectrum. But these lines are all due to normal helium, and the highest level reached by the second line of the so-called principal series is some 8,500 kms., while the better-known D₃ reaches a level of 7,500 kms. The lines due to ionized helium are represented by the general series formula

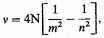
and the best known of them, in the visible range, are the Rydberg line 4,686 and the Pickering system

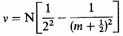
once ascribed to "cosmic hydrogen." Mitchell⁵ states that 4,686 occurs in the flash spectrum, and reaches a level of 2,000 kms. If the identification be all right, helium would present a seemingly anomalous case, for, whereas other elements are ionized in the upper strata, it is ionized in the lower strata of the chromosphere.

The above sketch embodies, in short, the problems before us. The alkaline earths and the heavier elements are ionized throughout the whole of the solar atmosphere, but the ionization is complete in the chromosphere, which seems to contain no normal atom at all. But hydrogen and helium are probably unionized throughout the whole chromosphere, and in the case of helium we have probably some slight ionization in the lower parts—a rather anomalous case.

The explanation of these problems, and some other associated problems of solar physics, will be attempted in this paper. The method is based upon a recent work of Eggert⁶—"On the State of Dissociation in the Inside of fixed Stars." In this problem, Eggert has shown that by applying Nernst’s formula of "Reaction-isobar,"

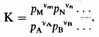
to the problems of gaseous equilibrium in the inside of stars, it is possible to substantiate many of the assumptions made by Eddington⁷ in his beautiful theory of the constitution of stars. These assumptions are that in the inside of stars the temperature is of the range of 10⁵ to 10⁶ degrees and the pressure is about 10⁷ Atm., and the atoms are so highly ionized that the mean atomic weight is not much greater than 2. This method is directly applicable to the study of the problems sketched above. The equation of the Reaction-isobar is

and the summation is extended over all the reacting substances. The present case is treated as a sort of chemical reaction, in which we have to substitute ionization for chemical decomposition. The next section shows how U is to be calculated. The equation will be resumed in §3.

2

We may regard the ionization of a calcium atom as taking place according to the following scheme, familiar in physical chemistry,

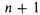
Where Ca is the normal atom of calcium (in the state of vapour), Ca₊ is an atom which has lost one electron, U is the quantity of energy liberated in the process. The quantity considered is 1 gm. atom.

The value of U in the case of alkaline earths, and many other elements, can easily be calculated from the value of the ionization potential of elements as determined by Franck and Hertz, MacLennan⁸ and others. Let

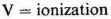
potential. Then, to detach one electron from the atomic system, we must add to each atom an amount of energy equivalent to that acquired by an electron falling through a potential difference V, where V (in volts) is given by the quantum relation,

v₀ being the convergence frequency of the principal series, i.e., (1, s) in Paschen’s notation.⁹ If this quantity be multiplied by the Avogadro number N, and expressed in calories, we obtain U.

Thus if

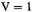
volt, we have

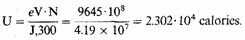
Table 38.2 contains for future use the values of the ionization potentials as far as known, and the calculated value

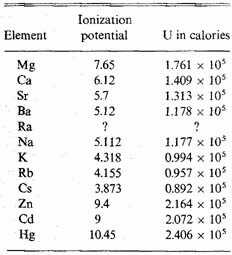Table 38.2 Values of the ionization potentials and of U (U = heat of dissociation)

of U. Here I wish to remark that an element may have more than one ionization potential, depending upon the successive transfer of the outer electrons one by one to infinity, or the simultaneous existence of two more constitutions of the normal atom (e.g. helium and parhelium). The ionization potential given in the table corresponds to the case when only one electron is transferred to infinity leaving an excess of unit positive charge in the atom. We have made it clear in the introduction that the high-level alkaline earth-lines are due to the atoms with one plus charge in excess.

The cases of hydrogen and helium will be taken up later on.

3. EQUATION OF THE REACTION-ISOBAR FOR IONIZATION

As mentioned in the introduction, the equation of gaseous equilibrium proceeds according to the equation,

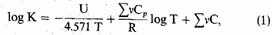
where the reaction proceeds according to the scheme,

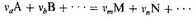
and K is the "Reaction-isobar,"

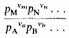
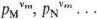
being the partial pressures of the reacting substances—M, N, etc.

In the present cases, viz., for a reaction of the type,

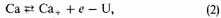
we have

We can take

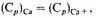
and

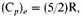
the electron being supposed to behave like a monatomic gas.

Eggert calculates the chemical constant from the Sackur-Tetrode-Stern relation,

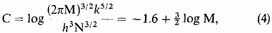
where

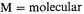
weight, the pressure being expressed in atmospheres.

Now C has the same value for Ca and Ca₊. For the electron

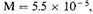
and

We have thus

To calculate the "Reaction-isobar" K, let us assume that P is the total pressure, and a fraction x of the Ca-atoms is ionized.

Then we have

This is the equation of the "reaction-isobar" which is throughout employed for calculating the "electron-affinity" of the ionized atom.

IONIZATION OF CALCIUM, BARIUM, AND STRONTIUM

With the aid of formula (1), the degree of ionization for any element, under any temperature and pressure, can be calculated when the ionization potential is known. As a concrete example, we may begin with Calcium, Strontium, and Barium.

Saha notes that pressure has a great influence on the degree of ionization and presents tables showing the percentages of ionization of calcium, strontium, and barium under varying conditions of pressure and temperature. As an example, an extract from the table for calcium is given below. Saha then assumes that the temperature of the photosphere and the reversing layer are, respectively, 7,500 K and 6,000 K. The partial pressure in the reversing layer is supposed to vary from 10 atm in the reversing layer to 10^-12 atm in its outermost layers.

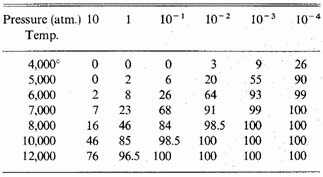Table 38.3 Percentage ionization of calcium

An examination of tables IV, V, VI, [not reproduced] shows that, under the above-mentioned assumptions, about 34 per cent. of the Ca-atoms are ionized on the photosphere. When the pressure falls to 10^-4 atmosphere, almost all the atoms get ionized, so that up to this point in the solar atmosphere, we shall get combined emission of the H, K, and the g-line, but above this point, we shall have only the H, K lines. This is in very good agreement with observed facts.

In the case of strontium and barium, owing to their comparatively low ionization potential, ionization at 6,000° is practically complete at 10^-3 atmosphere, and the heights shown by the lines of the unionized atoms of these elements are still lower.

The results of the flash-spectrum observations are thus seen to be very satisfactorily accounted for on the basis of our theory.

Laboratory experiments also, as far as they go, are in qualitative agreement with our theory. It is well known that in the flame, the [lines] due to the ionized atom either do not occur at all, or even if they do occur they are extremely faint compared with the lines of the unionized atom. As the temperature is increased, the "enhanced lines" begin to strengthen, until at the temperature of the arc they are comparable in intensity to the lines of the normal atom.

Here Saha presents the intensity of enhanced and ordinary lines in furnace spectra as a function of temperature¹⁰ and compares these lines with the photosphere lines, with the chromosphere lines, and with his theory.

The tables show that an increase of temperature causes an increase of ionization and the proportion of emission centres of the enhanced lines. The increasing intensities of the double lines are mainly to be ascribed to this fact. These become comparable in intensity to the principal lines of the normal atom only when the degree of ionization is rather large. Comparing the relative intensities of the corresponding lines of the calcium and barium group, we find that for the same temperature the enhanced lines of barium are relatively stronger than the calcium lines; and this, according to our theory, is due to the comparatively lower ionization potential of barium.

4. HYDROGEN IN THE SUN

Saha notes that the hydrogen in the sun has not been detected in ionized or molecular form. The dissociation of the hydrogen molecule into atoms is shown to be complete in the conditions prevailing in the sun. Consideration of the ionization of hydrogen shows that, at a temperature of 6,000 K, hydrogen can be completely ionized at the pressure of 10^-11 atm. Thus, only at the highest point of the chromosphere, where the partial pressure falls to 10^-11 atm, can the ionization be complete and the vanishing of the atomic hydrogen lines be expected. Helium is then shown to be unionized under conditions in the solar atmosphere, because of its high ionization potential.

SUMMARY

1. In the present paper it has been shown from a discussion of the high-level chromospheric spectrum that this region is chiefly composed of ionized atoms of Calcium, Barium, Strontium, Scandium, Titanium, and Iron. In the lower layers both ionized and neutral atoms occur.

2. An attempt has been made to account for these facts from the standpoint of Nernst’s theorem of the "Reaction-isobar," by assuming that the ionization is a sort of reversible chemical process taking place according to the equation

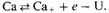
The energy of ionization U can be calculated from the ionization-potential of elements as determined by Franck and Hertz, and MacLennan. For determining Nernst’s chemical constant and the specific heat, the electron has been assumed to be a monatomic gas having the atomic weight of 1/1836.

3. The equation shows the great influence of pressure on the relative degree of ionization attained. The almost complete ionization of Ca, Sr, and Ba atoms in the high-level chromosphere is due to the low pressure in these regions. The calculated values are in very good accord with observational data and the laboratory experiments of King.

4. Hydrogen has been shown to be completely dissociated into atoms at all points in the solar atmosphere.

5. It has also been shown that the greater the ionization potential of an element, the more difficult ionization will be for that element under a given thermal stimulus. Calculations have been made in the case of hydrogen (

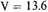
volts) and helium (

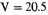
volts), which show that these elements cannot get ionized anywhere in the Sun to an appreciable extent. Helium can have appreciable ionization only in stars having the highest temperature (>16,000° K.), which only are therefore capable of showing the Rydberg line 4,686 and the Pickering lines

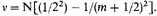
1. J. Fraunhofer, Denkschriften der königlichen Akademie der Wissenschaften zu München 5, 193 (1817); trans. by J. S. Ames in Prismatic and Diffraction Spectra (New York: Harper, 1898) and reproduced by H. Shapley and H. E. Howarth in A Source Book in Astronomy (New York: McGraw-Hill, 1929); G. R. Kirchhoff, Monatsberichte der königlichen Preussischen Akademie der Wissenschaften zu Berlin, 662 (1859). Also see Philosophical Magazine 19, 193 (1860), 21, 185, 241 (1861), 22, 329, 498 (1861).

2. J. N. Lockyer, The Chemistry of the Sun (London: Macmillan and Co., 1887).

3. R. H. Fowler and E. A. Milne, Monthly Notices of the Royal Astronomical Society 83, 403 (1923), 84, 499 (1924).

4. M. Saha, Proceedings of the Royal Society (London) 99A, 135 (1921). Also see E. A. Milne, Observatory 44, 261 (1921).

1. Mitchell, Astrophysical Journal 38, 424 (1913).

2. Fowler, Phil. Trans. 214, 225 (1914).

3. Kossel and Sommerfeld, Ber, d. d. Phys. Gesellschaft, Jahrgang 21, 240.

4. Ibid., p. 250.

5. Mitchell, pp. 490–491.

6. Eggert, Phys. Zeitschrift 20, 570 (1919).

7. Eddington, M.N.R.A.S. 77, 16, 596.

8. McLennan, Proceedings of the Physical Society of London, 31, 1, 30 (1918).

9. Paschen uses the symbol (1.5, s), but following Sommerfeld (p. 243), I have taken off .5 and used (1, s).

10. King, Astrophysical Journal 48, 13 (1918).

Contents:

Related Resources

None available for this document.

Download Options

Title: A Source Book in Astronomy and Astrophysics, 1900-1975

Select an option:

*Note: A download may not start for up to 60 seconds.

Email Options

Title: A Source Book in Astronomy and Astrophysics, 1900-1975

Select an option:

Email addres:

*Note: It may take up to 60 seconds for for the email to be generated.

Chicago: Meghnad Saha, "Ionization in the Solar Chromosphere," A Source Book in Astronomy and Astrophysics, 1900-1975 in A Source Book in Astronomy and Astrophysics, 1900-1975, ed. Kenneth R. Lang and Owen Gingerich (Cambridge: Harvard University Press, 1979), 236–242. Original Sources, accessed July 3, 2025, http://www.originalsources.com/Document.aspx?DocID=97BJM2U3S44WW6L.

MLA: Saha, Meghnad. "Ionization in the Solar Chromosphere." A Source Book in Astronomy and Astrophysics, 1900-1975, Vol. 40, in A Source Book in Astronomy and Astrophysics, 1900-1975, edited by Kenneth R. Lang and Owen Gingerich, Cambridge, Harvard University Press, 1979, pp. 236–242. Original Sources. 3 Jul. 2025. http://www.originalsources.com/Document.aspx?DocID=97BJM2U3S44WW6L.

Harvard: Saha, M, 'Ionization in the Solar Chromosphere' in A Source Book in Astronomy and Astrophysics, 1900-1975. cited in 1979, A Source Book in Astronomy and Astrophysics, 1900-1975, ed. , Harvard University Press, Cambridge, pp.236–242. Original Sources, retrieved 3 July 2025, from http://www.originalsources.com/Document.aspx?DocID=97BJM2U3S44WW6L.