Electricity a Wave in the Ether

James Clerk Maxwell

In several parts of this treatise an attempt has been made to explain electromagnetic phenomena by means of mechanical action transmitted from one body to another by means of a medium occupying the space between them. The undulatory theory of light also assumes the existence of a medium. We have now to show that the properties of the electromagnetic medium are identical with those of the luminiferous medium.

To fill all space with a new medium whenever any new phenomenon is to be explained is by no means philosophical, but if the study of two different branches of science has independently suggested the idea of a medium, and if the properties which must be attributed to the medium in order to account for electromagnetic phenomena are of the same kind as those which we attribute to the luminiferous medium in order to account for the phenomena of light, the evidence for the physical existence of the medium will be considerably strengthened.

But the properties of bodies are capable of quantitative measurement. We therefore obtain the numerical value of some property of the medium, such as the velocity with which a disturbance is propagated through it, which can be calculated from electromagnetic experiments, and also observed directly in the case of light. If it should be found that the velocity of propagation of electromagnetic disturbances is the same as the velocity of light, and this not only in air, but in other transparent media, we shall have strong reasons for believing that light is an electromagnetic phenomenon, and the combination of the optical with the electrical evidence will produce a conviction of the reality of the medium similar to that which we obtain, in the case of other kinds of matter, from the combined evidence of the senses.

When light is emitted, a certain amount of energy is expended by the luminous body, and if the light is absorbed by another body, this body becomes heated, showing that it has received energy from without. During the interval of time after the light left the first body and before it reached the second, it must have existed as energy in the intervening space.

According to the theory of emission, the transmission of energy is effected by the actual transference of light-corpuscles from the luminous to the illuminated body, carrying with them their kinetic energy, together with any other kind of energy of which they may be the receptacles.

According to the theory of undulation, there is a material medium which fills the space between the two bodies, and it is by the action of contiguous parts of this medium that the energy is passed on, from one portion to the next, until it reaches the illuminated body.

The luminiferous medium is therefore, during the passage of light through it, a receptacle of energy. In the undulatory theory, as developed by Huygens, Fresnel, Young, Green, etc., this energy is supposed to be partly potential and partly kinetic. The potential energy is supposed to be due to the distortion of the elementary portions of the medium. We must therefore regard the medium as elastic. The kinetic energy is supposed to be due to the vibratory motion of the medium. We must therefore regard the medium as having a finite density.

In the theory of electricity and magnetism adopted in this treatise, two forms of energy are recognised, the electrostatic and the electrokinetic, and these are supposed to have their seat, not merely in the electrified or magnetized bodies, but in every part of the surrounding space, where electric or magnetic force is observed to act. Hence our theory agrees with the undulatory theory in assuming the existence of a medium which is capable of becoming a receptacle of two forms of energy.

Let us next determine the conditions of the propagation of an electromagnetic disturbance through a uniform medium, which we shall suppose to be at rest, that is, to have no motion except that which may be involved in electromagnetic disturbances.

Let C be the specific conductivity of the medium, K its specific capacity for electrostatic induction, and u its magnetic "permeability." [p.211]

The quantity V, in Art. 784, which expresses the velocity of propagation of electromagnetic disturbances in a non-conducting medium is, by equation (10), equal to

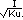
If the medium is air, and if we adopt the electrostatic system of measurement, K=1 and u=1/v2, so that V=v, or the velocity of propagation is numerically equal to the number of electrostatic units of electricity in one electromagnetic unit. If we adopt the electromagnetic system, K=1/v2 and u=1, so that the equation V=v is still true.

On the theory that light is an electromagnetic disturbance, propagated in the same medium through which other electromagnetic actions are transmitted, V must be the velocity of light, a quantity the value of which has been estimated by several methods. On the other hand, v is the number of electrostatic units of electricity in one electromagnetic unit, and the methods of determining this quantity have been described in the last chapter. [Here inserted.]

Comparison of Units of Electricity

[Since the ratio of the electromagnetic to the electrostatic unit of electricity is represented by a velocity, we shall in future denote it by the symbol v. The first numerical determination of this velocity was made by Weber and Kohlrausch.

Their method was rounded on the measurement of the same quantity of electricity, first in electrostatic and then in electromagnetic measure.

The quantity of electricity measured was the charge of a Leyden jar. It was measured in electrostatic measure as the product of the capacity of the jar into the difference of potential of its coatings. The capacity of the jar was determined by comparison with that of a sphere suspended in an open space at a distance from other bodies. The capacity of such a sphere is expressed in electrostatic measure by its radius. Thus the capacity of the jar may be found and expressed as a certain length. See Art. 227.

The difference of the potentials of the coatings of the jar was measured by connecting the coatings with the electrodes of an electrometer, [p.212] the constants of which were carefully determined, so that the difference of the potentials, E, became known in electrostatic measure.

By multiplying this by c, the capacity of the jar, the charge of the jar was expressed in electrostatic measure.

To determine the value of the charge in electromagnetic measure, the jar was discharged through the coil of a galvanometer. The effect of the transient current on the magnet of the galvanometer communicated to the magnet a certain angular velocity. The magnet then swung round to a certain deviation, at which its velocity was entirely destroyed by the opposing action of the earth’s magnetism.

By observing the extreme deviation of the magnet the quantity of electricity in the discharge may be determined in electromagnetic measure, as in Art. 748, by the formula

where Q is the quantity of electricity in electromagnetic measure. We have therefore to determine the following quantities:

H, the intensity of the horizontal component of terrestrial magnetism; see Art. 456.
G, the principal constant of the galvanometer; see Art. 700.
T, the time of a single vibration of the magnet; and
O, the deviation due to the transient current.

The value of v obtained by MM. Weber and Kohlrausch was

  v=310740000 metres per second.

The property of solid dielectrics, to which the name of Electric Absorption has been given, renders it difficult to estimate correctly the capacity of a Leyden jar. The apparent capacity varies according to the time which elapses between the charging or discharging of the jar and the measurement of the potential, and the longer the time the greater is the value obtained for the capacity of the jar.

Hence, since the time occupied in obtaining a reading of the electrometer is large in comparison with the time during which the discharge through the galvanometer takes place, it is probable that the estimate of the discharge in electrostatic measure is too high, and the value of v, derived from it, is probably also too high.]

They are quite independent of the methods of finding the velocity of light. Hence the agreement or disagreement of the values of V and of v furnishes a test of the electromagnetic theory of light. [p.213]

In the following table, the principal results of direct observation of the velocity of light, either through the air or through the planetary spaces, are compared with the principal results of the comparison of the electric units:—

Fizeau	314000000
Aberration, etc., and Sun’s Parallax	308000000
Foucault	298360000

Weber	310740000
Maxwell	288000000
Thomson	282000000

It is manifest that the velocity of light and the ratio of the units are quantities of the same order of magnitude. Neither of them can be said to be determined as yet with such a degree of accuracy as to enable us to assert that the one is greater or less than the other. It is to be hoped that, by further experiment, the relation between the magnitudes of the two quantities may be more accurately determined.

In the meantime our theory, which asserts that these two quantities are equal, and assigns a physical reason for this equality, is certainly not contradicted by the comparisons of these results such as they are.

1856 Weber and Kohlrausch	3.107X1010 (cm. per second)
1868 Maxwell	2.842X1010
1869 W. Thomson and King	2.808X1010
1872 McKichan	2.896X1010
1879 Ayrton and Perry	2.960X1010
1880 Shida	2.955X1010
1883 J.J. Thomson	2.963X1010
1884 Klemencic	3.019X1010
1888 Himstedt	3.009X1010
1889 W. Thomson	3.004X1010
1889 E.B. Rosa	2.9993X1010
1890 J. J. Thomson and Searle	2.9955X1010

Cornu (1878)	3.003 x 1010
Michelson (1879)	2.9982 x 1010
Michelson (1882)	2.9976 x 1010
Newcomb (1885)	2.99615
	2.99682 x 1010
	2.99766

In other media than air, the velocity V is inversely proportional to the square root of the product of the dielectric and the magnetic inductive capacities. According to the undulatory theory, the velocity of light in different media is inversely proportional to their indices of refraction.

There are no transparent media for which the magnetic capacity differs from that of air more than by a very small fraction. Hence the principal hart of the difference between these media must depend on their dielectric capacity. According to our theory, therefore, the dielectric capacity of a transparent medium should be equal to the square of its index of refraction.

But the value of the index of refraction is different for light of different kinds, being greater for light of more rapid vibrations. We must therefore select the index of refraction which corresponds to waves of the longest periods, because these are the only waves whose motion can be compared with the slow processes by which we determine the capacity of the dielectric.

The only dielectric of which the capacity has been hitherto determined with sufficient accuracy is paraffin, for which in the solid form MM. Gibson and Barclay found.

     K=1.975.

Dr. Gladstone has found the following values of the index of refraction of melted paraffin, sp. g. 0.779, for the lines A, D and H:—

Temperature	A	D	H
54°C	1.4306	1.4357	1.4499
57°C	1.4294	1.4343	1.4493

from which I find that the index of refraction for waves of infinite length
would be about          1.422.
The square root of K is      1.405.
The difference between these numbers is greater than can be accounted for by errors of observation, and shows that our theories of the structure of bodies must be much improved before we can deduce their optical from their electrical properties. At the same time, I think that the agreement of the numbers is such that if no greater discrepancy were found between the numbers derived from the optical and the electrical properties of a considerable number of substances, we should be warranted in concluding that the square root of K, though it may not be the complete expression for the index of refraction, is at least the most important term in it.