Mathematical Representations of Motion

1. Thomas Bradwardine: "Bradwardine’s Function" and the Repudiation of Four Opposition Theories on Proportions of Motion

Translated by H. Lamar Crosby, Jr.¹

Annotated by Edward Grant

CHAPTER 2

Having looked into these introductory matters,² let us now proceed with the undertaking which was proposed at the outset. And first, after the manner of Aristotle, let us criticize erroneous theories, so that the truth may be the more apparent.

There are four false theories to be proposed as relevant to our investigation, the first of which holds that: the proportion between the speeds with which motions take place varies as the difference whereby the power of the mover exceeds the resistance offered by the thing moved.³

This theory claims in its favor that passage from Book I of the De caelo et mundo (in the chapter on the "infinite") in the text which reads:

"It is necessary that proportionally as the mover is in excess, etc.," together with Averroes’ Comment⁴ 71 on Book IV of the Physics, in which he says: "Every motion takes place in accordance with the excess of the power of the mover over that of the thing moved." In Comment 35, on Book VII of the Physics, he further states that: "The speed proper to any given motion varies with the excess of the power of the mover over that of the thing moved," and in the final Comment, Comment 39, he says that: "The speed of alteration and the quantity of time will vary in accordance with the amount whereby the power of that which is causing the alteration exceeds the resistance offered by what undergoes the alteration." Many other passages afford similar remarks.

The present theory may, however, be torn down in several ways:

First, according to this theory, it would follow that, if a given mover moved a given mobile through a given distance in a given time, half of that mover would not move half of the mobile through the same distance in an equal time, but only through half the distance. The consequence is clear, because, if the whole mover exceeds the whole mobile by the whole excess, then half the mover exceeds half the mobile by only half the former amount; for, just as 4 exceeds 2 by 2, half of 4 (namely, 2) exceeds half of itself (that is, 1) by 1, which is only half of the former excess.

That such a consequence is false is apparent from the fact that Aristotle proves, at the close of Book VII of the Physics, that: "If a given power moves a given mobile through a given distance in a given time, half that power will move half the mobile through an equal distance in an equal time" [250a.4–6—Ed.]. Aristotle’s reasoning is quite sound, for, since the half is related to the half by the same proportion as the whole is to the whole, the two motions will, therefore, be of equal speed.

Secondly, it follows from this theory that, given two movers moving two mobilia through equal distances in equal times, the two movers, conjoined, would not move the two mobilia, conjoined, through an exactly equal distance in an equal time, but, instead, through double that distance. This consequence follows necessarily because the excess of the two movers, taken together, over the two mobilia, taken together, is twice the excess of each of them over its own mobile; for, just as anything having a value of 2 exceeds unity by 1, so two such "2’s" (which make 4) exceed two "1’s" (which make 2) by 2, which is twice the excess of 2 over 1. The foregoing holds in all cases in which two subtrahends are equally exceeded by two minuends.

That the above consequence is false is evident from the foregoing argument of Aristotle, in Book VII of his Physics, where he demonstrates the following conclusion: "If two powers move two mobilia, separately, through equal distances in equal times, those powers conjoined, will move the two mobilia conjoined, through an equal distance in a time equal to the former one" [250a.25–28—Ed.]. This argument of Aristotle is sufficient proof that the relation between a single mover and its mobile, and a compound mover and its mobile, is a proportional one.

In the third place, it would follow that a geometric proportion (that is, a similarity of proportions) of movers to their mobilia would not produce equal speeds, since it does not represent an equality of excesses; for, although the proportions of 2 to 1 and 6 to 3 are the same, the excess of the one term over the other is 1 in the first case and 3 in the second case.

The consequence to which we are thus led is, however, false and opposed to Aristotle’s opinion, as expressed at the close of Book VII of the Physics and in many other places, where, from an equality of proportions of movers to their mobilia, he always argues equal speeds. Averroes supports the same view in his remarks on the passages just mentioned and also in his Comment 71 on Physics IV, Comment 63 on De caelo et mundo I, and in many other places.

Nor can it be legitimately maintained that, in the passages cited, Aristotle and Averroes understand, by the words "proportion" and "analogy," arithmetic proportionality (that is, equality of differences), as some have claimed. Indeed, in Book VII of the Physics, Aristotle proves this conclusion: "If a given power moves a given mobile through a given distance in a given time, half that power will move half the mobile through an equal distance in an equal time, because, ’analogically,’ the relation of half the mover to half the mobile is similar to that of the whole mover to the whole mobile."⁵

Such a statement, interpreted as referring to arithmetic proportionality, is discernibly false (as has already been made sufficiently clear in the first argument raised against the present theory). Moreover, regarding this same passage, Averroes says that the proportion will be the same "in the sense that geometricians universally employ in demonstrations."

The above thesis of Aristotle may be demonstrated geometrically as follows: As is the whole mover to half the mover, so is the whole mobile to half the mobile. Therefore, permutatively (by Axiom 7 of Chapter I):⁶ As is the whole mover to the whole mobile, so is half the mover to half the mobile. And this is what was to have been proved.

The reading of Aristotle proposed by the present theory does not stand up, moreover, because, in Book VII of the Physics, Aristotle also proves this conclusion: "If two movers, separately, move two mobilia through equal distances in equal times, those two movers, conjointly, will move those two mobilia, conjoined, through a distance and in a time equal to the former,"⁷ if the medium through which the motions take place remains the same, "for the motions are ’analogous’." By "analogous" he means a proportional, but not in the sense of arithmetic proportionality, for the simple mover does not exceed the simple mobile by the same amount that the compound mover exceeds the compound mobile, (as was made clear in the second argument against this theory).

Averroes, commenting on this passage, proves that, "although the proportion will be the same, the excess will not." He does this by employing Proposition [Crosby has "Theorem"—Ed.] I of Book V of Euclid’s Elements, which states that: "If the members of a given set of quantities are either equal multiples of, equally greater or less than,⁸ or exact equals of the members of a corresponding set, it follows that the relations between corresponding individual terms of the two sets will be the same as the aggregate relation of the two sets." Therefore, the above-mentioned reading of Aristotle cannot be taken as valid.

The fourth criticism is as follows: It would follow, on the basis of the present theory, that a mixed body, possessing internal resistance, could move faster through a medium than through a vacuum, Let A, for example, be a heavy mixed body (possessing within itself both motive and resistive power)⁹ and imagine it to descend of itself through some medium, B. Let C represent a quantity of pure earth, possessing less power than the excess of motive power over resistance in A. A will, of itself, move at a determinate speed in a vacuum. Now let the medium, B, be rarified to the point at which C moves in it with a speed equal to that of A in the vacuum. If A is now placed in the same medium with C, it should move faster than C (for it possesses a greater excess of motive power over resistance). C will move in that medium with a speed equal to that of A, moving in the vacuum. Therefore A will move faster through the medium than through the vacuum.¹⁰

That it is, in fact, possible to rarify B to a point at which C would move in it with the speed just specified is evidently true, for, by rarefaction of the medium, local motion can be accelerated to any desired degree. This is shown to be the case in Book IV of the Physics (in the chapter on the "void" [215b.1–11—Ed.]), where it is stated that, with the moving power remaining constant, it is possible to arrive at any given speed of local motion by rarefying the medium.

Thus (positing that a local motion could take place in a void), we find that the same body could move at the same velocity in both a medium and a vacuum.¹¹

Our fifth criticism is that it would also follow that, if a given mover exceeded its resistance by a lesser amount than another mover exceeded its resistance, the former motion would be the slower one. Let a large quantity of earth, possessing a given resistance which its downward force greatly exceeds, be supposed to fall. Let also another quantity of earth, possessing a lesser such excess of power, be supposed to fall. Letting the larger quantity of earth and the resistance associated with it remain constant, let the medium in which the smaller quantity of earth moves now be rarefied to the point at which its speed becomes equal to that of the larger quantity. The smaller quantity now moves its resistance with the same speed that the larger moves its own, and yet exceeds it by a smaller amount.¹²

Sixthly, it would also follow that, if a bit of pure earth were moving in some medium whose resistance its power exceeded by a ratio of two to one, or more, it could not move at double that speed in any other medium. It could not exceed any medium by double the first excess, for, in that case, the entire moving power would be excess, and, with the moving power remaining constant, it would consequently not be possible to increase the speed of the motion indefinitely by rarefaction of the medium. Such a consequence has already been established as false.¹³

In the seventh place, another consequence would be that, if a given mover were to exceed its resistance by a greater amount than another mover exceeded its own resistance, the former motion would be the faster. Then, since a strong man exceeds anything he moves by a greater excess of power than a weaker mover (such as a boy, or a fly, or something of that sort) exceeds what it moves, he should move it more rapidly.

Experience, however, teaches us the contrary, for we see that a fly carrying some small particle flies very rapidly, and that a boy also moves a small object rather rapidly. A strong man, on the other hand, moving some large object which he can scarcely budge, moves it very slowly, and even if there were added to what he moves a quantity larger than either the fly or the boy can move, the man will then move the whole not much more slowly than he did before.

From all these considerations, therefore, the following negative conclusion is sufficiently well established:

The proportion of speeds in motions does not vary with the amount whereby the power of the mover exceeds that of the thing moved.

Objections to this conclusion are not difficult to dissolve, for Aristotle and Averroes, when they say that the speed of a motion varies in accordance with the amount by which the power of the mover excels or exceeds that of the thing moved, understand by "excellence," or "excess," a proportion of greater inequality whereby the power of the mover excels, or exceeds, the power of the thing moved.¹⁴

CHAPTER 2, PART 2

[THEORY II]

Let us now turn to the second erroneous theory, which supposes the proportion of the speeds of motions to vary in accordance with the proportion of the excesses whereby the moving powers exceed the resisting powers.¹⁵ This idea is evidently based on Averroes’ Comment 36 on Book VII of the Physics, for he there states that the speed of a motion is determined by the proportion whereby the power of the mover exceeds that of the thing moved.

This theory should, however, be refuted as false. For just imagine the case in which the excess of the power of the mover over that of the thing moved is equal to the power of the thing moved. No mover will be able to move any mobile either faster or slower than the speed produced by this proportion, because no other proportion can be either greater or smaller than that whereby the excess of power of this mover over its mobile is related to the power of the mobile as a whole (as is demonstrable by Theorem VII, Chapter I).¹⁶

In the second place, a moving power moves a whole mobile primarily by means of its total strength, and not by means of a residuum of its strength. A motion and its speed vary primarily and essentially, therefore, with the relation, or proportion, between the entire power of the mover and that of the thing moved, and not (except accidentally and secondarily) according to a proportion of excess.

This negative conclusion is therefore evident: The proportion of the speeds of motions does not vary in accordance with the proportion¹⁷of the excess of the motive power over the power of the thing moved. The above-mentioned statement by Averroes may, if anyone were so to desire, be interpreted in the same way as were the other authorities cited in support of Theory I.

CHAPTER 2, PART 3

[THEORY III]

There follows the third erroneous theory, which claims that: (with the moving power remaining constant) the proportion of the speeds of motions varies in accordance with the proportion of resistances,¹⁸and (with the resistance remaining constant) that it varies in accordance with the proportion of moving powers.¹⁹

With respect to its first part, this theory is seen to be founded on many passages of Aristotle’s writings. In Book IV of the Physics (in the chapter on the "void") he speaks as follows: "Let B represent a given quantity of water and D a given quantity of air. Now, by however much air is thinner and more incorporeal than water, by so much will A (that is, the moving body) move faster through D than through B. Let the one speed bear the same ratio, or proportion, to the other as that whereby air differs from water, and then, if air is twice as thin, the body will traverse B in twice the time required to traverse D" [215b.4–8—Ed.]. Furthermore, the text immediately following manifestly makes the supposition that, with the moving power remaining constant and the medium being varied, the proportion of the speeds of motions varies in accordance with the proportion of media, and that, conversely, the proportion of the times measuring those motions varies also in accordance with the proportion of media (namely, that the longer time corresponds to the motion through the denser medium and the shorter time to the motion through the rarer medium).

Further, in Book I of the De caelo (in the chapter on the "infinite") he speaks as follows: "It is held to undergo a greater and tess effect by the action of the same agent, in a longer and shorter time, any such effects are divided proportionally to the time" [275a.32—275b.2—Ed.].

And, at the end of Book VII of the Physics, Aristotle wishes it to be understood that, if a given power moves a given mobile through a given distance in a given time, the same power will move half the same mobile through twice the distance in an equal time, and through the same distance in half the time [249b.30—250a.2—Ed.].

Thus much in favor of the first half of the present theory.

In support of the second part of this position, Aristotle holds, in Book IV of the Physics (in the chapter concerning the "void") that, other conditions remaining constant, heavy and light bodies differing in quantity will move through a given distance in the same medium more swiftly and more slowly in accordance with the proportion of the heavy and light bodies to each other [216a.14–16—Ed.].

According to Averroes’ exposition, at the close of Book VII of the Physics, Aristotle intends that, if a given power moves a given mobile through a given distance in a given time, double the power will move that mobile through double the distance in an equal time.

At the close of Book VIII of the Physics, Aristotle maintains that a motive power which is double another such power will move the same mobile in half the time required by the lesser power [266b.10–11—Ed.], and that, universally, a motive power greater than another will move the same mobile which the smaller power moves in a time that is less by converse proportion (that is, that less time is required by the larger power and more time by the lesser power) [266b.17–19—Ed.].

Moreover, Aristotle intends the same thing in Book I of the De caleo (in the chapter on the "infinite"), where, speaking of heavy bodies that fall equal distances in the same medium, he writes as follows: "The ’analogy’ (that is, the proportion) between the weights will be the contrary of that between the times. For example, if the whole weight in a given time, then double the weight in half that time" [273b.31—274a.2—Ed.].

Further, in Book III of the De caelo, where Aristotle proves every body to possess a rectilinear gravity or levity, he states that heavy bodies unequal in power will traverse, in the same medium and the same time, distances proportional to those powers [perhaps 301b.11–15—Ed.].

The same is evident from Theorem I of the De ponderibus, which states the following: "The proportion between the speeds of descent of any given heavy bodies is the same as that between their respective weights."²⁰

The theory may also be set forth by the following reasoning: If one mover has exactly twice the power possessed by another, it can move the same mobile exactly twice as much, or move twice the mobile the same amount, for if it is exactly twice the power, it can accomplish exactly twice as much. If it could accomplish more than twice as much, it would be of more than twice the power; and if it were not capable of twice, but only of less, it would be of less than twice the power.

So much for the second part of this position, and thus we have seen what are the foundations of both parts of the theory.

The theory is, however, refutable on two grounds: first, on that of insufficiency, second, because it yields false consequences.

It is insufficient, because it does not determine the proportion of the speeds of motions except in cases where either the mover or the mobile are constant. Concerning motions in which the moving forces, as well as the mobilia, are varied, it tells us almost nothing.

The theory is, on the other hand, to be refuted on the ground of falsity, for the reason that a given motive power can move a given mobile with a given degree of slowness and can also cause a motion of twice that slowness. According to this theory, therefore, it can move double the mobile. And, since it can move with four times the slowness, it can move four times the mobile, and so on ad infinitum. Therefore, any motive power would be of infinite capacity.²¹

A similar argument may be made from the standpoint of the mobile. For any mobile may be moved with a given degree of slowness, with twice that degree, four times, and so on without end; and, therefore, by the given mover, and by half of it, one fourth of it, and so on, without end. Any mobile could, therefore, be moved by any mover.²²

Nor is it legitimate to object that slowness of motion cannot be doubled indefinitely, for, supposing this to be true, let A represent some slowness of a mobile that cannot be doubled. Now imagine a sphere or cylinder, revolving about a fixed axis; then, at some point near the pole of the sphere, or the axis of the cylinder, there is a degree of slowness double that of A, as is quite clear and easy to demonstrate. Now, at this point let there be attached a strong, long cord, at whose end is affixed a given weight, B. Then the slowness of the motion of B is twice that of A, and this is what we wished to demonstrate.

Nor can some quibbler properly claim that the motion of B is accidental motion, merely motion in potentia, and that it really has no relevance to the question; for this motion possesses a mover, a thing moved, initial and final limits, a time and a space traversed. . . all in actu. Therefore, the motion is a real one. Nor can it be maintained that the mover is not in actu, but only in potentia, inasmuch as it is part of the sphere, or cylinder, and because it is the whole that moves primarily and the part by consequence. In that case, if a man were to pull that weight by hand, by means of the cord, it would move accidentally, because by virtue of a part of the man; it would then follow that no motion, extrinsically caused, could be a "real" motion, (one which is in actu), since no mover can apply itself wholly to the thing moved, but can only do so by means of a part.

Thirdly, the present theory is to be refuted on the ground of falsity, because sense experience teaches us the opposite. We see, indeed, that if, to a single man who is moving some weight which he can scarcely manage with a very slow motion, a second man joins himself, the two together can move it much more than twice as fast.²³ The same principle is quite manifest in the case of a weight suspended from a revolving axle, which it moves insensibly during the course of its own insensible downward movement (as is the case with clocks). If an equal clock weight is added to the first, the whole descends and the axle, or wheel, turns much more than twice as rapidly (as is sufficiently evident to sight).

Since the situation regarding retardation is closely similar, whether the thing moved be constant and the mover be diminished or the reverse we arrive at this negative conclusion: With the mover remaining constant, the proportion of the speeds of motions does not vary in accordance with the proportion of resistances, nor, with the resistance remaining constant, does it vary in accordance with the proportion of movers.

As for the reasons which seemed to support this theory, it should be pointed out that all authorities claiming that, with the mover remaining constant, the proportion of the speeds of motions varies in accordance with the proportion of resistances, really mean that the proportion of speeds varies with the proportion of the things affected to the things affecting them.²⁴

As a matter of fact, in the case of the first authority cited (that of Book IV of the Physics) it should be realized that what Aristotle means is that, to whatever extent the proportion of air to a given body which moves through it is smaller than that of water to that same body (due to the greater thinness and incorporeality of air), to that extent the body wilt move faster through air than through water; for to whatever extent the proportion of air to a given body is smaller than the proportion of water to that body, to the same extent is the proportion of the body to air larger than its proportion to water, and, as will later be shown, the proportion of the speeds of motions varies in accordance with the proportion of movers to things moved.

The second authority cited should also be construed in the same sense, together with that passage, from Book I of the De caelo et mundo, which reads: "It is held to undergo a greater and less effect, by the action of the same agent, in a longer and shorter time, and any such effects are divided proportionally to the time." In other words, the proportions of any given effects are divided proportionally to the time.

In the case, moreover, of the theorem drawn from Book VII of the Physics (which states that, if a given power moves a given mobile through a given distance in a given time, the same power will move half of it through double the distance in an equal time), Aristotle understands, by "half the mobile," a part of the mobile possessing a proportional relation to the given moving power which is half that of the whole mobile to that power.

This quite clearly appears to be Averroes’ interpretation, for, regarding the passage in question, he proves the above-mentioned theorem as follows: "When we divide the motion (or the thing moved), it follows necessarily that the proportion of the power of the mover to the motion (or thing moved) is twice the former proportion. This would, nevertheless, be untrue, unless understood in the sense previously indicated, for, although a given whole may bear a given proportion to some other whole, it does not follow that it bears to half of the latter whole a proportion double the first proportion [after "bears" Crosby has "a proportion to half of the latter whole that is half the original proportion"—Ed.] (as will be shown in what follows)." It is in this sense that the following authorities should be interpreted.

The theorem drawn from the De ponderibus must be read in the same way. "Between whatever heavy bodies, etc. . . .," that is, between whatever heavy bodies the proportion of speed of descent and that of the proportion of weight to resistance are taken in the same order. (And with the proviso that the resistance remains equal.)

The author of this work, however, proposes no principle in proof of this theorem, and it may well be objected against the above interpretation, that neither does any commentator prove the theorem in this sense, but rather in another, which the present theory supports (namely, that there is neither any proportion nor excess of motive power over resistive power) and which is, in fact, what the words of this theorem really mean.

The fact is that no commentator whom we have seen either proves this theorem according to our interpretation, or according to any other. One commentator, for example, takes two unequal weights and two unequal lines representing their descents, and then, first taking it as granted by his adversary that the proportion of the larger weight to the smaller is greater than the proportion of the longer line to the shorter, he argues from this that the proportion of the smaller weight to the larger is less than the proportion of the shorter line to the longer. From this he concludes that the proportion of weights is less than that of descents (the opposite of which had been stipulated).

This however, presents no obstacle, for it was admitted that the proportion between the weights was greater than that between the descents, in the first place, and it not only does not invalidate the theorem, but it follows (conversely) that the proportion of the smaller weight to the larger will be smaller than that of the shorter descent, or line, to the longer.²⁵

Another commentator, also taking two unequal weights and their unequal descents, adds to the smaller weight a second, such that the two together are equal to the larger. He now posits that the descent of the added weight, by itself, through a time equal to that of the previous descents, when added to the descent of the lesser weight, is equal to the descent of the larger weight.

This had neither been previously proved, nor is independently known, nor follows logically, nor is universally true. In many cases it is, in fact, false (as will appear from what follows).

From this supposition, at any rate, he concludes that the proportion of the larger weight to the weight that was added is less than that of the descent of the larger weight to the descent of the additional weight (the opposite of what he states to have been given). Yet this is not the case, for it was not previously laid down as universally true that, "of any given unequal weights, the proportion of the larger to the smaller is in the proportion of their descents, taken in the same order," but only specifically that, "of these two weights, which have been chosen, and of their descents." This is, therefore, not incompatible with the thesis that, in the case of certain other weights, the proportion of the larger to the smaller should be less than that of their respective descents; for, in the case of some weights, the proportion of the larger to the smaller is equal to that of their respective descents, in other cases it is greater, and in yet other cases less (as will be clearly demonstrated, later on).²⁶

As for the argument which is most convincing to writers on this subject, it should be replied that, although the causal principle adduced in its proof is true, the first consequence drawn from it does not hold. Fundamentally, a power which is double another power can move a mobile which is twice that moved by the lesser power through an equal distance and time. Instead of it following from this that the greater power can move the lesser mobile twice as fast, it rather follows that the greater power can move the lesser mobile at a speed which is as much greater as that expressed by the proportion of double the resistance to the former speed, and that it requires twice the power to do it. That speed will, in some cases, be exactly double the former speed, in some cases more than double, and in some cases less than double (as will be clear from later portions of this work).²⁷

CHAPTER 2, PART 4

[THEORY IV]

A fourth theory declares there to be neither any proportion nor any relation of excess between motive and resistive powers. It holds that, instead of there being some proportion or excess of motive power over thing moved, motions take place in accordance with some sort of "natural dominance" of mover over moved.

This contention may be seen as founded on the authority of Averroes, who (Comment 79, Physics VIII) in solution of the same problem, says that an incorporeal power is neither to be called finite nor infinite, because only bodies may be referred to in this way. Furthermore, one incorporeal power may not be referred to as greater than another, since the terms, greater and less, may be applied only to quantities. Moreover, powers separate from body can neither be proportionals nor possess proportional relations, since a proportion can only be between one magnitude and another.

From the above it is seen to follow that no motive power can be either finite or infinite, greater or lesser, or in any way proportional to the power of the thing it moves, because no motive power is a body, but is rather a form (either extensive within the body or separate from it).

This theory, together with Averroes’ opinion, may be further confirmed by the definition of proportion, for a proportion is a comparison of two things of the same kind (as is evident on the basis of the definition of proportion set forth in Chapter I). It is obvious, however, that active and passive powers are not of the same kind.

Furthermore, if active and passive powers were to bear a proportion to each other, they would then be comparables. They would, therefore, be of exactly the same species and would consequently, have a subject or substance of exactly the same species. This consequence is false, because powers are divided into active and passive, as is a genus, by incompatible differences. That it nevertheless follows is shown by Aristotle, at the end of Book VII of the Physics, where he expresses the opinion that all things which are to be compared must be of the same individual species and entirely without difference with respect to the subject or substance of comparison, as well as to that regard or those regards in which the comparison is made.

Further, if there were some proportion of a motive power to the thing it moves, it would have to be one of greater inequality, since it would have to exceed the power of the thing moved. And since everything which exceeds something else is divisible into what exceeds and what is exceeded (as appears from Book IV of the Physics, in the chapter on the "void" [215b.12–18—Ed.]), it follows that any motive power may be so divided. This is false, for all incorporeal motive powers are fundamentally indivisible, and an embodied motive power is smaller in extension than the power of the thing moved.

Nor can it be claimed that Aristotle is here speaking of excesses only in the strictest sense (the sense in which they are found among quantities), for he is actually referring to the excess or rarity of one medium over another. To the same effect, in Book I, Chapter 7 of Aristotle’s Rhetoric, where relative good and relative utility are under discussion, there appears the following: "So let the thing that surpasses be as much as and more than the exceeded thing contained within it." Aristotle’s dictum is, therefore, not true merely of the strict usage of "excellence."

This theory is, however, capable of disproof, for if there were no proportion between powers, for the reason that they are not quantities of the same kind, neither could there be such a proportion between musical pitches, and the entire science of harmonics would collapse, accordingly. For the epogdoös or "tone" is constituted in the proportion of nine to eight, the diatessaron in the proportion of four to three, the diapente in the proportion of three to two, the diapason (composed of diatessaron and diapente) in the proportion of two to one, the diapason and diapente combined in the proportion of three to one, and the double diapason in the proportion of four to one This is sufficiently evident from various passages of the Music [probably the De Musica of Boethius—Ed.].

Furthermore, Averroes, in Comments 36 and 38 on Book VII of the Physics, proves certain theorems concerning the proportion of the speeds of motions by means of geometric theorems, as has already appeared in the third argument against Theory I. In Comment 65 on Book I of the De caelo, he also proves this theorem: It being granted that the infinite can move the finite in a finite time and that a finite agent can, in the same time, move part of this finite resistance, no infinite can move a finite. What he does is take a second finite mover which bears the same relation to the former as the whole finite resistance bears to this part. He then argues permutatively, from Definition xii of Book V of Euclid’s Elements, that the proportion of the larger finite mover to the whole resistance is equal to that of the lesser mover to the part. And from this he concludes that the larger finite mover moves the whole resistance in a time equal to that in which the lesser finite mover moves the part and also equal to that in which an infinite mover moves the whole resistance.²⁸

Further, according to the present theory and

(as a matter of fact) in reality, the power of the mover "dominates" that of the thing moved. In many passages Averroes says that the power of the mover exceeds that of the thing moved, and that the mover is of greater power than the thing moved. If, therefore, it "dominates" and exceeds and is of greater power, this must necessarily take place according to some proportion, whether strictly or generally understood, and both Aristotle and Averroes, in many passages, suppose there to be some proportion of the power of the mover to that of the thing moved.

We thus arrive at this affirmative conclusion: A proportion is found to exist between any motive power and the resistive power of the thing it moves.

The first reasons which were brought forward in favor of the present theory are easily countered by means of the definition of proportion already given in Chapter I, for the proportion found to exist between motive and resistive power is not a strict, but only a general one.

The next argument concerning comparison is overthrown by a similar distinction in the meaning of the word, "comparison." The authority which was cited is to be understood as speaking of strict rather than general comparison. It is to be noted, indeed, that comparison is made: (1) within a genus (as, for example, indicated by the terms: "more virtuous," "wiser," etc.), (2) within the most general genus (for example, "form is more [Crosby omits "more" (magis)—Ed.] substance [after "substance" Crosby has "rather," which is omitted here—Ed.] than matter or a compound of the two"), and (3) also in transcendence of every genus (for example, "substance is more [Crosby omits "more" (magis) and has "rather" after "being"—Ed.] being than accident").

As for the final argument, it is to be replied that it is true that there is, in the general sense, a proportion of excess between motive and resistive power. To the authority cited as saying that everything that exceeds is divided into what exceeds and what is exceeded, it must be replied that, just as "what exceeds" may be taken in two senses, so also "to be divided into what exceeds and what is exceeded" may be taken in two senses (i.e., generally and strictly). Everything that exceeds is, therefore, strictly divisible in this manner.

In the general sense, on the other hand, what exceeds may be divided as follows: In a general sense everything that exceeds may be reduced or lessened until equal to what was exceeded; and thus may be understood the entire latitude whereby it exceeds and likewise the similarity or equality which is contained virtually and potentially in the thing that exceeds.

Or it may be carried out thus: Everything exceeding something else is divided, in the general sense, into excess and what is exceeded, not, of course, in itself, but in comparison to something else outside itself (for example, action, and passion or resistance). In this sense, the powers of mover, moved and resistance can be compared to each other in every way in terms of excess and what exceeds. And if one were to take the example of a motive power equal to a resistive power, that motive power is twice half of the resistive power, not because it can produce twice the motion, but because twice the halved resistance has precisely the power of resistance that the motive power has of moving. Concerning every other proportion of mover to moved this is proportionally true.

This theory, therefore, together with the former ones, is pronounced false.

CHAPTER 3

Now that these fogs of ignorance, these winds of demonstration, have been put to flight, it remains for the light of knowledge and of truth to shine forth. For true knowledge proposes a fifth theory which states that the proportion of the speeds of motions varies in accordance with the proportion of the power of the mover to the power of the thing moved.²⁹

This is what Averroes intends when he says, in Comment 71 on Book IV of the Physics: "It is manifest that, universally, the cause of the diversity and equality of motion is the equality and diversity of the proportion of mover to thing moved. If, therefore, there are two movers and two things moved, and the proportions between these movers and the things which they respectively move are equal, then the two motions are of equal speed. If the proportion is varied, the motion is also varied in that proportion."

Further on in the same comment, he also says: "The difference between motions with respect to slowness and fastness varies in accordance with the proportion between the two powers (namely, motive and resistive)."

In Comment 36, on Book II of the De caelo, he says: "Fastness and slowness do not occur otherwise than in accordance with the proportion of the power of the mover to that of the thing moved. By however much, therefore, the proportion is greater, by so much will the motion be faster; and by however much the proportion is less, by so much will the motion be slower."

In Comment 35, on Book VII of the Physics, from a doubling of the proportion of mover to moved he argues a doubling of the speed of the motion, as follows: "If we divide the mobile in two, it necessarily comes about that the proportion of the power of the mover to the thing moved becomes double the former proportion, and thus the speed will be twice what it was before."

Further on, in the final comment, he remarks that: "These two (that is, the speed of alteration and the quantity of time) vary in accordance with the proportion between that which causes the alteration and that which undergoes it. If, therefore, the proportion is great, the speed will be great and the time short, and conversely."

Concerning this same problem, both Aristotle and Averroes (as is evident in the third argument against Theory I) express, in many passages, the opinion that, from an equality of proportion between mover and moved, there follows equality of speed. Equality of the proportion of movers to mobilia is, therefore, the causal condition which, when fulfilled, posits an equal speed of motions and which, when not fulfilled, makes impossible an equality of speeds. Equality of the proportion of movers to mobilia is, thus, the primary and precise cause of equality of the speeds of motions, and to the variation of this cause there directly corresponds the variation of proportion between different motions.

Furthermore, there does not seem to be any theory whereby the proportion of the speeds of motions may be rationally defended, unless it is one of those already mentioned. Since, however, the first four have been discredited, therefore the fifth must be the true one.

We, therefore, arrive at the following theorem: Theorem I. The proportion of the speeds of motions varies in accordance with the proportion of motive to resistive forces, and conversely. Or, to put it in another way, which means the same thing: The proportion[the words "of the proportion," which immediately follow, are omitted here, since they are not represented in the Latin text Ed.] of motive to resistive powers is equal to the proportion of their respective speeds of motion, and conversely. This is to be understood in the sense of geometric proportionality.³⁰

Theorem II. If the proportion of the power of the mover to that of its mobile is that of two to one, double the motive power will move the same mobile exactly twice as fast. This may be demonstrated by means of an example. Let A be a motive power that is twice B (its resistance), and let C be a motive power that is twice A. Then, (by Theorem I, Chapter I) the proportion of C to B is exactly double that of A to B. Therefore (by the immediately preceding theorem), C will move B exactly twice as fast as A does. This is what was to be proved.³¹

Theorem III. If the proportion of the power of the

mover to that of its mobile is two to one, the same power will move half the mobile with exactly twice the speed. This you may demonstrate by an argument like that used for Theorem II.³²

Theorem IV. If the proportion of the power of the mover to that of its mobile is greater than two to one, when the motive power is doubled the motion will never attain twice the speed. This may be demonstrated by means of Theorem IV, Chapter I; and Theorem I, Chapter III.³³

Theorem V. If the proportion of the power of the mover to that of its mobile is greater [Crosby has "less" although the Latin reads maior—Ed.] than two to one, when the resistance of the mobile is halved the motion will never attain twice the speed.³⁴ This may be demonstrated by means of Theorem III, Chapter I and Theorem I, Chapter III.

Theorem VI. If the proportion of the power of the mover to that of its mobile is less than two to one. when the power moving this mobile is doubled it will increase the speed to more than twice what it was.³⁵ This is likewise easily demonstrable, from Theorem VI, Chapter I and Theorem I, Chapter III.

Theorem VII. If the proportion of the power of the mover to that of its mobile is less than two to one, when the same mover moves half that mobile the speed of the motion will be more than doubled.³⁶ This may be demonstrated clearly, from Theorem V, Chapter I and Theorem I, Chapter III.

Theorem VIII. No motion follows from either a proportion of equality or one of lesser inequality, between mover and moved.³⁷ With the addition of the following axiom, independently known:

Axiom 1. All motions of the same species may be compared to each other with regard to slowness and fastness; this theorem may be proved by means of Theorems VII and VIII of Chapter I and Theorem I, Chapter III.

Theorem IX. Every motion is produced by a proportion of a greater inequality, and from every proportion of greater inequality a motion may arise. The first part of this may be proved by Theorems I and VIII of Chapter III and the axiom just given. The second part is demonstrable from the fact that every excess of mover over moved suffices to produce motion, as will be shown elsewhere.

Theorem X. Given any motion, one twice as fast and one twice as slow can be determined. This may be proved by Theorem I and Theorem IX (Part 2) of Chapter III, with the help of the following axiom, independently known:

Axiom 2. A proportion of greater inequality of mover to moved may be halved or doubled indefinitely.³⁸

Theorem XI. An object may fall in the same medium both faster, slower, and equally with same other object that is lighter than itself.

Let, for example, A represent a heavy mixed body composed of heavy and light and having a certain weight, and let B represent some pure heavy body, as small as you please. Now let a given medium be rarefied to the point at which B bears to it a proportion equal to, or greater than, that of the heaviness to the lightness in A. Then let both bodies be placed in the same medium. The heaviness of A will now be in a lesser proportion to its total intrinsic and extrinsic resistance than B is to its resistance. Therefore, by Theorem I, Chapter III, A moves more slowly than B.³⁹

Conversely, let the medium be condensed to the point at which the proportion of B to it is less than the proportion of the heaviness of A to its entire intrinsic and extrinsic resistance. Then, by Theorem I of this chapter, A moves faster than B.⁴⁰

Thirdly, let the medium be so determined that the proportion of B to it is equal to the proportion of the heaviness of A to its entire intrinsic and extrinsic resistance. Then, by Theorem I of the present chapter, A and B will move at equal speeds.⁴¹

Alternatively, let A be supposed to have a determinate speed, C, in a vacuum, and let some medium be rarefied until B falls in it with speed C or faster; then A. placed in the same medium, will fall more slowly than B.⁴² Conversely, let the medium now be condensed as required, and the remaining two consequences will follow.⁴³

Corollary 1. It is manifest, from the foregoing, that the fastness and the slowness of any pure body and the slowness of any mixed body may be doubled indefinitely, but that the fastness of a mixed body may not be so doubled by rarefaction of the medium.⁴⁴ This corollary is sufficiently well established on the basis of what has been said above.

Theorem XII. All mixed bodies of similar composition will move at equal speeds in a vacuum. In all such cases the moving powers bear the same proportion to their resistances. Therefore, by Theorem I of this chapter, all such bodies move at the same speed.⁴⁵ From this you must also understand that:

Corollary 2: If two heavy mixed bodies of unequal weight, but similar composition, were balanced on a scale within a vacuum, the heavier would descend.

Let A and B represent two such heavy bodies, A greater and B less; let C and D represent the heaviness and lightness of A, respectively, and let E and F represent the heaviness and lightness of B, respectively. Then C, D, E, and F are four proportionals, C being the greatest and F the smallest.⁴⁶ Therefore (by Axiom [Crosby has "Theorem" —Ed.] VIII, Chapter I) C and F, combined, exceed D and E, combined.⁴⁷ Since C and F tend to raise B and only D and E resist, therefore (by Part ii of Theorem IX of the present chapter) B ascends and A descends.⁴⁸

^1. Reprinted with permission of the copyright owners, the Regents of the University of Wisconsin, from Thomas of Bradwardine: His Tractatus de Proportionibus; Its Significance for the Development of Mathematical Physics, edited and translated by H. Lamar Crosby, Jr. (Madison, Wis.: University of Wisconsin Press, 1955). The following selection is from chapters 2 and 3, pp. 87–117. Bradwardine wrote the Tractatus de proportionibus (Treatise on Proportions) in 1328.

^2. The introduction and chapter 1 were devoted to a description of the various types of ratios, proportion-alities, and the enunciation of a number of axioms and theorems necessary for subsequent developments. Many of the preliminaries were drawn from the Arithmetica of Boethius and the Elements of Euclid (especially Bk. V).

^3. This first erroneous theory is representable by

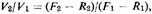
where V is velocity, F motive force, and R the resistance of the moving body (or, should the body fall with a natural downward motion, R would represent the resistance of the medium).It is likely that Bradwardine’s version of this theory was derived ultimately from Avempace, a conjecture that gains support from the fact that a few lines further on, we find mentioned Averroes’ Comment 71 on Book IV of Aristotle’s Physics, the very place where Avempace’s views were reported. If so, this could only have occurred by reading into Avempace’s words a great deal more than was warranted, for it was noted (Selection 44, n. 17) that Avempace did not represent the natural motion of bodies by the formulation

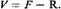
^4. References to the comments of Averroes on the Physics and De caelo (On the Heavens) of Aristotle may be found in the Aristotelis opera cum Averrois commentariis (Venice, 1562–1574; Frankfurt: Minerva, 1962), Vols. IV and V. Comment 71 is translated above, in Selection 44.

^5. 250a.4–9. In this quotation, Bradwardine has omitted an inessential line.

^6. The seventh axiom or assumption declares that if four quantities are related as

then permutatively

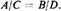
^7. 250a.25–28. This quotation is meant to be identical with one given above even though the Latin texts, and hence the translations, differ somewhat, most noticeably in the replacement of "powers" (potentiae) by "movers" (motores).

^8. The phrase "equally greater or less than" does not appear in the modern Greek text nor in the popular medieval Latin version by Campanus of Novara.

^9. A heavy mixed body was one compounded of both heavy and light elemental bodies, where the light elements, with their natural upward inclination, were assumed to function as internal resistance to the prevailing heavy elements, whose natural downward inclination functioned as the motive force actually moving the body downward. Every such body was held to be capable of moving through a hypothetical vacuum with finite speed—rather than instantaneously, as Aristotle held—since its internal resistance guaranteed that the motion would take time and consequently be theoretically measurable. The innovator of the concept of internal resistance, a radical departure from Aristotle, is unknown to me. Mixed bodies and internal resistance play a fundamental role in Selections 55.2,4,5.

^10. Let

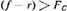
represent the difference between motive force and internal resistance in A, a heavy mixed body that can fall in a resistant medium B and also fall in the void with a determinate speed. Furthermore, let C be a quantity of pure elemental earth whose motive power is represented by F_c(because C is a pure elemental body, it has no internal resistance). Finally, it is assumed that (although the motive power of a body C is always relative to the resistive power of a corporeal medium, in this example Bradwardine seems to compare to the absolute power of C without reference to a medium; indeed, it is as if C were falling in a void).

Since A is taken as falling with a finite velocity in the void, it is possible to produce an equal velocity for C falling in medium B by properly adjusting the density of B. In terms of the first erroneous theory, we may represent this as:

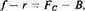
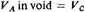
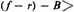
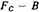
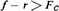
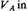
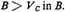
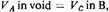
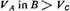
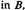
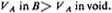
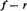
so that in B. But now if A is let fall in the same medium B, it will fall with a speed greater than C, since (for by assumption ) so that Drawing together these two consequences, we see that and from which it follows that By the assumption of a subtractive relation between the motive force and internal resistance of A (that is, ), one derives the absurd consequence that one and the same heavy mixed body wilt fall more quickly in a plenum than in a vacuum.

^11. A lapse in Bradwardine’s thought seems to have occurred here. For the proof just presented (and summarized in the preceding note) demonstrates that the same body would move faster through a medium than through a void, and not that it would move with equal speeds in medium and vacuum (in Physics IV.8.215b.20—216a.7, Aristotle showed that one and the same body would, in the same time, fall equal distances in void and plenum).

^12. It must be understood that Bradwardine is here speaking of heavy mixed bodies of earth possessing internal resistances as well as being opposed by an external resistance in the form of an ambient medium. Let A₂ and A₁ be heavy mixed bodies, with

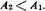
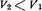
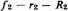
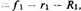
If where f is motive force and r internal resistance, then, as a consequence of the erroneous theory, (where V₂ is the velocity of A₂ and V₁ of A₁). 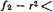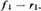Taking R₁ as the initial resistance of the external medium and R₂ as the resistance of the medium after it has been rarefied to the point where it follows that the velocities of A₂ and A₁ are equal—that is, the two forces f₂ and f₁ now move A₂ and A₁ with the same speeds even though That Bradwardine should have taken seriously this criticism is surprising. An adherent of the theory in question would undoubtedly have replied that it is the total resistance—not just internal resistance—which must be subtracted from the motive force, and if the remainders are equal, so also are the resultant velocities.

^13. On the assumption that a medium is infinitely rarefiable (this was assumed above), Bradwardine now argues that if

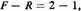
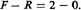
where F is the motive force of a piece of pure earth, say A, and R the resistance of the medium, then A could not double in speed in any whatever corporeal medium, but could do so only in void space, where But if, as all believed, a medium is infinitely rarefiable, it ought to be possible to double any speed by holding the force constant and suitably rarefying the medium. Hence a universally accepted Aristotelian position is violated and the theory is held to collapse. Bradwardine’s argument is wide of the mark, since a partisan of this theory was committed to a rejection of the Aristotelian position that velocity is inversely proportional to the density of the medium.

^14. That is, velocity varies as F/R, where

(rather than as where ).

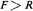
^15. We may represent this as

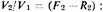
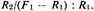
I know of no supporters of this theory prior to Bradwardine, and can cite only Giovanni Marliani (d. 1483) as one who adopted it after Bradwardine. In Latin, the language describing this theory is almost indistinguishable from that of the previous theory. It is only by means of specific examples that one can tell them apart with any assurance.

^16. If

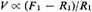
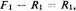
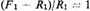
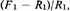
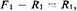
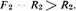
and then and we have a ratio of equality, which, by Theorem VII of chapter 1, can bear no exponential relation to ratios of greater or lesser inequality. In short, a ratio of equality, (1/1)ⁿ, cannot be made equal to p/q, where and p and q are integers. Thus where cannot be made equal to, or greater than, or be compared in any way with, where As a consequence of this theory, then, it follows that whatever the speed with which F₂ moves R₂, it is not comparable to the speed with which F₁ moves R₁. While this conequence is an important feature of Bradwardine’s "true" theory (see later), it seems strange to us that he should invoke Theorem VII of chapter 1 against this "erroneous" theory, where such a theorem would have been wholly irrelevant. However, it was fairly typical of medieval scholastic argumentation to repudiate opposing theories by deriving consequences from the basic presuppositions of one’s own theory, which were then used against opposition theories subscribing to a quite different set of fundamental assumptions. (Nicole Oresme does much the same thing; see n. 59 below.)

^17. I have substituted the remainder of this sentence for Crosby’s version which reads: "whereby the excess of the moving power over its mobile is related to the power of that mobile." While this presents an accurate verbal description of the theory Bradwardine repudiates, it adds elements that do not appear in the Latin text, which differs very little from the first rejected theory.

^18.

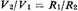
when

^19.

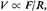
when The two parts of this third theory are representable as which actually represents Aristotle’s verbal formulation despite Bradwardine’s attempt to associate Aristotle with his own theory.

^20. This is from the Liber Jordani de ponderibus (The Book of Jordanus on Weights), the third treatise mentioned in section 2 of the introduction to Selection 39. Despite the title, it is not by Jordanus of Nemore.

^21. If

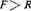
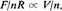
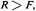
where and produces motion, then where Thus, when and however great R becomes, F will nevertheless be capable of producing a positive velocity in accordance with the ratio it bears to nR. Hence F will be of "infinite capacity," able to move any resisting weight, however large (Oresme draws the same consequence and then demonstrates that, although it should follow from the false theory, it actually does not; see Selection 51.2). The theory is rejected by Bradwardine as physically impossible, since it violated the generally accepted axiom that in order for motion to occur a motive power must exceed the resistance opposing it, whether this resistance be another body or a corporeal medium. Bradwardine’s new theory remedied this defect (see n. 30). Aristotle, who first formulated and accepted this third theory, actually anticipated Bradwardine’s criticism and would have countered by insisting that if after successive halvings of a velocity, R became equal to, or greater than, F, motion would cease and mathematical rules of proportionality would no longer apply (see his shiphauler argument in Physics VII.5.250a.10–20).

^22. Here

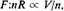
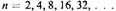
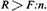
where As the motive force, F, is successively halved, it will happen eventually that But however small and feeble F becomes it can yet produce a proportionate velocity in R. See note 30.

^23. As can be seen further on (Theorem VI, chapter 3), Bradwardine’s theory will mathematically yield such results.

^24. Bradwardine argues here that partisans of the third erroneous theory have misinterpreted the authors cited in their favor. The references and quotations from Aristotle, Averroes, and the Liber de ponderibus do not, Bradwardine insists, uphold the position that when

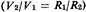
the ratio of velocities will vary reversely as the ratio of resistances but rather that the ratio of velocities will vary as the relation between me whole ratio F₂/R₂ to the whole ratio F₁/R₁. These authors do not take V to vary with R alone when F is held constant; nor indeed do they take it to vary with F alone when R is held constant; but it will vary with alterations in the whole ratio F/R. Bradwardine sees these authors as proponents of a viewpoint that is akin to his own, although, as he declares, none of them have demonstrated it. In truth, none of these authors subscribed to the position attributed to them by Bradwardine.

^25. If

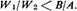
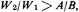
(W is the weight of a body) and where A and B are lines representing the speeds of descent of the weights, and if it is further assumed that it follows that But Bradwardine insists that this does not controvert the initial assumption that since the anonymous commentator has merely converted a relation between ratios of greater inequality to one between ratios of lesser inequality.

^26. If W is the weight of a body and V its velocity, the commentator assumes initially that

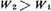
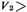
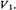
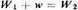
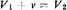
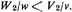
and He then says that (w is the added weight) and assumes, therefore, that (where v is the velocity of w), from which he apparently concluded that Although this conclusion does not seem to follow from the data, Bradwardine accepts it as proper but argues that he sees in it nothing that is incompatible with his own view, since this consequence does not invalidate any general rule to the effect that 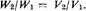In fact, there is no such universally proven rule, so that it cannot be said that this commentator has disproved it. 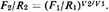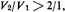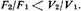Indeed his consequence is compatible with consequences that are derivable from Bradwardine’s own theory, where generally For example, in Theorem VI of chapter 3 (see later), (that is, the ratio of motive forces or weights are related as 2/l) and so that Therefore, just as in the consequence drawn by the commentator, the ratio of forces or weights are not related as the ratio of velocities taken in the same order.

^27. See in Theorems I–VII of chapter 3.

^28. Averroes does little more than repeat the argument given by Aristotle in Book I of De caelo (275a.14–22), where the latter assumes that A is an infinite power, BF (mistakenly called B at the outset) a finite resistive power, and C the time of A’s acting on BF. Now, if A should produce a finite motion in BF during time C, then during the same time a finite power, D, should be able to cause the same motion in F, a finite resistive power or mobile less than BF. Now, because

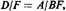
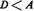
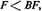
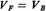
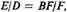
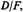
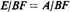
where and it follows that in time C (V is velocity). Next, take another power E such that so that by permutation of ratios from which it follows that and, as Aristotle concludes, "the finite and the infinite effect the same alteration in equal times. But this is impossible; for the assumption is that the greater effects it in a shorter time." The relevance of this argument for Bradwardine is that Averroes (and, of course, Aristotle) has accepted as proper the relation between the finite movers and their respective resistances and would obviously oppose this fourth theory.

^29. This fifth and "true" theory is Bradwardine’s function, which we have already represented as

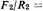
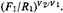
In general, to obtain n times any velocity arising from a ratio F/R, the latter must be raised to the nth power. So vague is the language used here and in Theorem I to describe this theory that without reliance on the subsequent theorems it would be almost impossible to discern Bradwardine’s meaning. In what immediately follows, Bradwardine associates Averroes, and to a lesser extent Aristotle, with his own theory, but there is little doubt that their views are quite properly represented by Bradwardine’s third erroneous theory.

The exponential function described here, and commonly called "Bradwardine’s Function," probably had its origin in a pharmaceutical rule which sought to calculate the effects of hot and cold parts in compound medicines. On the formulation of this rule by

and Arnald of Villanova, see Michael R. McVaugh, "Arnald of Villanova and Bradwardine’s Law," Isis LVIII (1967), 56–64. For the suggestion that Bradwardine might have derived his function directly from Walter of Odington’s Icocedron, in which the rule is applied to the quantitative composition of intensive qualities, see Donald Skabelund and Phillip Thomas, "Walter of Odington’s Mathematical Treatment of the Primary Qualities," Isis LX (1969), 331–350.

^30. This is Bradwardine’s function, already cited in the preceding note. No formal proof of it is offered, but its truth is assumed on grounds that only five possibilities exist, and since four have already been "proven" false, Bradwardine’s theory stands confirmed by a process of elimination. We can now see how Brad-wardine avoided the major criticism which he directed against the third erroneous theory (see n. 21 and n. 22). If initially motion occurs, then necessarily

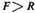
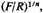
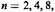
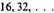
and it is thereafter impossible for R to become equal to, or greater than, F. This is obvious, since in his function, successively halving a velocity arising from a given ratio F/R is achieved by taking where In this way Bradwardine remained faithful to contemporary Aristotelian physics by insisting that velocity is determined by a ratio of force to resistance, but avoided the mathematical difficulties.

^31. Once again using F’s, R’s, and V’s instead of A,B, and C, we see that if

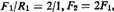
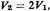
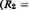
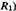
and then so that since 2/1, the exponent, represents the ratio of velocities Therefore, F₂ moves the same resistance with twice the velocity with which it is moved by F₁.

^32. If

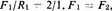
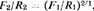
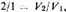
and then where and it is shown that the same power moves half the original resistance with twice the speed of the whole resistance.

^33. If

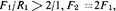
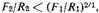
and it follows that so that

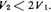
^34. If

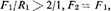
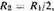
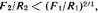
and it follows that so that

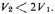
^35. If

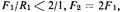
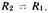
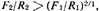
and it follows that so that

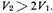
^36. If

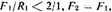
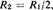
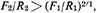
and it follows that so that

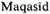
^37. When

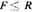
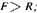
no motion can occur. In medieval physics this was accepted as almost axiomatic, since it was held that motion could occur only when the motive force exceeded the resisting body or medium (that is, when this is made explicit in the next theorem). 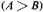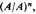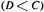The proof of Theorem VIII is based on Theorems VII and VIII of Chapter 1, where it is shown that a ratio of equality, A/A, is not relatable by any exponent whatever to a ratio of greater inequality A/B in the sense that where n may have any value however great, always remains equal to A/A and consequently cannot be made equal to, or greater than, A/B; similarly, a ratio of lesser inequality D/C is not relatable by means of any exponent to a ratio of greater inequality A/B, since whatever the value of n in the expression 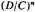it will always remain less than A/B.

Although Bradwardine might have appealed to the accepted axiom that no motion could be produced when

he chose instead to "demonstrate" this mathematically by showing that in the context of his function even if velocities could arise when such motions were not comparable to motions arising when

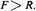
^38. When

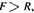
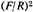
he motion produced can always be doubled or halved by squaring or extracting the square root of F/R—that is, determining or

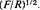
^39. Let f/r represent the ratio of heavy to light (or motive force to internal resistance) in body A, and let B represent a pure heavy body as small as you please; and assume also that R is an external medium which is rarefied so that the ratio of

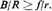
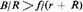
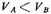
Upon placing both bodies in R, we see that and therefore in R.

^40. Here

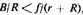
so that

^41. Here the density of the medium is such that

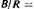
and

^42. In heavy mixed body A let f/r produce speed C in a vacuum and assume the density of medium R to be such that pure elemental body B is related to it as

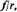
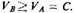
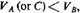
so that Subsequently dropping A in R makes since

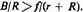
^43. By properly adjusting the density of R one can arrange to have A and B fall in R so that

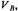
and

^44. The speeds of a pure elemental body and a heavy mixed body can be halved indefinitely because theoretically a corporeal medium can be made infinitely dense. But only a pure elemental body can have its velocity doubled indefinitely, since it was almost universally assumed that a corporeal medium is rarefiable ad infinitum. But rarefaction of a medium ad infinitum will not permit the infinite doubling in speed of a mixed body, for when R becomes zero or void in the formulation

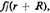
a maximum finite speed would be determined by the ratio of motive force to internal resistance, namely f/r.

^45. Since the two unequal mixed bodies are of homogeneous composition, the ratio of motive force to internal resistance (f/r) per unit of matter is equal in each body and consequently their velocities of fall in the void will be equal. Bradwardine relies here on a specific factor (namely equality of ratio per unit of matter) rather than utilizing an extensive factor, or gross weight, as did Aristotle. Indeed the distinction is analogous to that between specific and gross weight. This important conclusion, which was rejected by Aristotle as an absurdity (Physics IV. 8.216a. 14–21), is akin to that enunciated in the sixteenth century by Galileo in his De motu (On Motion) (and even earlier by Giovanni Benedetti), where it is declared that homogeneous bodies of different weight or size would fall in the void with equal finite velocities. Galileo, however, abandoned the medieval distinction between pure elemental bodies (for Brad-wardine such bodies, devoid of internal resistance, could not fall in the void with finite velocities) and mixed bodies, and the dichotomy between absolute heaviness and lightness, so that his arguments in favor of essentially the same conclusion were quite different, despite a few striking similarities (see Selection 55.5 and n. 79 thereto).

^46.C, D, E, and F are four proportionals, because A and B are homogeneous bodies.

^47. Axiom VIII, Chapter 1, states that if "four quantities are proportionals and the first the largest and the last the smallest, the sum of the first and last wilt be necessarily found greater than the sum of the other two" (trans. Crosby).

^48. Upon suspending heavy mixed bodies A and B (with

) from a balance placed in a vacuum, we see

from the figure (added here for convenience; it is not in the text) that C and F (that is, the downward acting motive power of A and the upward acting resistive power or lightness of B) act conjointly to move B upward, while D and E (the upward acting resistive power or lightness of A and the downward acting motive power or B) act conjointly to move A downward. Since we have a constrained system and

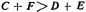
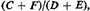
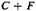
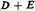
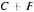
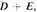
(by Axiom VIII, Chapter 1, quoted in n. 47), we can form a ratio of greater inequality where acts as total motive power and as total resistance. Now, by the second part of Theorem IX, Chapter 3 "from every proportion of greater inequality a motion may arise," so that motive power will cause A to descend, while the total resistance, will cause B to ascend.