|
|
A Source Book in Astronomy, 1900–1950
Contents:
Bart Jan Bok The Distribution of the Stars in Space University of Chicago Press Chicago 1937 40–43
Wolf’s Method of Measuring Dark Nebulae
By Bart J. Bok
In 1923 Wolf illustrated, with a striking diagram, a paper on the distribution of the stars for a field in the region of obscuration near the Veil Nebula in Cygnus. Similar diagrams have been published in several of Wolf’s more recent papers. The advantages of this form of presentation are so obvious that it is not surprising that most of the papers relating to dark nebulae, published during the last ten years, are illustrated with "Wolf diagrams" for the regions under investigation. Figure 1 shows a Wolf diagram for the region near the North America Nebula. The abscissae are apparent photographic magnitudes; and the ordinates are the values of log A(m), A(m)dm being the number of stars per square degree having apparent magnitudes between m and
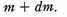
The full-drawn curve gives the counts for the unobscured comparison field, and the dotted curve the data for the region of the dark nebula. Wolf concludes from this curve that the counts for the obscured region indicate the presence of two dark nebulae, one at a distance corresponding to that of the average star of the eighth or ninth magnitude, the other at a distance of an average star of the twelfth magnitude. Using the secular parallaxes of Kapteyn and van Rhijn, Wolf places the first nebula at a distance of between 100 and 200 parsecs, the second at a distance of 600–700 parsecs. The absorbing power of the first nebula would amount to 0.5 mag., while that of the second nebula is estimated to be as high as 3.5 mag.
The very extensive use which has been made in recent years of Wolf diagrams, similar to Figure 1, renders it necessary to examine critically the conclusions that may be drawn from a mere inspection of the curves in such a diagram. Unfortunately, it appears that the results obtained in this fashion are frequently open to criticism. The first source of error may be in drawing the continuous curve through the points in the diagram. All too frequently investigators have disregarded the fundamental fact that the natural uncertainty equals the square root of the average counted number. Before attempting to draw the smooth curve, the investigator should know exactly the amount of the fluctuations in the value of log A(m) to be expected because of purely random fluctuations. As an illustration of the procedure we shall consider a dark nebula with a total area of 0.5 square degree in which 50 stars have been counted between magnitude limits 13.5 and 14.5. The natural uncertainty in this counted number is equal to ±7. Reduced to an area of one square degree, the counted number becomes equal to 100, with a natural uncertainty of ±14. In our particular case the value of log
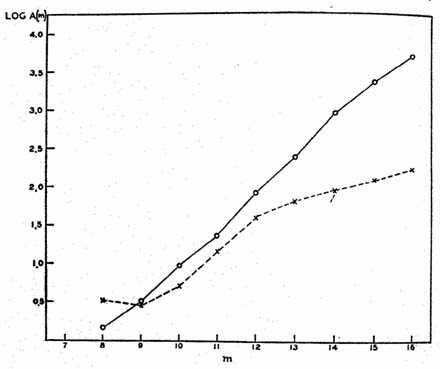 Fig. 1. A Wolf diagram for the region of the North American Nebula. Fig. 1. A Wolf diagram for the region of the North American Nebula.
A′ (14) should be taken equal to 2.00±0.06; the influence of random fluctuations may be best represented by drawing a vertical arrow of appropriate length. The only justifiable procedure for obtaining the Wolf curves is to draw through the observed points the smoothest curves which represent the observed values of log A(m) within the limits of accuracy set by the random fluctuations. The difference between the observed and the smoothed values of log A(m) should exceed the natural uncertainty in one out of three cases, because of random fluctuations. In the drawing of the smooth curves the influence of the purely accidental errors in the magnitudes and in the counts should, of course, be considered.
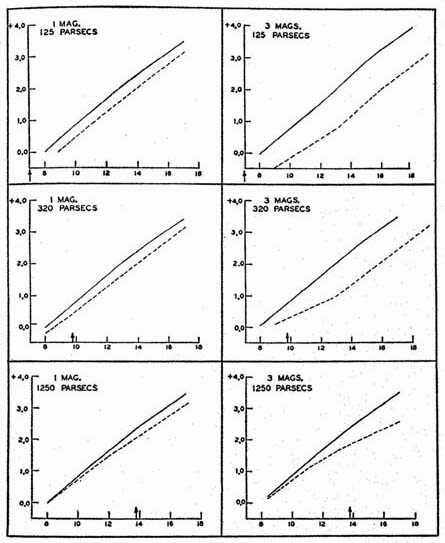 Fig. 2. Imaginary Wolf diagrams, constructed by Freeman D. Miller. Fig. 2. Imaginary Wolf diagrams, constructed by Freeman D. Miller.
There has been a regrettable tendency among the users of Wolf diagrams to assert that every single discontinuity in the curve for the obscured region is indicative of the presence of another dark nebula. In hardly any case is it justifiable to postulate, on the basis of general star counts alone, the existence of more than a single dark nebula in a field. The influence of the large spread in the general luminosity function has not been sufficiently appreciated by most workers in this field.
Pannekoek’s theoretical curves for the field in Taurus show how gradually, for an infinitely thin nebula, the divergence between the curves for the free and for the obscured regions grows with the apparent magnitude. The point is further illustrated in Figure 2, where a series of imaginary Wolf diagrams, constructed by Freeman D. Miller for use in his unpublished dissertation, is reproduced. The upper curve in each diagram represents the value of log A(m) computed for the average unobscured region in the zone of galactic latitude 0°–20°, from Seares’s counts. Using van Rhijn’s general luminosity function, the density distribution for the average field was found by a method of trial and error. With these densities Miller computed the log A(m) curves which would have been observed if a dark nebula absorbing either one or three magnitudes were interposed at distances of 125, 320, or 1250 parsecs. I doubt very much whether anyone who might be presented with a series of counts similar to those of the last diagram of Figure 2 would have suspected, without further analysis, that the difference between the free and the obscured regions could be explained by the presence of a single dark nebula absorbing 3.0 mag. at a distance of 1250 parsecs! It is significant that some observed Wolf curves bear a marked resemblance to Miller’s theoretical curves; the Wolf diagram for the obscuration near the North America Nebula (Fig. 1) is similar to the last diagram of Figure 2. A single dark nebula at a distance of the order of 1000 parsecs, with an absorption of at least 2.5 mag., will explain all the significant features of the Wolf diagram in Figure 1. The large spread in the general luminosity function is responsible for the fact that the influence of this particular dark nebula may be already traced among the ninth magnitude stars.
[For Wolf’s method also see "Ueber den dunkeln Nebel N.G.C. 6960" by M. Wolf, Astronomische Nachrichten, 219, 109–116 (1923).]
Contents:
Chicago:
Bart J. Bok, "Wolf’s Method of Measuring Dark Nebulae," A Source Book in Astronomy, 1900–1950 in A Source Book in Astronomy, 1900–1950, ed. Harlow Shapley (Cambridge: Harvard University Press, 1960), 285–288. Original Sources, accessed September 18, 2025, http://www.originalsources.com/Document.aspx?DocID=D3FHGA8SXHD2Z6M.
MLA:
Bok, Bart J. "Wolf’s Method of Measuring Dark Nebulae." A Source Book in Astronomy, 1900–1950, in A Source Book in Astronomy, 1900–1950, edited by Harlow Shapley, Cambridge, Harvard University Press, 1960, pp. 285–288. Original Sources. 18 Sep. 2025. http://www.originalsources.com/Document.aspx?DocID=D3FHGA8SXHD2Z6M.
Harvard:
Bok, BJ, 'Wolf’s Method of Measuring Dark Nebulae' in A Source Book in Astronomy, 1900–1950. cited in 1960, A Source Book in Astronomy, 1900–1950, ed. , Harvard University Press, Cambridge, pp.285–288. Original Sources, retrieved 18 September 2025, from http://www.originalsources.com/Document.aspx?DocID=D3FHGA8SXHD2Z6M.
|