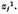Mathematics

Lipschitz on the Lipschitz Condition

¹

The most important advances in the theory of systems of differential equations since the work of Jacobi have stemmed from the development of complex analysis. For each system of differential equations, the first essential question is . . . whether one can determine a system of functions of the independent variable which satisfy the equations and which assume prescribed values for a given value of that variable. This question has been studied when the system of differential equations permits one to regard the variable and [its values] as [complex] quantities . . . . Since every function of a complex variable can be expanded in a power series [in

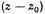
], except at certain [singular] points of its domain, the question posed evidently depends on this: can one find series, convergent in some domain of the independent variable, which, when substituted for the unknown functions, satisfy the differential equations? . . .

If, on the contrary, the expressions which enter into the system of differential equations are . . . real and do not permit an immediate extension to complex values, one may no longer assume that the unknown functions can be expanded in [such power] series . . . . One must use different methods to establish conditions for complete integrability. No work having precisely this aim has appeared to my knowledge; it is the object of the investigations which follow.²

Suppose that the given system of differential equations, where x is the independent variable and

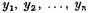
arc unknown functions, can be put into the following form:

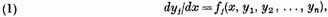
where j takes on the values

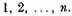
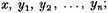
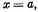
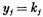
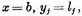
The functions f_j are [assumed] given on a continuously connected set of values of the variables this set of values will be called the domain ["champ"] G. For all values . . . in this domain, the n functions f_j are assumed to be single-valued, continuous, and uniformly bounded. Besides, they must be such that, given two points ["systèmes de valeurs"] and where x remains the same, the inequality³

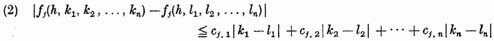
is satisfied: the quantities

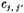
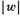
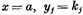
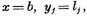
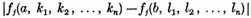
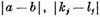
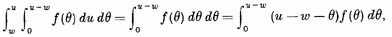
are positive constants, and, here and below, the symbol represents the absolute value of w. The continuity condition imposed requires that, for two systems of values and the difference can be made arbitrarily small when the difference tends to zero: hence if one takes into account the in- equality (2), one sees that this condition [implies] that one can take the difference so small that the inequality

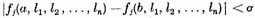
is satisfied, for any preassigned

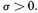
The system (1) will be completely integrated if one determines a system of functions

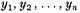
satisfying equations (1), and which for satisfy the equations⁴

Let the point

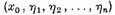
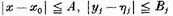
lie in the interior of the domain G, at a finite distance from its boundary. Then one can determine positive quantities A, B_j, such that all points satisfying the inequalities lie in the interior of G; hence, there exist positive finite constants C_j such that, for these same values, one always has

If one chooses the positive quantity M so that

the domain determined by the inequalities

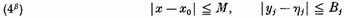
will be entirely in the interior of G; we shall call it H.

Under these conditions, there always exists a unique system of n functions

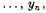
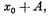
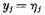
satisfying the differential equations (1), varying continuously in the interior of the domain H₀ as x ranges from to and satisfying the equations at

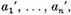
To prove this theorem, it suffices to consider x in the interval

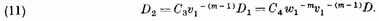
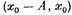
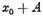
since the interval can be treated similarly. Hence let us imagine a sequence of intermediate values between x₀ and such that

and let us determine n quantities

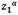
by the following n equations:

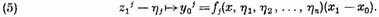
These equations would coincide with the given system of differential equations (1) if one there replaced dx and dy_j in the left side by

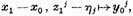
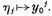
and x, y_j in the right side by x₀, By virtue of the inequalities (4) and (4^α), equations (5) imply the inequalities

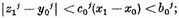
hence the point

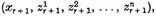
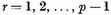
lies in the domain H. One can form similarly a sequence of points setting successively in the equation

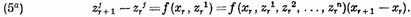
All these points will certainly be in the domain H. We subdivide the interval further by intercalculating between x_r and

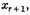
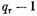
[any] increasing numbers

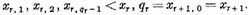
From this new partition of the interval

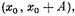
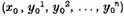
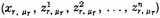
one obtains a sequence of points, beginning with all of which will be in the domain H₀: this new sequence of points is obtained if in the equation

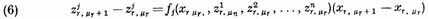
r is replaced by

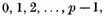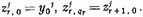
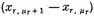
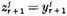
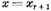
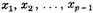
μ_r by the numbers and one finally takes If we regard the first quantities as fixed, and let the integers q increase and the intervals decrease indefinitely according to some law, the problem now is to establish that the values which correspond to . . . converge to a fixed limit . . . . This proof shows that, under the hypotheses stated above, it is always possible to choose so that for arbitrary r and σ,

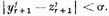
If in equation (6) one successively sets

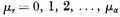
and adds, one obtains the equation

which, by (4), gives

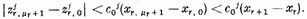
This inequality expresses the fact that if r is held fixed and the subscript μ_r is allowed to range from 0 to

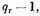
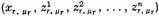
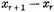
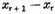
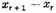
the point remains in a domain K₀ whose [diameter] can be made arbitrarily small by taking the differences sufficiently small. Since, by hypothesis, the function f_j is continuous in the domain D, the difference can always be taken small enough so that the values of f_j are less than any fixed positive constant ε, no matter how small. Supposing that the difference has been determined in this way, the number p is very large, and ε is some proper fraction, equation (7) will give for

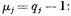
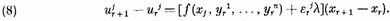
Subtracting equation (5^j) from this equation, it becomes

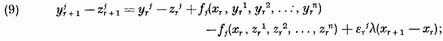
but, because of (2), one has

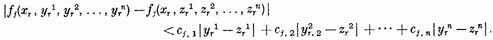
Hence, if one sets

equations (9) give the sequence of inequalities

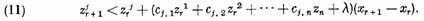
One also has

Now it is clear that, if one forms a sequence of quantities

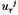
by means of the equations

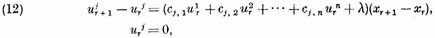
where the subscript r ranges from 0 to

one will always have

Now for our proof we must show that if we take λ sufficiently small, the quantities

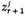
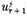
stay arbitrarily small: our goal will be attained if we prove the same thing for the quantities or for larger quantities.

Let c be a positive quantity greater than the largest of the n² constants

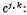
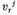
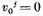
if one defines the quantities by the equations and

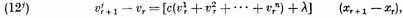
one will evidently have, for

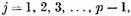
Now the first equation (12^j) gives

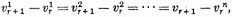
and, by virtue of the second, one has

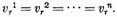
Hence equation (3) can be written

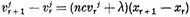
or

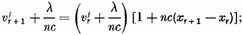
and so one has

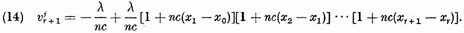
Now the product

where nc is positive, itself has a positive value smaller than

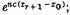
hence one has

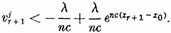
Comparison of this inequality with the inequalities (13) and (13^j) gives

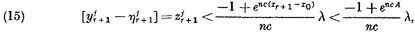
and, since the factor

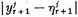
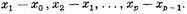
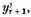
is bounded, one concludes from this that the difference can be made arbitrarily small; for λ is an arbitrarily small quantity depending only on the choice of the intervals As the differences can be taken arbitrarily small, the quantities which correspond to the fixed value of the variable, converge to a determined limit, independent of the law of increase of the number q_r and of the law of decrease of the new intervals. 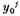By equations (8), these limiting values define a system of solutions of the differential equations, a system for which the functions y^j equal when The existence of a system of solutions satisfying the given initial conditions is therefore established, and the first part of our program has been completed.

[Lipschitz concludes his paper with a rather sketchy and confused proof of uniqueness: "there exists no other solution of the system (1) satisfying the stated conditions."]

¹ Lipschitz, "Sur la possibilité d’intégrer complètement un système . . . ," Bull. Sci. Math. 10 (1876), 149–159.

² The author evidently does not know the works of Cauchy, which have been summarized in an incomplete manner by M. the Abbé Moigno, in his Traité de calcul intégral, and those of Coriolis, in the Journal de Liouville. [Note by the editor of the Bulletin des Sciences Mathématiques.]

³ The inequality (2) is a form of the now classic "Lipschitz condition."

⁴ Lipschitz used superscripts where we have used subscripts,

where we have used η_i, and where we have used