PHYSICS

Experiments in Acoustics

Theon of Smyrna II. 12–13 (pp. 56–61, Hiller)²

12. Pythagoras is reputed to have discovered the numerical ratio of sounds that are consonant with one another, namely, the fourth, in the ratio of 4:3, the fifth, in the ratio of 3:2, the octave, in the ratio of 2:1, the octave plus fourth, in the ratio of 8:3 (which is multisuperparticular, for it is

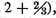
the octave plus fifth, in the ratio of 3:1, the double octave, having the ratio of 4:1; and of the other intervals, those that encompass a tone, in the ratio of 9:8, and those that encompass what is now called a semitone, formerly a diesis, in the ratio of 256 to 243.³

He investigated these ratios on the basis of the length and thickness of strings, and also on the basis of the tension obtained by turning the pegs or by the more familiar method of suspending weights from the strings.

And in the case of wind instruments the basis was the diameter of the bore, or the greater or lesser intensity of the breath. Also the bulk and weights of disks¹ and vessels were examined. Now whichever of these criteria is chosen in connection with any one of the aforesaid ratios, other conditions being equal, the consonance which corresponds to the ratio selected will be produced.²

For the present let it suffice for us to illustrate by means of the lengths of strings, using the so-called monochord.³ For if the single string in the monochord is divided into four equal parts, the sound produced by the whole length of the string forms with the sound produced by three quarters of the string (the ratio being 4:3) the consonance of a fourth. Again, the sound produced by the whole string forms with that produced by half the string (the ratio being 2:1) the consonance of an octave. And the sound produced by the whole string forms with that produced by one quarter of the string (the ratio being 4:1) the consonance of a double octave.

Again, the sound produced by three quarters of the string forms with that produced by half the string (the ratio being 3:2) the consonance of a fifth. The sound produced by three quarters of the string forms with that produced by one quarter (the ratio being 3:1) the consonance of octave plus fifth. If the string is divided into 9 equal parts, the sound produced by the whole string and that produced by 8 parts (the ratio being 9:8) encompass the interval of one tone.

All the consonances are contained in the tetractys consisting of 1, 2, 3, and 4. For in these numbers are the consonances of the fourth, the fifth, the octave the octave plus fifth, and the double octave>, that is, the ratios of 4:3, 3:2, 2:1, 3:1, and 4:1.

Some sought to obtain these consonances from weights, others from magnitudes, others from motions [and numbers corresponding thereto],¹ still others from vessels [and magnitudes corresponding thereto].¹ They say that Lasus of Hermione and the Pythagorean Hippasus of Metapontum investigated the motions with regard to speed and slowness, through which the consonances. . . .²

Believing <that the consonances depended> on numbers, he obtained these ratios in experiments on vessels. For taking vessels that were in all respects identical he left one empty and half filled another with liquid. On striking each he found that the consonance of an octave was produced.³ Again, leaving one of the vessels empty, and filling the other to the one-quarter mark, he struck the vessels and found that the consonance of a fourth was produced. The consonance of a fifth was produced when he filled one vessel to the one-third mark.⁴ The ratios of the empty spaces in the respective vessels was 2:1 in the case of the octave, 3:2 in the case of the fifth, and 4:3 in the case of the fourth.

A similar investigation is made by the division of the strings, as we have said, not, however, with one string as in the case of the monochord, but with two. Thus, tuning two strings to the same pitch, he stopped one of them by pressing it down at its midpoint and the half string produced with the other [full] string the consonance of an octave. When he stopped off a third part of the string, the remaining two-thirds gave with the other [full] string the consonance of a fifth. Similarly in the case of a fourth, when a fourth part was stopped off on one of the strings, the other three parts produced with the other [full] string that consonance.

He performed the same experiment on the pan-pipes with the same result.

Now some produced the consonances by the method of weights, suspending from two strings weights in the aforesaid ratios.¹ Others used the method of lengths, <stopping off different parts> of strings and determining the consonances that are about to be found in strings.

13. . . . that a musical sound

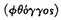
is the incidence of the voice on a single pitch. For they say that the sound must always be homogeneous without the slightest divergence and not composed of different levels of pitch. Now some voices are high and others low in pitch, and the same is therefore true of musical sounds, of which the high pitched is swift and the low pitched slow.

Now if one should blow into two pipes of equal thickness and bore, provided with holes in the manner of a flute, one pipe being double the other in length, the breath in the case of the half sized flute is thrown back with double the speed (of the other). The consonance of an octave results, the lower sound being that of the longer pipe, the higher sound that of the shorter.

The speed or slowness of the motion is the cause of this. And the same consonances are obtained on a single pipe in accordance with the distances between the holes thereof. For if the pipe be divided into two equal parts, the accord of an octave is produced if one blows first into the whole length and then through the hole dividing the pipe in half. Again, if the pipe is divided into three equal parts and two of the parts are on the side near the mouthpiece and one part away from it,² the consonance of a fifth is produced if one blows first into the whole length and then into two-thirds the length. And when there are four divisions, three parts on the side of the mouthpiece and one part away from it, the consonance of a fourth is produced if one blows first into the whole length and then into three-quarters of the length.

Eudoxus and Archytas held that the doctrine of consonances depended on numbers. At the same time they held that the ratios depended on movements and that the swift motion was high pitched³ since it beat upon and pierced the air continuously and more swiftly, whereas the slower motion was low pitched being less active.

So much for the discovery of the consonances. Let us now return to the discussion of Adrastus. He says that when the instruments have been previously constructed according to these ratios for the purpose of obtaining the consonances, the correctness of the ratios is confirmed by the sense of hearing. Conversely, when the sense of hearing is taken as the starting point, the correctness of the perception is confirmed by the [measurement of the] ratio. . . .¹

Boethius, De Institutione Musica I. 10–11 (Friedlein)

10. This,² then, was the chief reason why Pythagoras abandoned the auditory sense as a criterion of judgment and had recourse to the divisions of a measuring rod. He had no faith in a human ear, which suffers change not only naturally but by reason of external accidents and varies with age. He had no confidence in musical instruments because of the great variation and instability to which they were subject. In the case of strings, for example, damper air would weaken the vibrations, drier air strengthen them; a thicker string would produce a lower tone, a thinner string a higher tone, or there might be some other disturbance of the original uniformity. Since the same was true of the other instruments he gave them no consideration, believing as he did that they were worthy of very little confidence. Instead he sought long and ardently for a method by which he might learn the fixed and unalterable measurement of consonances.

Now by a stroke of divine fortune he was passing a metal workers’ shop and heard the hammers when struck produce somehow a single concord from their diverse sounds. Surprised to find that which he had long been seeking he went into the shop and after long consideration concluded that it was the variation in the force of those using the hammers that produced the diversity of sounds. To verify this he had the men exchange hammers. But it turned out that the character of the sounds did not depend on the strength of the men but remained the same even after the hammers were exchanged. On noting this he weighed the hammers. Now there happened to be five hammers, and those two which gave the consonance of an octave (diapason) were found to weigh in the ratio of 2 to 1.³ He took that one which was double the other and found that its weight was four-thirds the weight of a hammer with which it gave the consonance of a fourth (diatessaron). Again he found that this same hammer was three-halves the weight of a hammer with which it gave the consonance of a fifth (diapente). Now the two hammers to which the aforesaid hammers had been shown to bear the ratio of 4 to 3 and 3 to 2, respectively, were found to bear to each other the ratio of 9 to 8. The fifth hammer was rejected, for it made no consonance with the others.

Thus, while it is true that before Pythagoras the musical consonances were called the octave (diapason), fifth (diapente), and fourth (diatessaron), the latter being the smallest consonance, Pythagoras was the first to find, by this method, the proportions involved in these consonances.

To make clearer what has been said, let us suppose, for example, that the weights of the four hammers are represented by the numbers 12, 9, 8, and 6. Then the hammers with weights 12 and 6 gave the consonance of an octave. The hammer of weight 12 gave with that of weight 9 (the ratio being 4 to 3) the consonance of a fourth. The same consonance was given by the hammer of weight 8 with that of weight 6. The hammer of weight 9 gave with that of weight 6 the consonance of a fifth, as did that of weight 12 with that of weight 8. That of weight 9 gave with that of weight 8 (the proportion being 9 to 8) the interval of a tone.

11. On returning home Pythagoras tried to determine by various researches whether the whole theory of consonances could be explained by these proportions. Thus he attached equal weights¹ to strings and judged their consonances by the ear. Again, he varied the procedure by doubling or halving the length of reeds and using the other proportions. In this way he achieved a very considerable degree of certainty.

Often as a means of testing the proportions he would pour cyathi² of fixed weight into vessels, and with a bronze or iron rod strike the vessels containing the various weights. He was overjoyed to find no reason to alter his conclusions. He then proceeded to examine the length and thickness of strings. In this way he discovered the [principle of the] monochord of which we shall speak hereafter. The monochord was called canon³ not merely from the wooden ruler by which we measure the length of strings corresponding to a given tone, but because it forms for this type of investigation so definite and precise a standard that no inquirer can be deceived by dubious evidence.

³ Since the ratio of a tone is 9:8, the ratio of the semitone should be the geometric mean between 1 and 9/8, viz.,

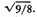
Perhaps to avoid the irrational, the Pythagoreans took the leimma or diesis for certain purposes as a semitone. It is defined as the quotient of the ratio of a fourth by that of a ditone, i.e.,

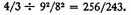
¹ Cf. Scholium on Plato’s Phaedo 108D:

"A certain Hippasus constructed 4 bronze disks in such a way that although their diameters were equal, the thickness of the first disk was four-thirds that the second, three-halves that of the third, and double that of the fourth. And when these disks were struck they produced a concord. Now the story goes that when Glaucus heard the sound from these disks, he was the first to try to play on them. And from his playing on them, the expression ’art of Glaucus’ is used even now."

² These experiments cannot all have been successfully performed. The inverse relation of pitch to string length (and also to string diameter) and to the length of the column of air in wind instruments seems to have been correctly tested. But there is no indication in the ancient sources that pitch varies not with the weight stretching the string, but with the square root thereof. Again, the results of the alleged experiment with "musical glasses" are quite erroneous. That the ratios of pitch really represent ratios of frequency of vibrations seems to be suspected in some of the accounts. Galileo’s demonstration of this marks the beginning of modern acoustical science. It was not until the seventeenth century that the absolute frequency of vibrations corresponding to the various pitches was experimentally investigated.

³ The apparatus consists of a single string, whose effective vibrating length may be varied, and a graduated ruler alongside it.

¹ The material here bracketed is probably not authentic.

² Lasus and Hippasus were members of the early Pythagorean school. Hippasus has sometimes been credited with the discovery of the irrational (see p. 14). There are stories of his expulsion and shipwreck for divulging secrets of the school. It has been suggested that the theory Lasus and Hippasus had referred to was that higher pitched sounds are more quickly propagated than lower pitched (see p. 287).

There seems to be a lacuna in the text of Theon at this point, but the experimenter referred to in what follows is probably Hippasus. For a different view see C. Jan, Musici Scriptores Graeci, p. 131.

³ The results described in this series of supposed experiments are entirely erroneous. Actually the sound obtained by striking the half-filled vessel would be a little lower in pitch. The accord of the octave could be produced by causing the respective columns of air (not the vessels themselves) to vibrate, e.g., by blowing across them, but also in the case of a narrow-necked vessel, by quickly removing a stopper or the finger inserted as a stopper. This seems to be the experimental fact at the basis of the tradition that Theon misinterprets, perhaps because he never repeated the experiment.

⁴ The other vessel remaining empty.

¹ See p. 295, n. 2.

² I.e., separated off by a hole of sufficient size properly placed.

³ On Eudoxus see p. 36, on Archytas p. 35. It is doubtful whether Archytas himself had a clear notion of the vibration of air in connection with pitch (see p. 287). But it is possible that Eudoxus or his followers developed the idea, and, contrary to the older practice, assigned higher numbers to the higher pitched tones.

¹ I.e., two sets of experiments were performed. In the one set the senses confirmed the ratios, in the other set the ratios confirmed the senses. This is of interest in connection with the history of experimental method.

² The preceding discussion had dealt with the unreliability, from certain viewpoints, of the evidence of the senses, e.g., the auditory sense.

³ Boethius does not indicate whether it was the lighter or the heavier hammer that sounded the higher note. But in any ease the theory is without basis. The proportions are valid only when applied to the length of strings (or pipes), other conditions (thickness, material, tension, etc.) being equal. This case is mentioned below.

The story of the hammers had become part of Pythagorean legend long before Boethius. It is told in other Pythagorean sources, e.g., Nicomachus, Manual of Harmonics.

¹ Other things being equal, the frequency of vibration varies not with the weight that stretches the string, but with the square root thereof.

² A measure of volume (12 cyathi equal about 1 pint). The reference is probably to the pouting of proportionate amounts of liquid into various vessels, but it is impossible that the consonances were obtained by striking the vessels (see p. 296, n. 3).

³ Latin regula (Greek

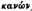
denotes not only the monochord with the straight edge for measurement, but also the standard or model determined by such measurement.