Emil Fischer

The Configuration of Grape Sugar and Its Isomers

All the previous observations in the sugar group are in such close agreement with the theory of the asymmetric carbon atom that we may venture to use it as a basis for the classification of these substances. The theory allows 16 isomers of the structure of grape sugar to be predicted. This number is reduced to 10 for its derivatives whose molecules are symmetric.

The following table, which is taken from page 11 of the booklet of van’t Hoff-Hermann, "Die Lagerung der Atome im Raum," contains the 16 different forms for the sugar, of which numbers 11 to 16 become identical with 5 to 10 in the hexavalent alcohols and the dibasic acids.

In order to choose the form which belongs to grape sugar on the basis of facts, it is first necessary to consider the saccharic acids, Of these, both optically active forms are known; moreover, the d-saccharic acid results on the one hand from grape sugar (d-glucose) and on the other hand from the stereoisomeric d-gulose.

From this it follows that both saccharic acids must come under the numbers 5 to 10; only then can they result from these two stereoisomeric sugars

But under these six numbers are two optically inactive systems (7 and 8) which consequently drop out.

Finally, numbers 6 and 10 can be excluded by the following considerations. Glucose and mannose are distinguished from each other only by the different arrangement on the asymmetric carbon atom which in the following formula is indicated by ^*

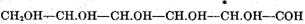
The same applies also to gluconic and mannonic acids, or sorbitol and mannitol, or finally, to saccharic acid and mannosaccharic acid.

I will place together the facts which concordantly lead to this conclusion

1. Mannose and glucose give the same osazone.

2. Arabinose adds hydrocyanic acid to give at the same time l-mannonic and l-gluconic acids.

3. Fructose is converted by sodium amalgam into a mixture of mannitol and sorbitol.

4. Mannonic acid and gluconic acid can be mutually converted into each other by heating them with quinoline.

5. All attempts to separate gluconic and mannonic acids into two components have remained fruitless.

But now, if saccharic acid, or, what is the same thing, sorbitol, possesses the configuration

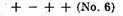
or

then mannosaccharic acid or mannitol must have one of the two configurations

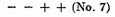
or

But these are the optically inactive systems which again are excluded by the activity of mannitol and mannosaccharic acid.

Consequently there remain for d- and l-saccharic acid only the two configurations

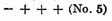
and

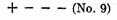
Since it is equally valid to call either one + or -, I will arbitrarily assign to the d-saccharic acid the formula

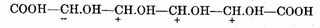
and to the l compound the opposite signs.

Two aldoses correspond to d-saccharic acid,

or

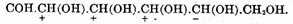
To distinguish which of these formulas belongs to grape sugar and which to d-gulose it is necessary to consider the arabinose and xylose. It is true they belong to the l-series; but this is immaterial for the conclusion.

Arabinose can be changed into l-glucose, while from xylose under the same conditions, l-gulose results.

For l-glucose and l-gulose there once more remains the choice between the formulas

or

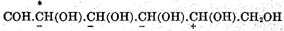
If we remove from both the asymmetric carbon atom marked with the ^* , which is the first result of the synthesis, there remain for arabinose and xylose the following formulas:

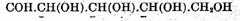
and

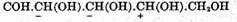
For the pentoses (of the structure of arabinose and xylose) the theory now permits us to predict eight isomers; but the number is reduced to four if the molecule is symmetric. There are thus only four pentavalent alcohols

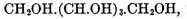
or four different trihydroxyglutaric acids.²

Two of these are optically active and opposites. For the two acids there are the formulas

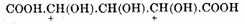
and

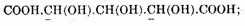
The middle carbon atom here has lost its asymmetry.

The two other formulas,

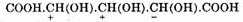
and

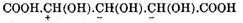
are, on the other hand, identical with their mirror images and must therefore be optically inactive. Possibly such isomers are so similar that they cannot be separated, since the optical test is automatically excluded.

Thus the possibility is offered of distinguishing between the above formulas for arabinose and xylose; since it suffices to test optically the pentavalent alcohols or dibasic acids corresponding to the two sugars.

The attempt has given an unambiguous result.

The arabitol prepared by Kiliani from arabinose, as mentioned earlier, on addition of borax turns polarized light to the left. The same is true for the trihydroxyglutaric acid also obtained by Kiliani from arabinose, as will be shown later.

On the other hand, the xylitol obtained from xylose remains inactive in the presence of borax, and the dibasic acid resulting from the sugar, to be described later, behaves in exactly the same way.

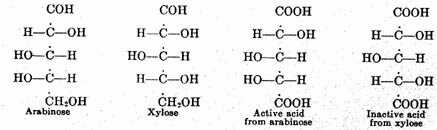
Since the hydroxyacids in general possess a very strong rotation, we are justified in assuming with greater assurance from these results that the derivatives of xylose which we have discussed are in fact optically inactive substances. It follows from this that arabinose has the first of the above two formulas,

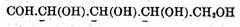
and xylose the second,

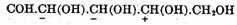
For the compounds of the hexose group, as is easily evident, the following configurations result:

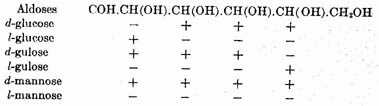
For galactose there still remains the choice between four configurations, as comparison with the formulas of mucic and allomucic acids indicates.

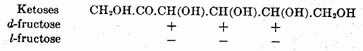
. . . In the first communication, I developed for grape sugar the formula

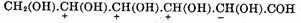
The + and - signs for spatial arrangement which were introduced by van’t Hoff and were retained in unaltered form by me can, however, in such complicated molecules easily result in a mistaken understanding. To prevent this, I consider a detailed interpretation of the formulas to be necessary, and for this purpose I call the four asymmetrical carbon atoms by the numbers 1 to 4:

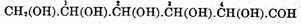
In the general considerations of van’t Hoff, which lie at the basis of my special deductions, carbon atom 1 compares only with 4, and similarly, carbon atom 2 only with 3. In grape sugar, therefore, the arrangement of hydrogen and hydroxyl on carbon atom 1 is the opposite of 4; further, this arrangement on 2 and 3 is the same. But now compare carbon atom 1 also with the two middle atoms. I did this when I related grape sugar to xylose and arabinose. This showed that the arrangement of hydrogen and hydroxyl on carbon atom 1 was the same as on 3.

It would now be possible to believe from superficial considerations that the same must also be true for carbon atoms 1 and 2. Actually, however, exactly the opposite occurs.

With the help of models, it is easy to see that on carbon atom 2 the sign changes, whether it is compared with 1 or 3.

Since, then, the above expression for the configuration of grape sugar is ambiguous, it seemed to me necessary to elucidate it through the following pictures.

Using the very simple Friedländer rubber models, the molecules of d-tartaric acid, l-tartaric acid, and inactive tartaric acids are constructed, and these are laid in such a way on the plane of the paper that the four carbon atoms lie in a straight line, and the hydrogen and hydroxyl which are under discussion stand above the plane of the paper. The following formulas are then obtained by projection:

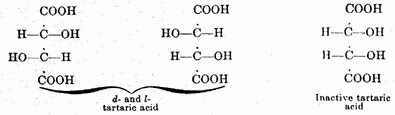
Proceeding in the same way with the models for d-and l-saccharic acids, these two projections result:

Once more I arbitrarily chose the form I for d-saccharic acid, and in it, it naturally remains undecided whether on the carbon atom marked with the ^* the order of the hydroxyl and hydrogen is clockwise or the reverse. Then grape sugar and its isomers give the following forms:

Finally, from the dulcite series, I give the formulas for both inactive dibasic acids which are most probable for mucic and allomucic acids

By the help of projections, the models of these molecules can easily be reconstructed.

Further, these methods of notation, without anything else, can be carried over either to the pentoses or to the heptoses, octoses, etc., in which the ambiguity of the old signs + and - constantly increases.

Thus the following projections are obtained for the two previously known pentoses and the trihydroxyglutaric acids resulting from them, corresponding to the earlier explanations:

The advantages of the new formulas are shown especially by the consideration of some reactions which lead to an increase or a decrease of asymmetric carbon atoms.

An example that will serve is the oxidation of fruit sugar.

According to the observation of Kiliani, on treatment with nitric acid this gives, along with glycolic acid, the inactive tartaric acid. The formation of the latter fits very simply into the above formula:

The results are not sufficiently certain for the changes of other sugars, or the related acids, into tartaric acid or its isomers. Therefore I myself will take up this study.

¹ Ber., 24: 1836–1845, 2683–2687 (1891); the selections given are taken from pages 1836 to 1840 and 2683 to 2386.

² In the van’t Hoff-Herrmann booklet, page 10, this case is discussed only briefly, and the number of isomers is established as three, However, Herr van’t Hoff had the kindness to send me a private communication that a mistake had occurred here, and his theory actually required four isomers, two active and two inactive.